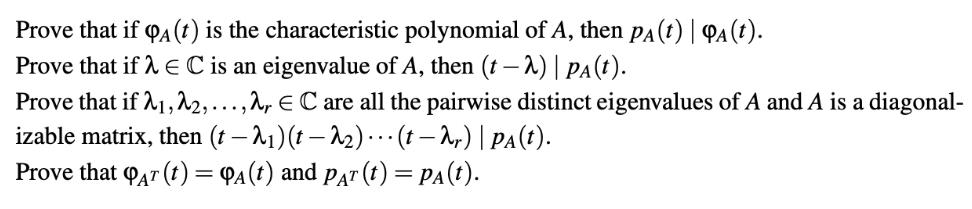
Prove that if QA (t) is the characteristic polynomial of A, then pa(t) | PA(t). Prove that if = C is an eigenvalue of A, then (t-) | PA(t). Prove that if 21, 22, ...,, E C are all the pairwise distinct eigenvalues of A and A is a diagonal- izable matrix, then (t-) (t) (t-r) | PA(t). Prove that Par(t) = QA(t) and Pr (t) = pa(t).