For Exercises 2 through 6, prove that T is a linear transformation, and find bases for both N(T) and R(T). Then compute the nullity
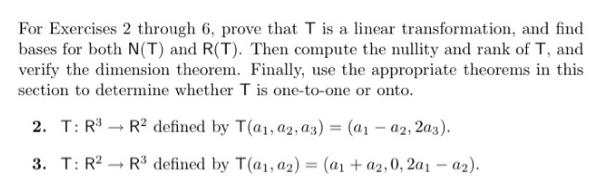
For Exercises 2 through 6, prove that T is a linear transformation, and find bases for both N(T) and R(T). Then compute the nullity and rank of T, and verify the dimension theorem. Finally, use the appropriate theorems in this section to determine whether T is one-to-one or onto. 2. T: R3 - R? defined by T(a1, a2, a3) = (a1 - a2, 2a3). %3! 3. T: R2 - R3 defined by T(a1, a2) = (a1 + a2, 0, 2a1 - a2). %3D
Step by Step Solution
3.51 Rating (154 Votes )
There are 3 Steps involved in it
Step: 1
TR3 R for NIT taiandas O 109 Mullity T dim NIt 1 ...
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Authors: David C. Lay
4th edition
321791541, 978-0321388834, 978-0321791542
