Prove the case involving E of the inductive step of the (strong) soundness theorem for natural deduction in classical propositional logic(you should use the format like below).

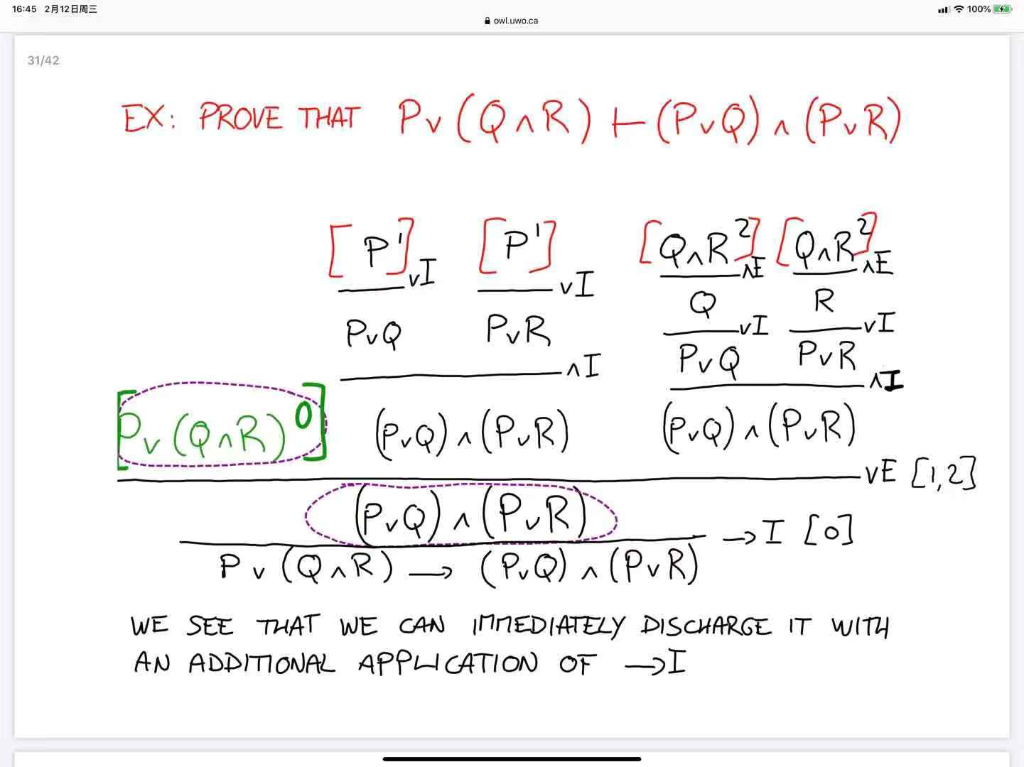
(Strong) soundness INDUCTIVE STEP: suppose that, for any R and A, if RFA with a proof of size n, then REA (IH). We want to prove that if S + B with a proof of size n+1, then SEB. Case 6: the step n+1 is obtained via -I. This means that B = -C for some formula C which was assumed earlier and produced al at the step n of the proof. This means that SU {C} + I with a proof of size at most n. Then, by IH, SU {C} = I. But this means that no interpretation can give the truth value 1 to all formulas in S and to C (because such interpretation would need to give (1) = 1, which is impossible). Then, an interpretation that gives the truth value 1 to all formulas in S must give v(C) = 0, and hence v(-C) = 1. That is, SEB as wanted. (Strong) soundness INDUCTIVE STEP: suppose that, for any R and A, if RFA with a proof of size n, then REA (IH). We want to prove that if SEB with a proof of size n+1, then SEB. Case 7: the step n+1 is obtained via -E. This is your duty to prove (Assignment 2, Ex. 2) 16:45 2 12 owl.uwo.ca 31/42 LOVE THAT EX: PROVE THAT Pv (QAR) +(PvQ) 1 (PvR) [P]. [P) [qur 7 COURSE PuQ PR - Pie PUR Bucano) (Pug). (PUR) Poq)n (PUR) VE [1,2] (PQ)^(PUR) PvQiR) - PuQ) 1 (PvR) -AI I [o] WE SEE THAT WE CAN IMMEDIATELY DISCHARGE IT WITH AN ADDITIONAL APPH CATION OF I (Strong) soundness INDUCTIVE STEP: suppose that, for any R and A, if RFA with a proof of size n, then REA (IH). We want to prove that if S + B with a proof of size n+1, then SEB. Case 6: the step n+1 is obtained via -I. This means that B = -C for some formula C which was assumed earlier and produced al at the step n of the proof. This means that SU {C} + I with a proof of size at most n. Then, by IH, SU {C} = I. But this means that no interpretation can give the truth value 1 to all formulas in S and to C (because such interpretation would need to give (1) = 1, which is impossible). Then, an interpretation that gives the truth value 1 to all formulas in S must give v(C) = 0, and hence v(-C) = 1. That is, SEB as wanted. (Strong) soundness INDUCTIVE STEP: suppose that, for any R and A, if RFA with a proof of size n, then REA (IH). We want to prove that if SEB with a proof of size n+1, then SEB. Case 7: the step n+1 is obtained via -E. This is your duty to prove (Assignment 2, Ex. 2) 16:45 2 12 owl.uwo.ca 31/42 LOVE THAT EX: PROVE THAT Pv (QAR) +(PvQ) 1 (PvR) [P]. [P) [qur 7 COURSE PuQ PR - Pie PUR Bucano) (Pug). (PUR) Poq)n (PUR) VE [1,2] (PQ)^(PUR) PvQiR) - PuQ) 1 (PvR) -AI I [o] WE SEE THAT WE CAN IMMEDIATELY DISCHARGE IT WITH AN ADDITIONAL APPH CATION OF