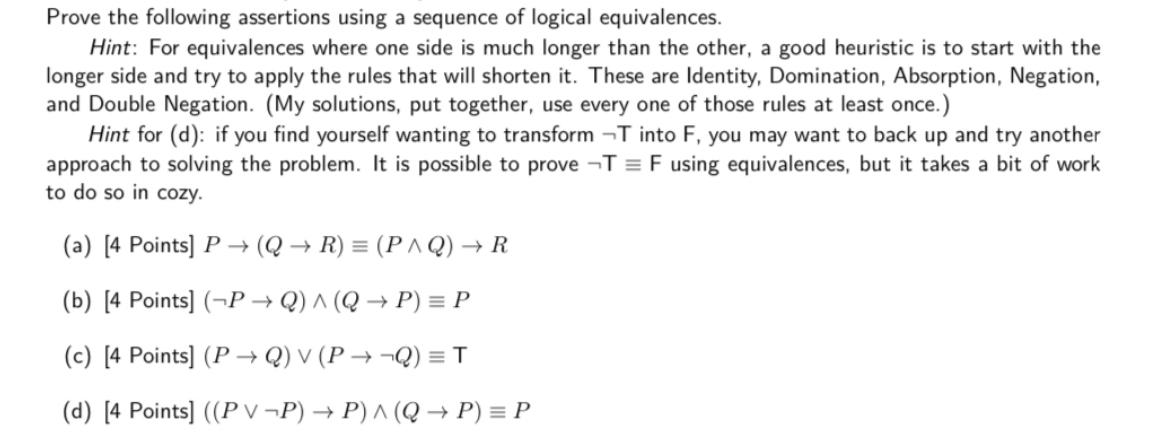
Prove the following assertions using a sequence of logical equivalences. Hint: For equivalences where one side is much longer than the other, a good heuristic is to start with the longer side and try to apply the rules that will shorten it. These are Identity, Domination, Absorption, Negation, and Double Negation. (My solutions, put together, use every one of those rules at least once.) Hint for (d): if you find yourself wanting to transform -T into F, you may want to back up and try another approach to solving the problem. It is possible to prove T = F using equivalences, but it takes a bit of work to do so in cozy. (a) [4 Points] P (QR) = (PAQ) R (b) [4 Points] (PQ) ^ (QP) = P (c) [4 Points] (PQ) V (P Q) = T (d) [4 Points] ((PV-P) P) ^ (Q P) = P