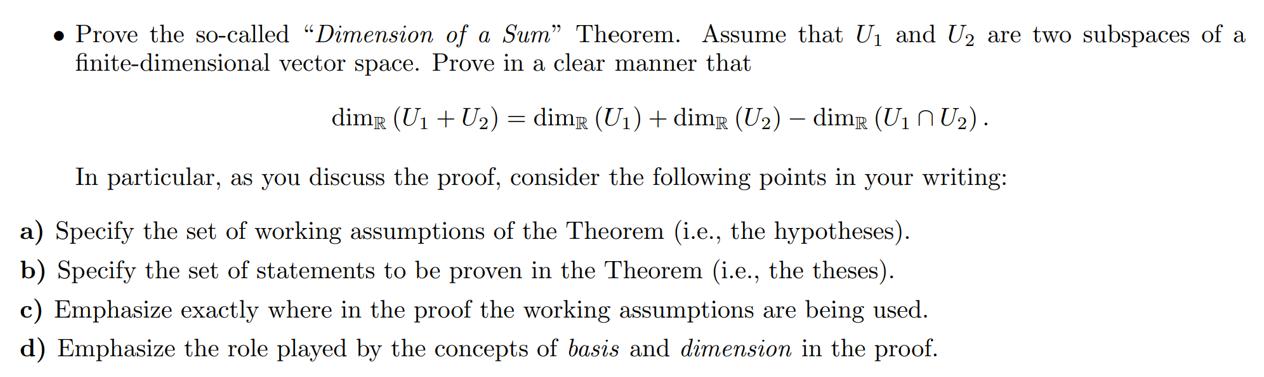
Prove the so-called "Dimension of a Sum" Theorem. Assume that U and U are two subspaces of a finite-dimensional vector space. Prove in a clear manner that dime (U+U) = dimR (U) + dime (U) - dime (UU). In particular, as you discuss the proof, consider the following points in your writing: a) Specify the set of working assumptions of the Theorem (i.e., the hypotheses). b) Specify the set of statements to be proven in the Theorem (i.e., the theses). c) Emphasize exactly where in the proof the working assumptions are being used. d) Emphasize the role played by the concepts of basis and dimension in the proof.