


Provide answers to all questions.,,,
1.
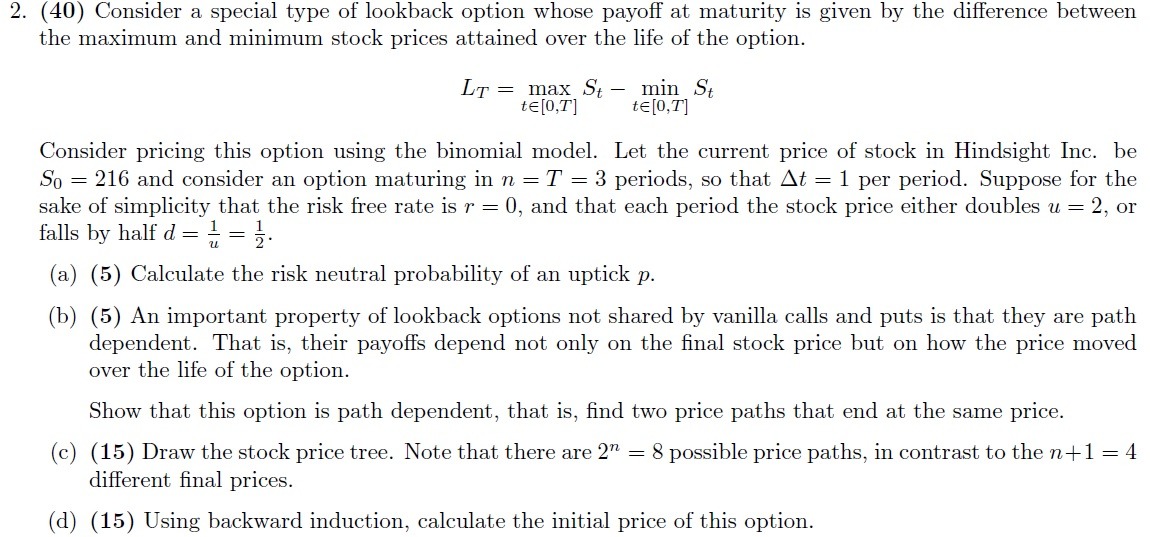
B.1 Two-period Intertemporal Optimization Consider again the 2-period consumption problem of Lecture 2. That is, assume that there are two periods * = 0, 1. Denote the interest rate by r, the discount factor by B, initial financial wealth by fo, income in period 0 by yo, income in period 1 by y1, consumption in period 0 by co, and consumption in period 1 by c1. Instead of logarithmic preferences u(c) = log c, assume that preferences are given by: u(c) = cl -0 - 1 1 - 0 1. Under what condition on o is this an increasing and concave util- ity function? Why should utility be increasing and concave? 2. Show using 4 different methods that: Bu'(ci) u'(co) 1+ 7 3. Replacing out u'(c) by its new expression, compute c1/co- 4. What is the intertemporal budget constraint? 5. Use the intertemporal budget constraint to solve for c and co. 6. Under what condition on o do you obtain the results we had in class when u(c) = logc? Why is that? 7. Assume that o = 1/2, and fo = 0, yo = $90, 000, y1 = 0, 8 = 1. What are co and c if r = 1%? What about if r = 2%? How much does co change then? How much in percentage terms? (Advice: You may use a Google Spreadsheet to do all of these calculations much faster) 8. Same questions if o = 1. (again, use a Spreadsheet) 250 FRANCOIS GEEROLF 9. Same questions if o = 2. (again, use a Spreadsheet) 10. Compare the changes in co following an increase in the real inter- est rate r in questions 7, 8, 9. Comment. 11. Application: real interest rates have gone down substantially recently. Imagine you were starting your first job today. Assuming you are maximizing utility (!), should you save more, or less, for retirement purposes, than if interest rates were higher?A Problem Set 1 A.1 Geometric Sums 1. Calculate the following geometric sum, when x * 1, as a function of x"+ in particular: Er=ltitrt.to =0 2. State a condition on x such that the infinite geometric sum (when 1 -+ co) has a finite value. 3. Assuming that this geometric sum is finite, calculate: I'M & x=ltxtrt.txt... 4. More generally, for m a positive integer, calculate: i=MI 5. What is the present discounted value of an infinite stream of in- comes, which grows at rate g = 2 %, starts at yo = 90000, if the interest rate is i = 3 %?Problems 1. Using the steady state equations for the (u, 0) relation (BC), the job creation condition (JC) and assuming a fixed wage rate w,compare the effects on the steady state levels of u, v and 0 of a smaller labour productivity (Ay 0, a sectoral shock). Formal derivation is not required; graphical analysis with economic explanation is sufficient] 2. Using the two dynamic equations for the unemployment rate u and the degree of tightness of the labour market 0, derive the effect on the steady state of the economy of an anticipated future increase in the wage rate w (firms know at time to that the wage rate will increase permanently at a future date (1). Describe the dynamic adjustment of u, v and o towards the new steady state equilibrium.2. Consider a firm that produces 10 units of gold a year from today. The price of gold next year, Sy, is normally distributed with mean 100 and volatility of 20%. The firm knows that there will be a buyer who is willing to pay the price of 90 per unit, no matter what the value of Sy is. At 7 = 1, the firm can choose whether to sell to this buyer or at the market price of ST. Firm cash flows are taxed at a flat rate of T = 30%. The risk-free rate is Ap = 5% and is compounded annually. Use two decimal places for your answers. (a) [2 points] Express the before-tax cash flows of the (unhedged) firm as a function of the gold spot price Sp. In particular, if we write it as a + b . max{ Sy -90, 0}, what are the values of a and b? (b) [2 points] Now suppose that there is a call option on gold whose strike price is 90. The premium of this option is 17. The firm decides to sell this call option to perfectly hedge its cash flow risk. If the proceeds from selling these options are invested in the risk-free asset and added to the firm profit in one year, what is the before-tax payoff of the hedged firm in one year? (c) [1 point] What is the present value of the cash flows of the hedged unlevered firm after taxes? (d) [3 points] If the hedged firm above issues the maximum amount of safe debt D to take advantage of the fact that the interest payments are tax deductible, what is the total value of the hedged levered firm after tax? How does it compare with your answer above, and why?2. (40) Consider a special type of lookback option whose payoff at maturity is given by the difference between the maximum and minimum stock prices attained over the life of the option. LT = max St - min St te[0,T] te[0,T] Consider pricing this option using the binomial model. Let the current price of stock in Hindsight Inc. be So = 216 and consider an option maturing in n = T = 3 periods, so that At = 1 per period. Suppose for the sake of simplicity that the risk free rate is r = 0, and that each period the stock price either doubles u = 2, or falls by half d = = = ?. (a) (5) Calculate the risk neutral probability of an uptick p. (b) (5) An important property of lookback options not shared by vanilla calls and puts is that they are path dependent. That is, their payoffs depend not only on the final stock price but on how the price moved over the life of the option. Show that this option is path dependent, that is, find two price paths that end at the same price. (c) (15) Draw the stock price tree. Note that there are 2" = 8 possible price paths, in contrast to the n+1 = 4 different final prices. (d) (15) Using backward induction, calculate the initial price of this option















