Provide solutions to these problems.

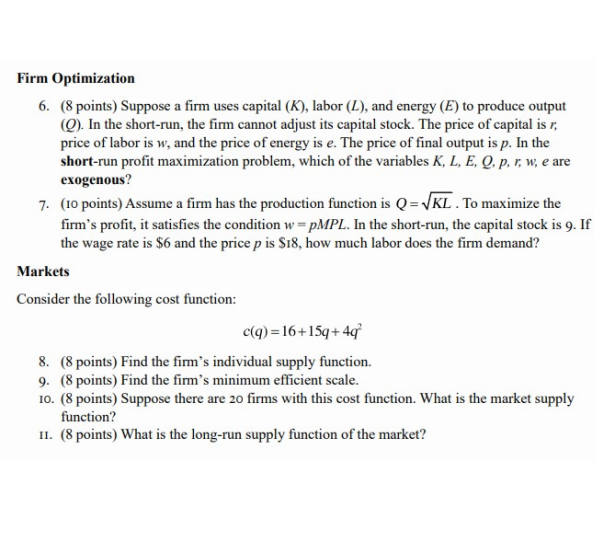
1- The data collected for USA over the period of 1950-2010. The following equation is estimated log(D.)=-2.88+1.06log(Y.) (0.12) (0.293) where D, is defense expenditure and Y is GDP. Standard errors are in parenthesis. Find 95% confidence interval for parameters and interpret the parameter estimates. 2-We aim to fit following model EARNINGS = B, + B.SM + B,SF + B,Math +u where hourly EARNINGS is regressed on a constant, Schooling of Mother (SM), Schooling of Father (SF) and Mathematical Capability (Math). Hourly earnings are measured in dollar, SM and SF are measured in schooling year and Math is measured by exam results. Regression analysis produces following output Dependent Variable: EARNINGS Method: Least Squares Sample: 540 Included observations: 540 Variable Coefficient Std. Error t-Statistic Prob. C -14.65851 3.589279 -4.083972 0.0001 SF 0.668173 0.220817 3.025912 0.0026 SM 0.277970 0.290546 0.956715 0.3391 Math 0.458015 0.066418 6.895906 0.0000 R-squared 0.166245 Mean dependent var 19.71924 Adjusted R-squared 0.161579 S.D. dependent var 14.60151 a-Interpret the parameter estimates (considering the test statistics). b- Comment on the goodness of fit of the model. C- A researcher claims that educated man/women prefer to get married to educated women/men. Suppose this argument is empirically verified for those individuals. Reassess the estimation results in the light of this evidence. d- It is claimed that the total effects of SM and SF on earnings is 1. Explain how you can this claim. Give all the details. d- A researcher calculates parent education as SP=SM+SF. Explain what would happen if the following model is estimated. EARNINGS = 6, + B,SM + B,SF + B,Math + +B,SP+u (2)6. Policy coordination and the world economy Consider an open economy in which the real exchange rate is fixed and equal to one. Consumption, investment, gov- ernment spending, and taxes are given by C - 10 + 0.8(Y - T), I = 10, G = 10, and 7 = 10 Imports and exports are given by IM =0.3Y and X =0.3 Y* where Y denotes foreign output. a. Solve for equilibrium output in the domestic economy, given Y'. What is the multiplier in this economy? If we were to close the economy-so exports and imports were iden- tically equal to zero-what would the multiplier be? Why would the multiplier be different in a closed economy? b. Assume that the foreign economy is characterized by the same equations as the domestic economy (with as- terisks reversed). Use the two sets of equations to solve for the equilibrium output of each country. [Hint: Usethe equations for the foreign economy to solve for Y" as a function of Y and substitute this solution for Y* in part (a).] What is the multiplier for each country now? Why is it different from the open economy multiplier in part (a)? C. Assume that the domestic government, G, has a target level of output of 125. Assuming that the foreign government does not change G' , what is the increase in G necessary to achieve the target output in the domestic economy? Solve for net exports and the budget deficit in each country. d. Suppose each government has a target level of output of 125 and that each government increases government spending by the same amount. What is the common in- crease in G and G necessary to achieve the target output in both countries? Solve for net exports and the budget deficit in each country. e. Why is fiscal coordination, such as the common increase in G and G' in part (d), difficult to achieve in practice?Firm Optimization 6. (8 points) Suppose a firm uses capital (K), labor (L), and energy (E) to produce output (O). In the short-run, the firm cannot adjust its capital stock. The price of capital is r, price of labor is w, and the price of energy is e. The price of final output is p. In the short-run profit maximization problem, which of the variables K, L, E, Q. p. r. w, e are exogenous? 7. (10 points) Assume a firm has the production function is Q= VKL . To maximize the firm's profit, it satisfies the condition w = pMPL. In the short-run, the capital stock is 9. If the wage rate is $6 and the price p is $18, how much labor does the firm demand? Markets Consider the following cost function: c(q) =16+15q+4q 8. (8 points) Find the firm's individual supply function. 9. (8 points) Find the firm's minimum efficient scale. 10. (8 points) Suppose there are 20 firms with this cost function. What is the market supply function? II. (8 points) What is the long-run supply function of the market