
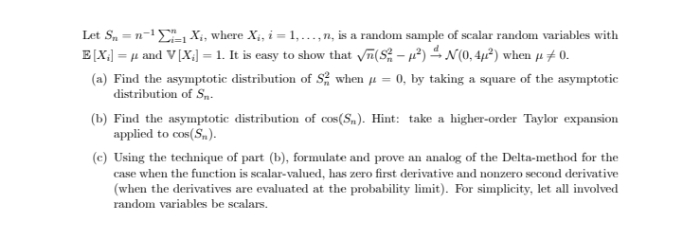
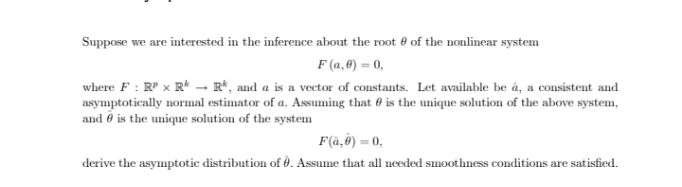


provide solutions using clear format
Suppose that 1 = Britoci, i=1,....n. where &; ~ IID (0, 1) while z; = i for some known A, and of = 6if for some known p. 1. Under what conditions on A and a is the OLS estimator of A consistent? Derive its asymptotic distribution when it is consistent. 2. Under what conditions on A and / is the GLS estimator of A consistent? Derive its asymptotic distribution when it is consistent.Suppose that Hi =atArtui, ASYMPTOTICS OF SAMPLE VARIANCE 19 where {u;} are IID with E [u;] = 0, Eu7] = 0' and E u] = v, while the regressor r, is determin- istically shrinking: I, = p' with pe (0, 1). Let the sample size be n. Discuss as fully as you can the asymptotic behavior of the OLS estimates (a, 8, a') of (a, 8, o') as n - co.Let Sa=n-1)_ Xi, where Xi, i = 1,..., n, is a random sample of scalar random variables with E[Xi] = / and V[X;] = 1. It is easy to show that vn(S; - p?) - N(0, 4p?) when it # 0. (a) Find the asymptotic distribution of S, when / = 0, by taking a square of the asymptotic distribution of Sn. (b) Find the asymptotic distribution of cos(S,,). Hint: take a higher-order Taylor expansion applied to cos(S.). (c) Using the technique of part (b), formulate and prove an analog of the Delta-method for the case when the function is scalar-valued, has zero first derivative and nonzero second derivative (when the derivatives are evaluated at the probability limit). For simplicity, let all involved random variables be scalars.Suppose we are interested in the inference about the root @ of the nonlinear system F (a, 0) = 0, where F : RP x R* - R, and a is a vector of constants. Let available be a, a consistent and asymptotically normal estimator of a. Assuming that O is the unique solution of the above system, and o is the unique solution of the system F(a, 0) = 0, derive the asymptotic distribution of . Assume that all needed smoothness conditions are satisfied.Let c1,..., In be a random sample from a population of r with finite fourth moments. Let f,, and ry be the sample averages of r and r', respectively. Find constants a and b and function c(n) such that the vector sequence c(n) En - a converges to a nontrivial distribution, and determine this limiting distribution. Derive the asymp- totic distribution of the sample variance a2 - (2.)-.Consider a positive (z, y) orthant R. and its unit simplex, i.e. the line segment a ty = 1, x 2 0, y 2 0. Take an arbitrary natural number & E N. Imagine a bug starting creeping from the origin (x, y) = (0,0). Each second the bug goes either in the positive a direction with probability p, or in the positive y direction with probability 1 -p, each time covering distance . Evidently, this way the bug reaches the simplex in & seconds. Suppose it arrives there at point (Ik, yk). Now let k - co, i.e. as if the bug shrinks in size and physical abilities per second. Determine (a) the probability limit of (Ik, Uk); (b) the rate of convergence; (c) the asymptotic distribution of (IA, 4)

















