Question
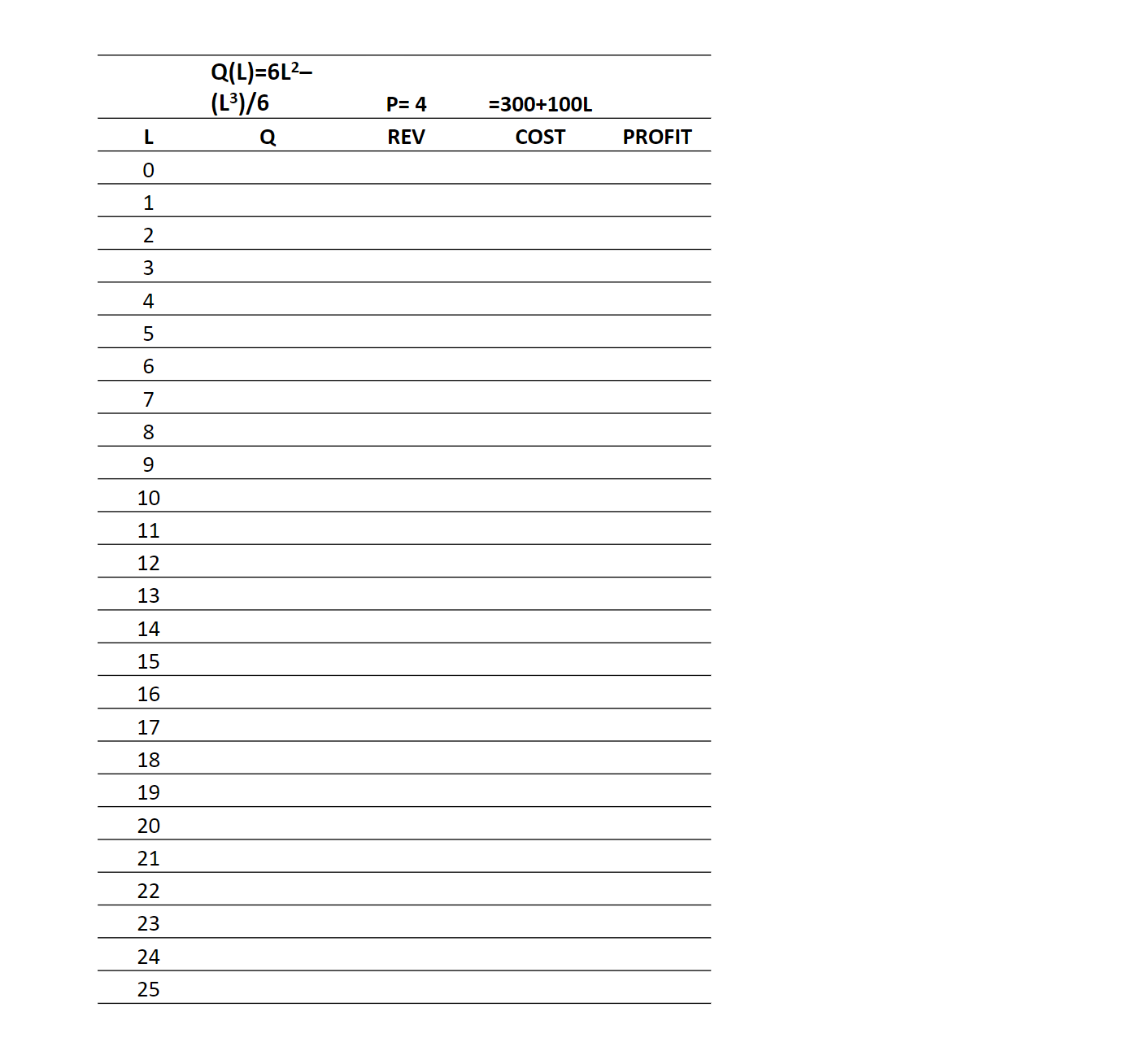
Q1: (10 points) Let Q(L) = 6L2 - (L3)/6 with costs = 300 + 100L. Let the price of output be P = $4. a)
Q1: (10 points) Let Q(L) = 6L2 - (L3)/6 with costs = 300 + 100L. Let the price of output be P = $4. a) Complete the table on the next page. You can excel. b) Graph revenues and costs as a function of Q: Can you explain why is revenue linear in output (a straight line) but costs are not linear. c) Identify the profit maximizing level of L (and hence Q) in your figure. d) Show the break-even level of Labour and Output. That is, what is the minimum level of L and Q that ensure positive profits. Why do we need to raise output high enough to break even? e) Why is it true that, at the optimal output, marginal cost = $4. Give the intuition. f) Suppose P falls to $1.8. Confirm that the firm will choose Q = 0 (eg shut-down). Explain what is happens.
Q2: (10 points) SHORT-RUN COSTS and OPTIMIZATION: Consider the following production function: Q = K1/3 L1/3. Let the price of output be P = $100 and factor prices w = $5 and r = $10. a) Assume that K is fixed at 64. What are the short-run costs? C=wL + r(64)?. b) Plot cost versus output. Show that costs are accelerating. Why is this the case? (hint. What are the returns to scale?) c) What is the firm's optimization problem? d) What is the supply function for this firm? Eg, what is the relationship between market prices and the firm's optimal choice of output. Hint: look at MC = P. Confirm that higher P leads to higher Q. e) Graph the supply curve. Discuss its shape (eg why does the slope increase?).
Q3: (10 points) LONG-RUN COSTS and OPTIMIZATION: Consider a firm that employs capital (K) and labour (L) and uses the production technology Q = 3K + 4L. Let factor prices be r = $40 for capital and w = $20 for labour. a) What is the firm's minimization problem? b) Plot the isoquant for Q =100. What is the Marginal Rate of Technical Substitution (MRTS)? c) For any output Q, what is the optimal combination of K and L given the input prices? Hint set MRTS = r/w and solve for L) d) What is the cost function? Confirm C(Q=100) = 500 e) Given the above, what must the supply curve look like in long-run equilibrium?
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


