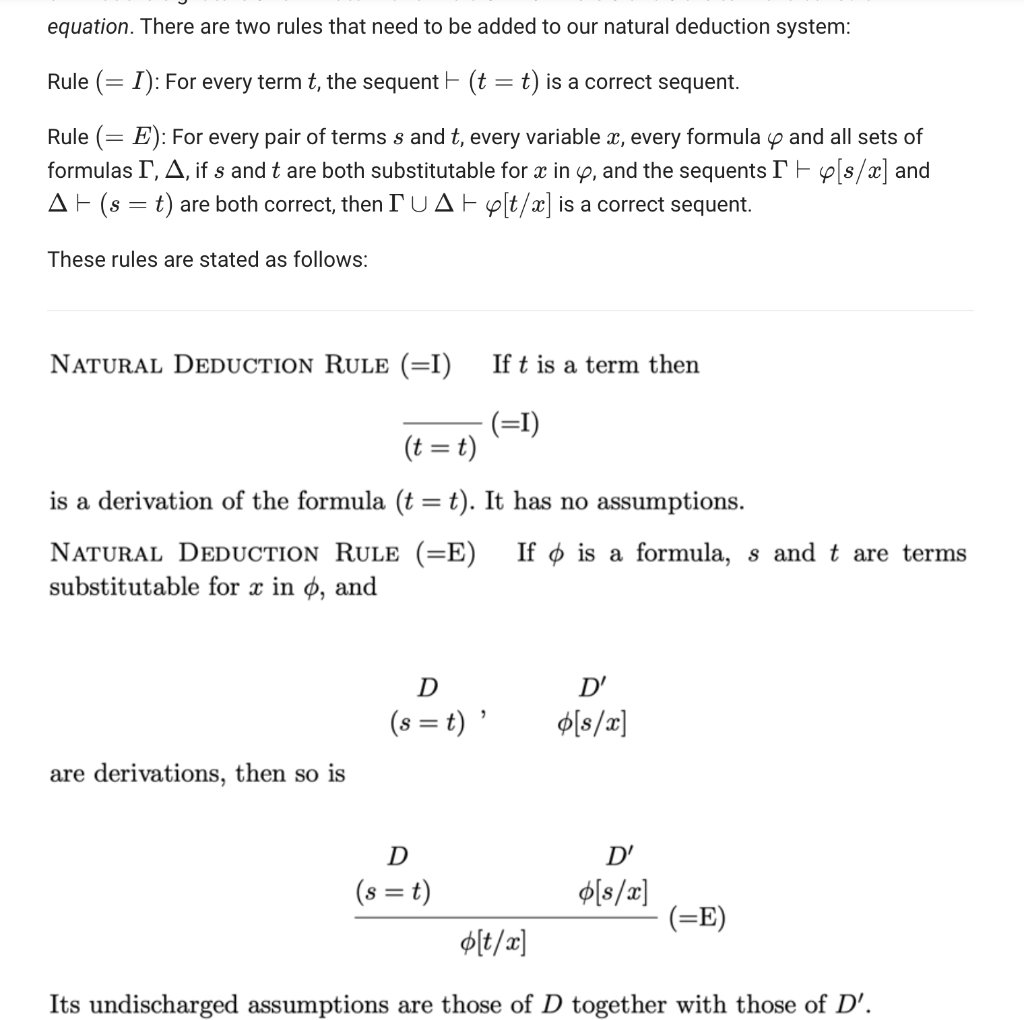
Q3 3 Points S1: For all let n E N, - = 5()) be an axiom. S2: For all n, m e N, let (5() = 5()) + ( = ) be an axiom. Let Is = {4 14 is an instance of S1 or is an instance of S2} Give a derivation to show that: Is +1+2 Please select file(s) Select file(s) equation. There are two rules that need to be added to our natural deduction system: Rule (= I): For every term t, the sequent + (t = t) is a correct sequent. Rule (= E): For every pair of terms s and t, every variable x, every formula y and all sets of formulas T, A, if s and t are both substitutable for x in y, and the sequents T 4 [s/x] and AF(s = t) are both correct, then TU AF [t/x] is a correct sequent. These rules are stated as follows: NATURAL DEDUCTION RULE (=I) If t is a term then (=I) (t = t) is a derivation of the formula (t = t). It has no assumptions. NATURAL DEDUCTION RULE (=E) If is a formula, s and t are terms substitutable for x in 0, and D (s = t)' D' [s/x] are derivations, then so is D (s = t) D' [s/x] (=E) ot/x] Its undischarged assumptions are those of D together with those of D'. Q3 3 Points S1: For all let n E N, - = 5()) be an axiom. S2: For all n, m e N, let (5() = 5()) + ( = ) be an axiom. Let Is = {4 14 is an instance of S1 or is an instance of S2} Give a derivation to show that: Is +1+2 Please select file(s) Select file(s) equation. There are two rules that need to be added to our natural deduction system: Rule (= I): For every term t, the sequent + (t = t) is a correct sequent. Rule (= E): For every pair of terms s and t, every variable x, every formula y and all sets of formulas T, A, if s and t are both substitutable for x in y, and the sequents T 4 [s/x] and AF(s = t) are both correct, then TU AF [t/x] is a correct sequent. These rules are stated as follows: NATURAL DEDUCTION RULE (=I) If t is a term then (=I) (t = t) is a derivation of the formula (t = t). It has no assumptions. NATURAL DEDUCTION RULE (=E) If is a formula, s and t are terms substitutable for x in 0, and D (s = t)' D' [s/x] are derivations, then so is D (s = t) D' [s/x] (=E) ot/x] Its undischarged assumptions are those of D together with those of D