Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Q4. Suppose that A is the set of positive integers. R = {(a,b) | |2a-b| = 1} is a relation on A. Find at
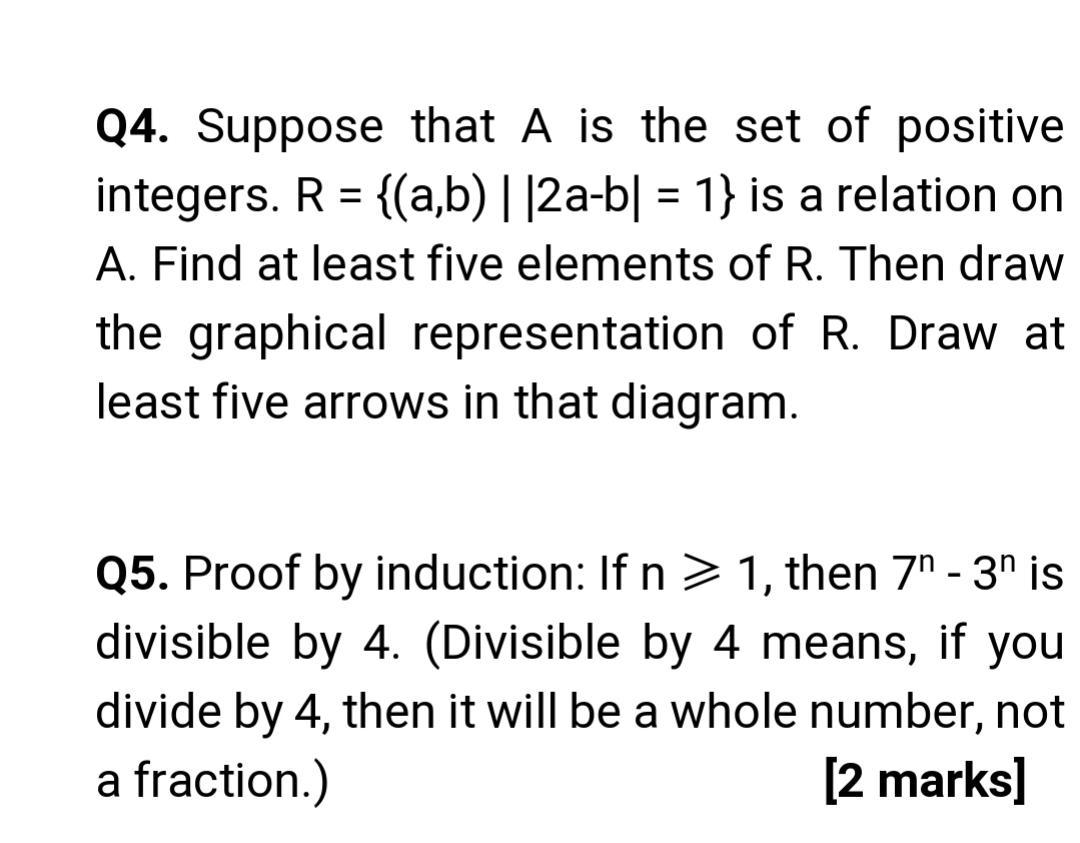
Q4. Suppose that A is the set of positive integers. R = {(a,b) | |2a-b| = 1} is a relation on A. Find at least five elements of R. Then draw the graphical representation of R. Draw at least five arrows in that diagram. Q5. Proof by induction: If n 1, then 7-3n is divisible by 4. (Divisible by 4 means, if you divide by 4, then it will be a whole number, not a fraction.) [2 marks]
Step by Step Solution
★★★★★
3.28 Rating (160 Votes )
There are 3 Steps involved in it
Step: 1
Q4 Relation R on Set A Given the relation R ab mid 2ab 1 on the set of positive integers A Finding E...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
A First Course In Abstract Algebra
Authors: John Fraleigh
7th Edition
0201763907, 978-0201763904
