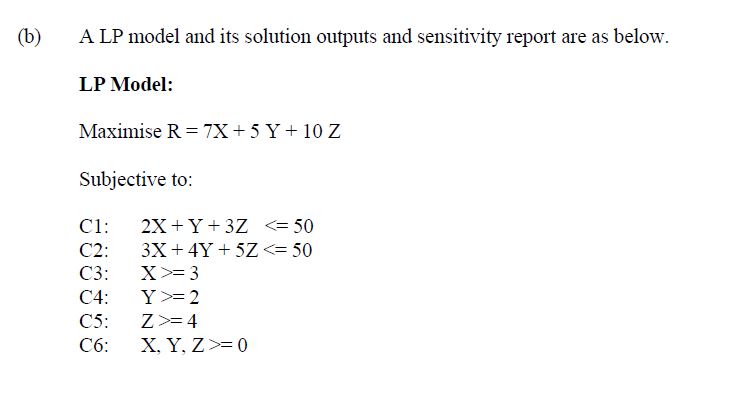
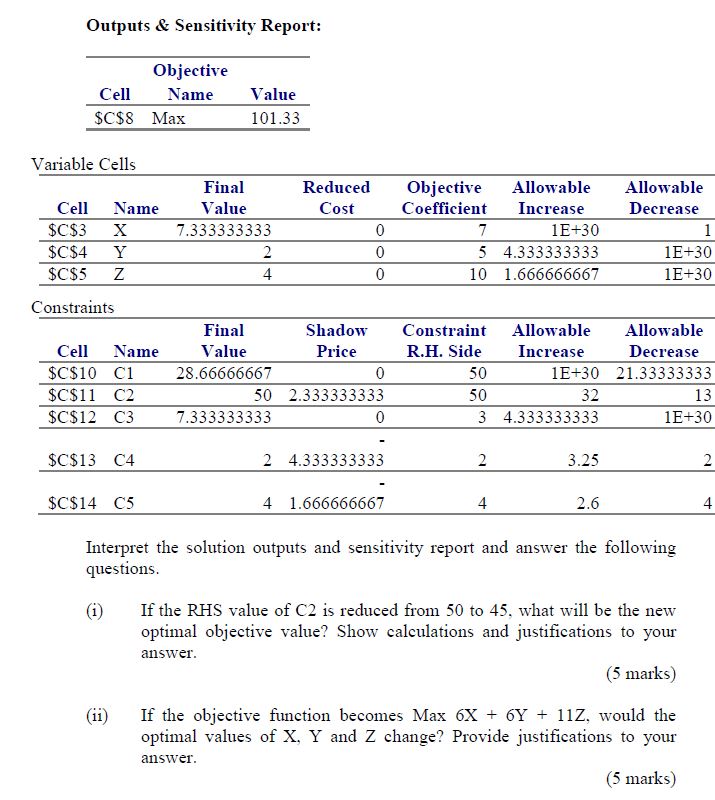
Question 1 (a) A restaurant needs full-time and part-time staffs for daily operation in the year of 2019. The forecasted number of customers for Monday is 80. The forecasted number then increases by 10% each day from Tuesday to Saturday. For example, it is forecasted that there will be 80*110% customers on Tuesday and 80*(110%)- customers on Wednesday, and so on. The forecasted number drops to 70 on Sunday. A full-time staff works for the restaurant throughout 2019. She/he works 7 days a week and is paid $100 a day. A full time staff can serve up to 30 customers a day. Part-time staff are hired on daily basis. A part-time staff is paid $64 a day and can serve up to 20 customers a day. There should be at least one (1) full-time staff. The ratio of full-time staff to part-time staff at any day is at least one to three (1:3). All forecasted demands need to be served. Apply the linear programme (LP) model to determine the number of full-time staffs required for 2019 and the number of part-time staffs required for every day in a week (Monday to Sunday) for 2019. State your model assumption, your objective and decision variables clearly. You only need to formulate the LP model and do not need to solve it. (15 marks)(b) A LP model and its solution outputs and sensitivity report are as below. LP Model: Maximise R = 7X + 5 Y + 10 Z Subjective to: C1: 2X+Y+ 3Z =3 C4: Y >=2 C5: Z >=4 C6: X. Y. Z >=0Outputs & Sensitivity Report: Objective Cell Name Value $C$8 Max 1 0 1 .33 Variable Cells Fin al Reduced Objective Allowable Allowable Cell Na me Value Cost Co efcient Increase Decrease $C$3 X 1333333333 0 7 1E+30 1 $C$4 Y 2 0 5 4.333333333 1E+30 $C$5 Z 4 0 10 \"1.666666661'r 1E+3{} Constraints Fin al Shadow Constraint Allowable Allowable Cell Na me Value Price R.H. Side Increase Decrea sc $C$10 C1 2866666667 0 50 1E+30 2133333333 $C$11 C2. 50 2.333333333 50 32 13 $C$12 C3 1333333333 0 3 4.333333333 1E+39 $C$13 C4 2 4.333333333 2 3.25 2 $C$14 C5 4 1666666667r 4 2.6 4 Interpret the solution outputs and sensitivity report and answer the following questions. (i) If the RHS value of C2 is reduced from 50 to 45. what will be the new optimal objective value? Show calculations and justications to your answer. (5 marks) (ii) If the objective function becomes Max 6X + 6Y + 112, would the optimal values of X Y and Z change? Provide justications to your