Question
Question 1: A particular analyst claims that she is good at picking winners in the US stock market, defined here as stocks with annual returns
Question 1:
A particular analyst claims that she is good at picking "winners" in the US stock market, defined here as stocks with annual returns in the top 10 percent of the population of all stocks in the US. Having heard similar stories many times before, you are skeptical. However, you are willing to give the analyst a chance.
The analyst picks fourteen stocks for you, and a year later four of them are in the top 10%. Assuming that the analyst is not better than chance (i.e., has a 10% chance of picking a winner), what is the probability of observing exactly four winners in a sample of 14? What is the probability of observing at least four winners?
Question 2:
U.S. News & World Report once reported that of the 900 diversified equity funds in existence at the end of the year, only 14 managed to beat the S&P 500 year in, year out, for six years in a row. That is, only 14 of the 900 equity fund managers were able to outperform the S&P 500 in each of the previous six consecutive years.
Suppose that each fund manager uses a strategy that is no more sophisticated than the toss of a coin. That is, a fund manager has exactly a 0.5 probability of beating the S&P 500 in any given year, and the fund managers' performance in any given year is independent of their performance in any of the other years.
What is then the probability that a fund manager will beat the S&P 500 every year over six years? Further, based on this probability, how many of the 900 fund managers would you expect to beat the S&P 500 in six out of six years?
Question 3:
The time it takes a student to go from her house to school is normally distributed with a mean of 20 minutes and a standard deviation of 5 minutes.
Estimate the percentage of the times she will be late for class if she leaves her house 30 minutes before the start of class.
If you were her, how much time would you allow to go to school each day?
Question 4:
An architect designing the gymnasium at a university wants to make the interior doors high enough so that 95% of the male students will have at least a 30 cm clearance.
Assuming that the heights of male students are normally distributed, with a mean of 179 cm and a standard deviation of 8 cm, how high must the architect make the doors?
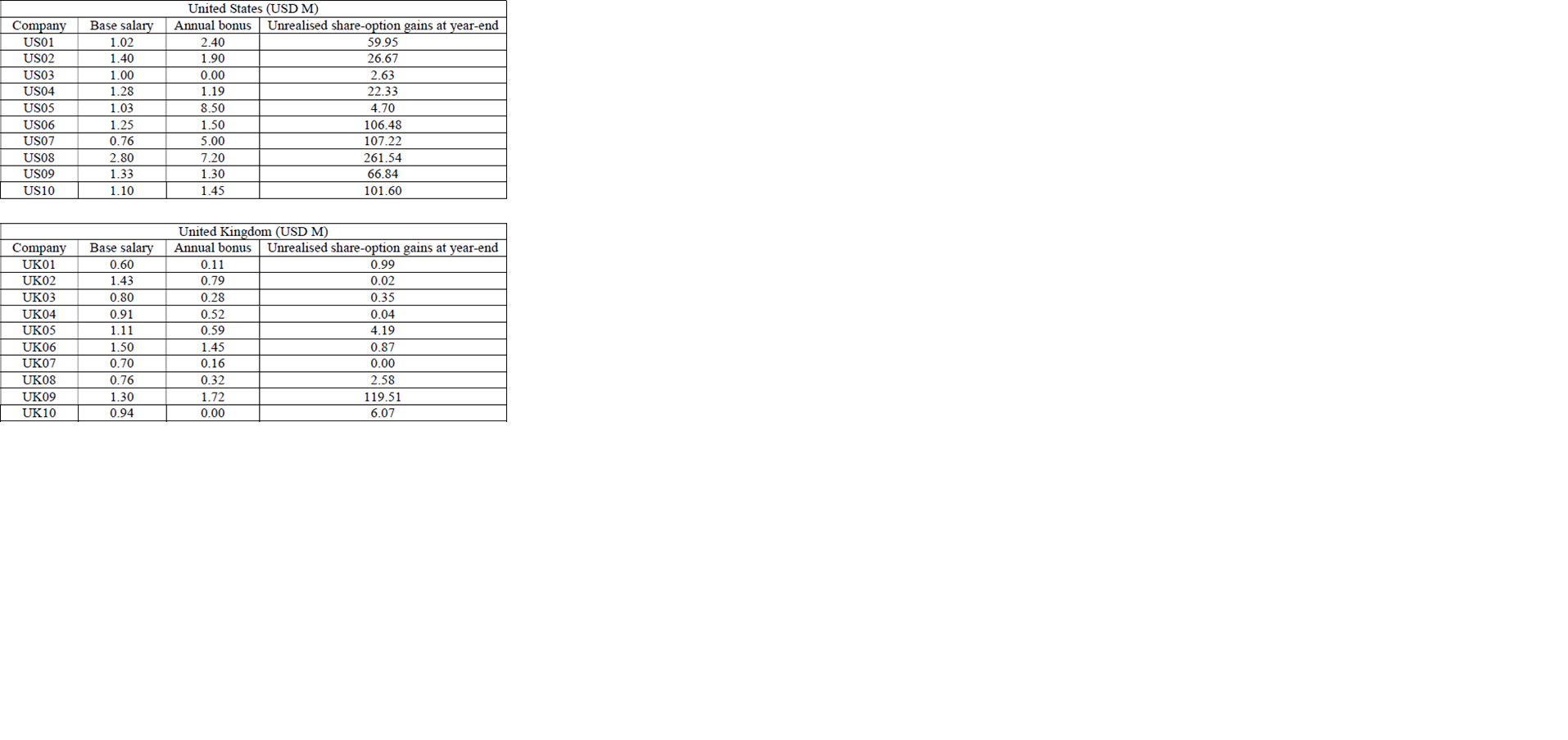
Question 5:
The tables below show the annual base salary, annual bonus, and the unrealized share option gains at year-end for the bosses of selected top companies in the United States and in the United Kingdom.
Where do bosses of top companies get paid more, on average, in the United States or in the United Kingdom? Do the analysis by compensation type (base salary, bonus, share-option gains) and by total compensation.
Does it matter which measure of location (mean and median) you use? Also, compute the measures of dispersion (range, variance, and standard deviation) for the United States and for United Kingdom, by compensation type and by total compensation.
(Note: these tables are available in an Excel file, CEO Compensation.xls, at the course web site.)
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


