



QUESTION 1
Let A, B, C, D, and E be sample space outcomes forming a sample space.Suppose that P(A)=0.25, P(B)=0.05, P(C)=0.3 and P(D)=0.15.
What is the probability of E?P(E) is equal to
a)0.25
b)0.75
c)0.3
d)1
QUESTION 2
Let A and B be mutually exclusive, with P( A)=0.5 and P(B)=0.2
what is the probability of P( A or B)?
a)0.6
b)0
c)0.7
QUESTION 3
Let A and B beindependent, with P( A)=0.5 and P(B)=0.2
what is the probability of P( A or B)?
- 0.7
- 0
- 0.6
QUESTION 4
Let A and B be mutually exclusive, with P( A)=0.5 and P(B)=0.2
what is the probability of P( A and B)?
a)0.7
b)0.6
c)0
d)
QUESTION 5
Let A and B be mutually exclusive, with P( A)=0.5 and P(B)=0.2
what is the probability ofP(B)=?
a)0.8
b)0.7
c)0
QUESTION 6
Let A and B be mutually exclusive, with P( A)=0.5 and P(B)=0.2
what is the probability ofP(A/B)?
a)0.5
b)0.8
c)0
QUESTION 7
Which of the following valuescannotbe?probabilities?
a)-0.6
b)1
c)3/5
d)5/4
e)0
f)0.03
g)1.59
QUESTION 8
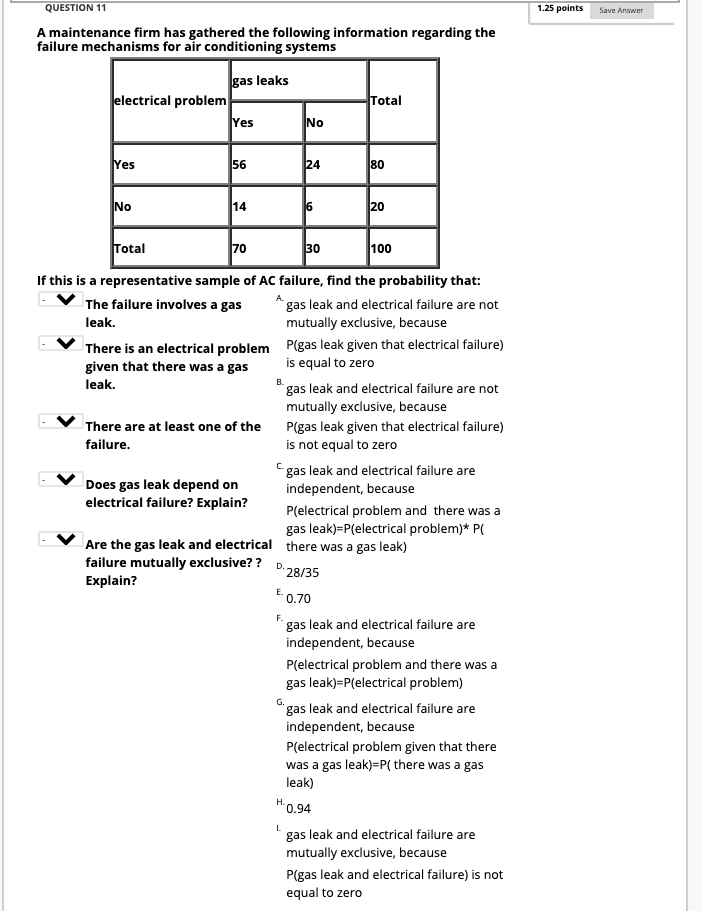
1.
When randomly selecting an
?adult, A denotes the event of selecting someone with blue eyes.
-A.B.
What do P(A) represent?
-A.B.
what P(A ) ?represent?
A.
probability of selecting an adult with blue eyes.
B.
probability of selecting an adult who does not have blue eyes.
QUESTION 9 0.5 points Save Answer When randomly selecting adults, let M denote the event of randomly selecting a male and let B denote the event of randomly selecting someone with blue eyes. - > what does P(M | B) represent? Is A. The probability of getting a male and getting - P(M | B) the same as P(B | M)? someone with blue eyes. B. The probability of getting someone with blue eyes, given that a male has been selected. C. No, because P(B | M) represents the probability of getting someone with blue eyes, given that a male has been selected. D. The probability of getting a male, given that someone with blue eyes has been selected. E. Yes, because P(B | M)represents the probability of getting a male, given that someone with blue eyes has been selected. F. No, because P(B | M)represents the probability of getting a male, given that someone with blue eyes has been selected. G. The probability of getting a male or getting someone with blue eyes.QUESTION 10 1 points Save Answer John and Jane are married. The probability that John watches a certain television show is .4. The probability that Jane watches the show is .5. The probability that John watches the show, given that Jane does, is .7. Find the probability that both John . 0.88 and Jane watch the show. B. 0.55 C. 0.35 Find the probability that Jane D. 0.45 watches the show, given that John does. Find the probability that Jane or John watches the show. Find the probability that neither Jane nor Jhon watch the show.QUESTION 13 It is generally recognized as wise to back up computer data. Assume that there is a 7% rate of disk drive failure in a year. ' V If all ofa computer's data are stored on a A- The probability of total data loss is not single hard disk drive, what is the probability changed by adding backup drives. that the drive will fail during a year? B. The probability of total data loss is ' V If all of a computer's data are stored on a substantially reduced by adding backup hard disk drive with a copy stored in a drives. second hard disk drive, what is the c 0 07 probability that both drives will fail during a " ' year? D 0.93 - V If all of a computer's data is stored on three 5- The Probability Of total data loss iS not independent hard disk drivesnwhat' is the changed by adding one backup drive, but it is probability that all three wrll fail during a dramatically reduced by adding two backup year? drives. F- 00049 ' V Describe the improved reliability that is 50000343 gained W'th backup drives. H.The probability of total data loss is slightly - V If all of a computer's data are stored on a increased by adding backup drives, single hard disk drive, what is the probability that the drive will not fail during a year? 1.25 points Save Answer QUESTION 14 1.5 points Save Answer To reduce laboratory costs. water samples from two public swimming pools are combined for one test for the presence of bacteria. Further testing is done only if the combined sample tests positive. Based on past results, there is a 0.008 probability of nding bacteria in a public swimming area. ' V Find the probability that a combined A001 59 sample from two public swimming areas 3 . . 01819 wull reveal the presence of bacteria. C'0.0238 ' V Find the probability that a combined sample from three public swimming areas will reveal the presence of bacteria ' " Find the probability that a combined sample from twenty five public swimming areas will reveal the presence of bacteria. QUESTION 11 1.25 points Save Answer A maintenance firm has gathered the following information regarding the failure mechanisms for air conditioning systems gas leaks electrical problem Total Yes No Yes 56 24 80 No 14 20 Total 70 30 100 If this is a representative sample of AC failure, find the probability that: V The failure involves a gas *gas leak and electrical failure are not leak. mutually exclusive, because V There is an electrical problem P(gas leak given that electrical failure) given that there was a gas is equal to zero leak. "gas leak and electrical failure are not mutually exclusive, because V There are at least one of the P(gas leak given that electrical failure) failure. is not equal to zero gas leak and electrical failure are Does gas leak depend on independent, because electrical failure? Explain? P(electrical problem and there was a V gas leak)=P(electrical problem)* P( Are the gas leak and electrical there was a gas leak) failure mutually exclusive? ? D. 28/35 Explain? E. 0.70 gas leak and electrical failure are independent, because P(electrical problem and there was a gas leak)=P(electrical problem) G . gas leak and electrical failure are independent, because P(electrical problem given that there was a gas leak)=P( there was a gas leak) H- 0.94 gas leak and electrical failure are mutually exclusive, because P(gas leak and electrical failure) is not equal to zeroQUESTION 12 joe is considering pursuing an MBA degree. He has applied to two different universities. The acceptance rate for applicants with similar qualications is 25 percent for University A and 40 percent for University B. ' v Is the acceptance decision at University A independent of the acceptance decision at University B? What is the probability thatjoe will be accepted at both universities? What is the probability thatJoe will be accepted at University A and rejected at University B? What is the probability thatjoe will not be accepted at either university? What is the probability thatJoe will be accepted by at least one of the two universities? What is the probability thatJoe will be accepted at one, and only one, university? A0.45 3' the acceptance decision at University A is independent of the acceptance decision at University 3 c0.10 D121.55 E0.15 F' the acceptance decision at University A is dependent of the acceptance decision at University 3 G111.45 1.5 points Save


















