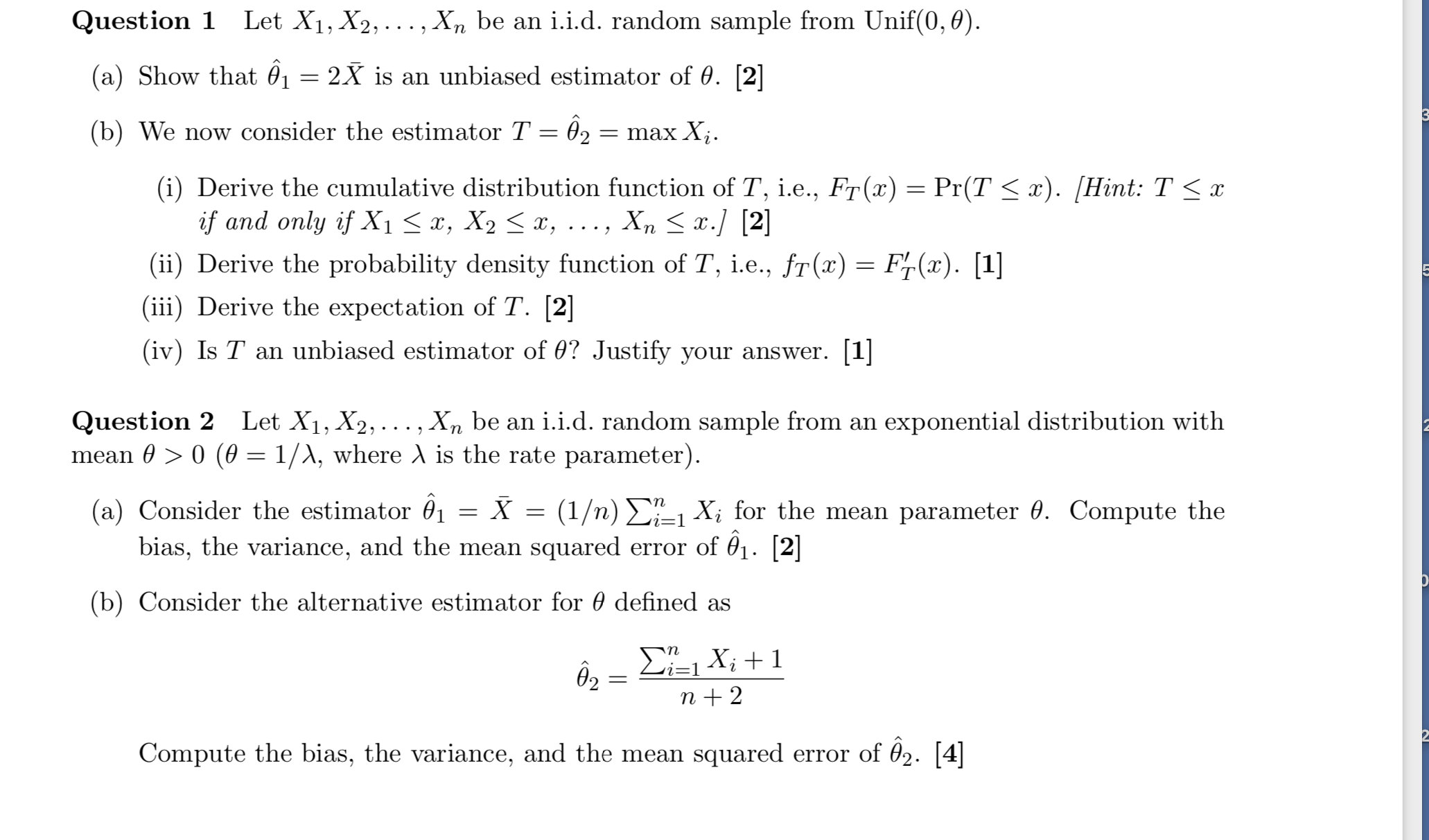
Question 1 Let X1, X2,..., Xn be an i.i.d. random sample from Unif(0, 0). (a) Show that = = 2X is an unbiased estimator of 0. [2] (b) We now consider the estimator T = 02 = max Xi. (i) Derive the cumulative distribution function of T, i.e., FT(x) = Pr(T x). [Hint: T x if and only if X x, X2 x, , Xn x.] [2] ... (ii) Derive the probability density function of T, i.e., (x) = F(x). [1] (iii) Derive the expectation of T. [2] (iv) Is T an unbiased estimator of 0? Justify your answer. [1] Question 2 Let X1, X2,..., X be an i.i.d. random sample from an exponential distribution with mean 0 > 0 (0 = 1/, where X is the rate parameter). i=1 Xi (a) Consider the estimator = X = (1/n) 1X; for the mean parameter 0. Compute the bias, the variance, and the mean squared error of . [2] (b) Consider the alternative estimator for defined as _1X + 1 = n+2 Compute the bias, the variance, and the mean squared error of 02. [4] 3