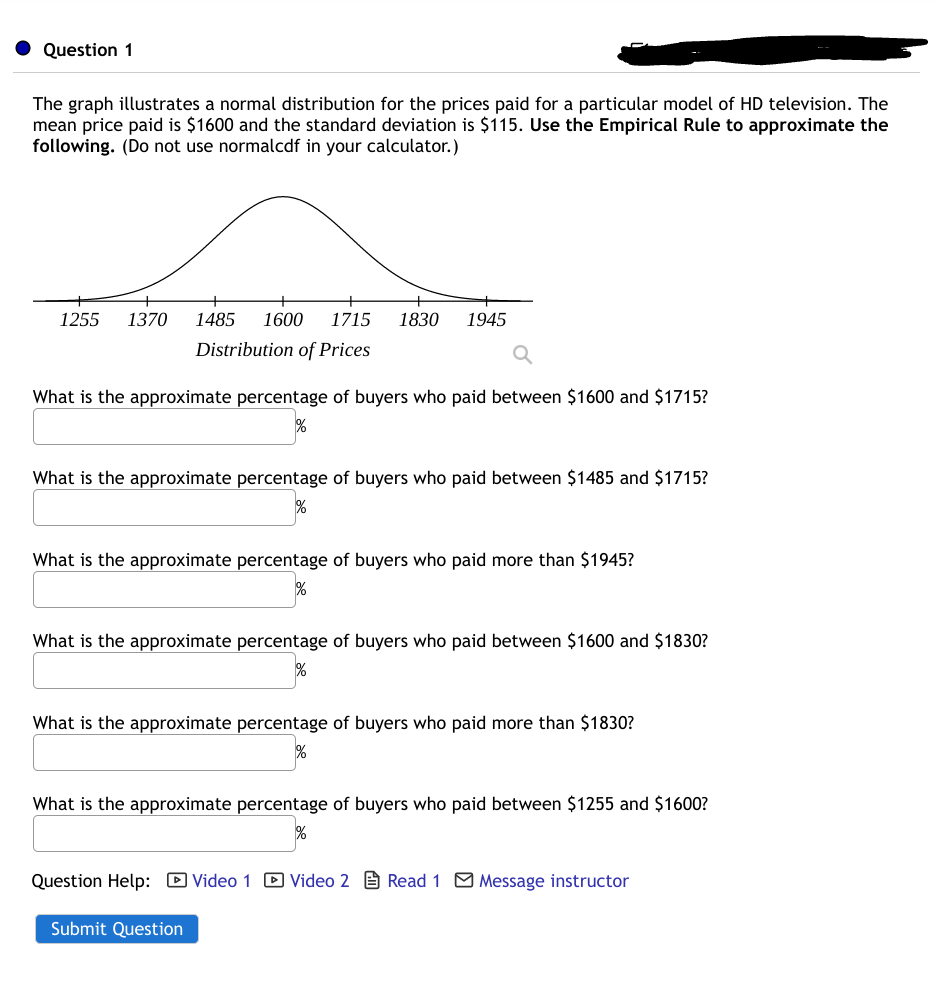
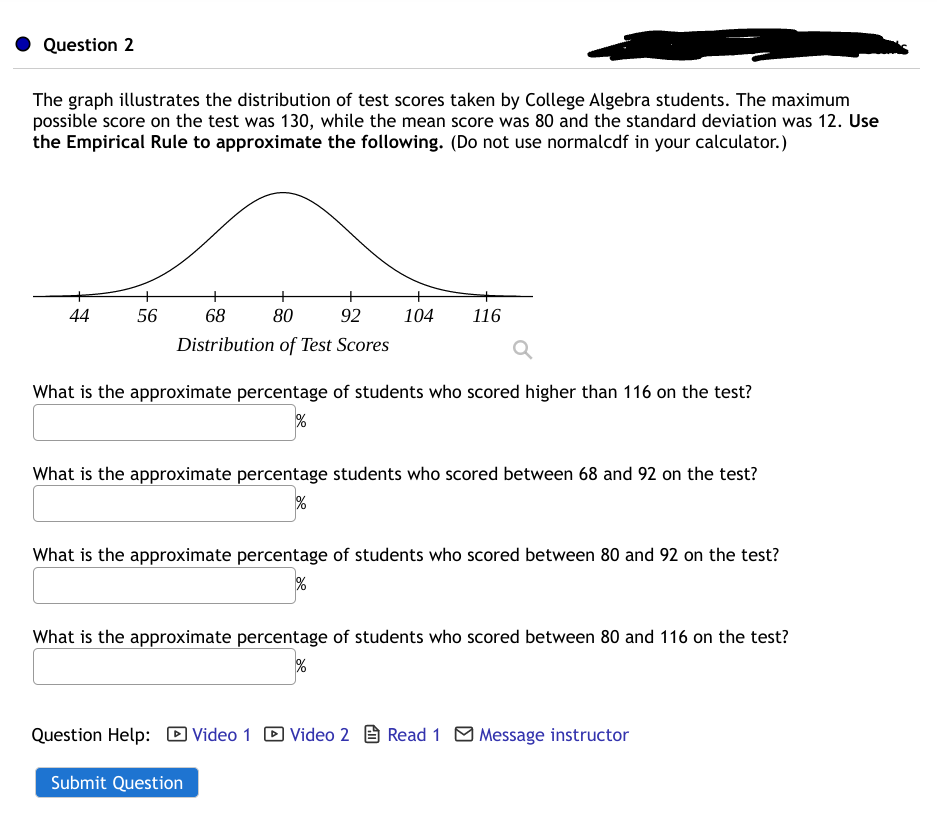
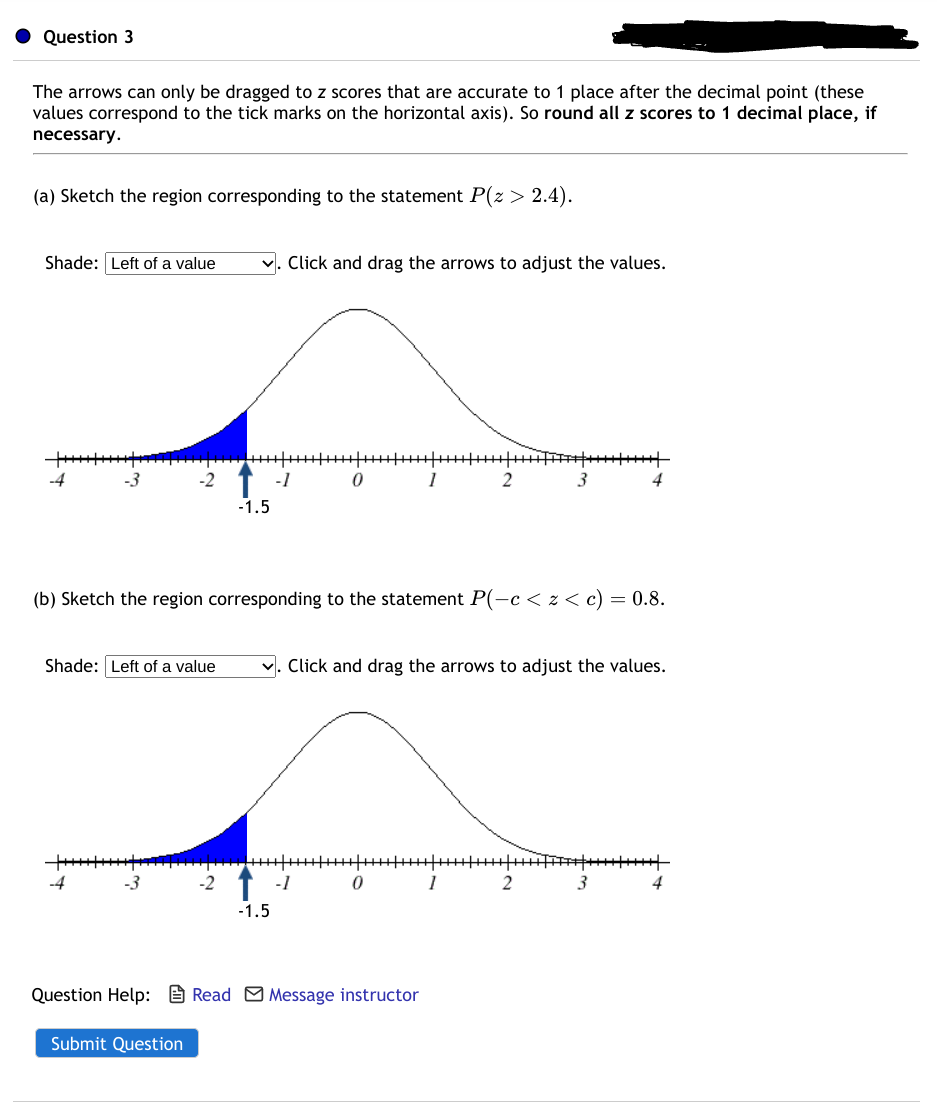
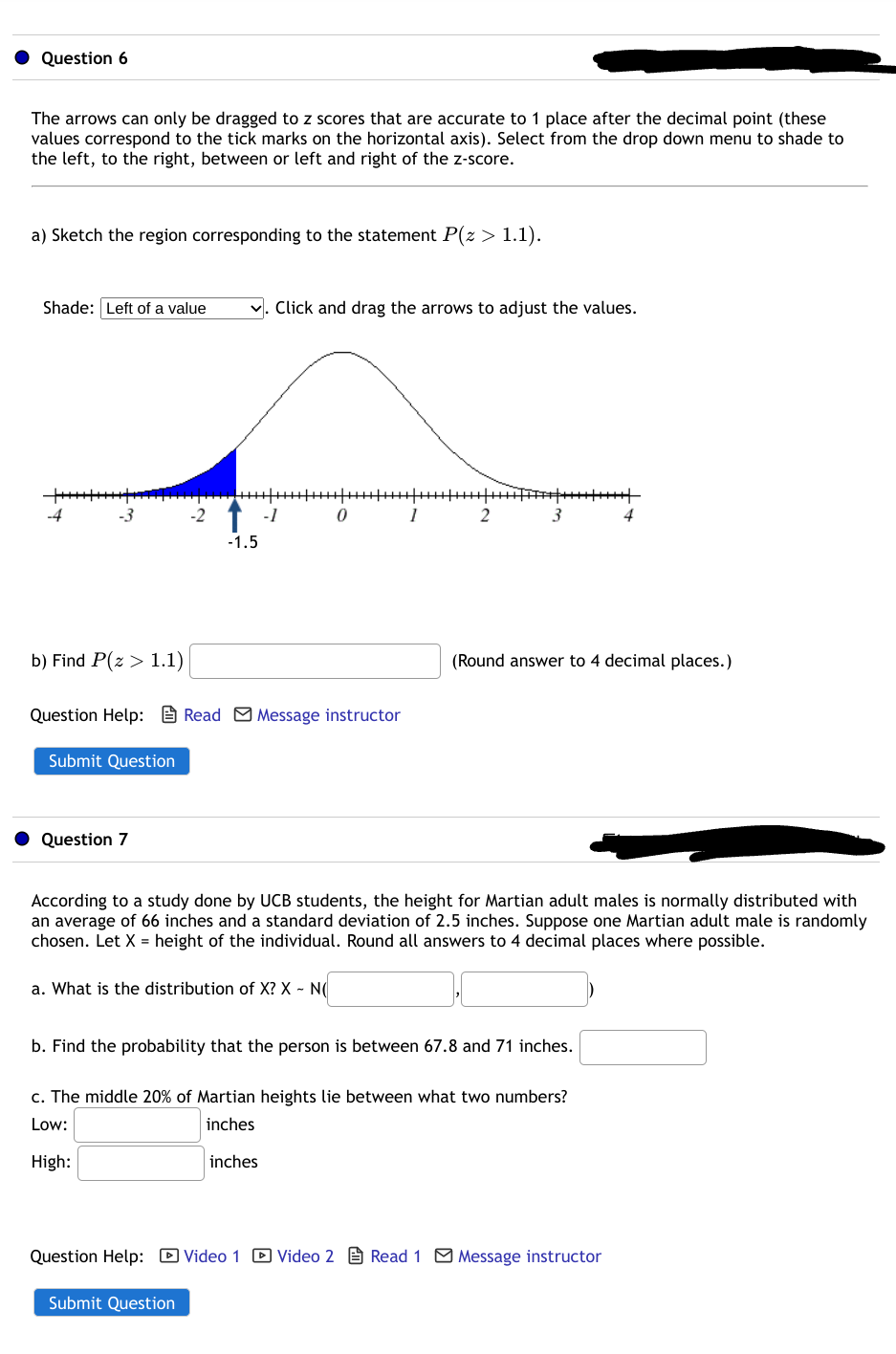
Question 1 The graph illustrates a normal distribution for the prices paid for a particular model of HD television. The mean price paid is $1600 and the standard deviation is $115. Use the Empirical Rule to approximate the following. (Do not use normalcdf in your calculator.) 1255 1370 1485 1600 1715 1830 1945 Distribution of Prices What is the approximate percentage of buyers who paid between $1600 and $1715? What is the approximate percentage of buyers who paid between $1485 and $1715? % What is the approximate percentage of buyers who paid more than $1945? % What is the approximate percentage of buyers who paid between $1600 and $1830? What is the approximate percentage of buyers who paid more than $1830? What is the approximate percentage of buyers who paid between $1255 and $1600? Question Help: Video 1 Video 2 Read 1 Message instructor Submit Question Question 2 The graph illustrates the distribution of test scores taken by College Algebra students. The maximum possible score on the test was 130, while the mean score was 80 and the standard deviation was 12. Use the Empirical Rule to approximate the following. (Do not use normalcdf in your calculator.) 44 56 80 68 Distribution of Test Scores 92 104 116 What is the approximate percentage of students who scored higher than 116 on the test? % What is the approximate percentage students who scored between 68 and 92 on the test? % What is the approximate percentage of students who scored between 80 and 92 on the test? % What is the approximate percentage of students who scored between 80 and 116 on the test? % Question Help: Video 1 Video 2 Read 1 Message instructor Submit Question Question 3 The arrows can only be dragged to z scores that are accurate to 1 place after the decimal point (these values correspond to the tick marks on the horizontal axis). So round all z scores to 1 decimal place, if necessary. (a) Sketch the region corresponding to the statement P(x > 2.4). Shade: Left of a value . Click and drag the arrows to adjust the values. -4 -3 -2 -1 0 2 3 4 -1.5 (b) Sketch the region corresponding to the statement P(-c Question 4 Using the standard normal distribution, find the two z-scores that that form the middle shaded region. The shaded region is symmetric about 2 = 0. Round your z-scores to two decimal places. Shaded Area = 0.64 -4 -3 -2 -1 -Z Negative z-score = Positive z-score = 0 ON 17 2 3 Question Help: Read Message instructor Submit Question In the video provided in these questions, note that he uses -99999 and 99999 for - and respectively, rather than -1E99 and 1E99, which is perfectly acceptable, but differs from what is taught in the textbook. Question 5 Sketch the region corresponding to the statement P(z < -0.2) Shade: Left of a value Click and drag the arrows to adjust the values. -1 0 2 3 4 -1.5 Sketch the region corresponding to the statement P(-c < z < c) = 0.4. Shade: Left of a value . Click and drag the arrows to adjust the values. -4 -1 0 2 3 4 -1.5 Question 6 The arrows can only be dragged to z scores that are accurate to 1 place after the decimal point (these values correspond to the tick marks on the horizontal axis). Select from the drop down menu to shade to the left, to the right, between or left and right of the z-score. a) Sketch the region corresponding to the statement P(z > 1.1). Shade: Left of a value . Click and drag the arrows to adjust the values. -4 -3 -2 -1 0 1 2 3 4 -1.5 b) Find P(1.1) Question Help: Read Message instructor Submit Question (Round answer to 4 decimal places.) Question 7 According to a study done by UCB students, the height for Martian adult males is normally distributed with an average of 66 inches and a standard deviation of 2.5 inches. Suppose one Martian adult male is randomly chosen. Let X = height of the individual. Round all answers to 4 decimal places where possible. a. What is the distribution of X? X - N b. Find the probability that the person is between 67.8 and 71 inches. c. The middle 20% of Martian heights lie between what two numbers? Low: High: inches inches Question Help: Video 1 Video 2 Read 1 Message instructor Submit Question