
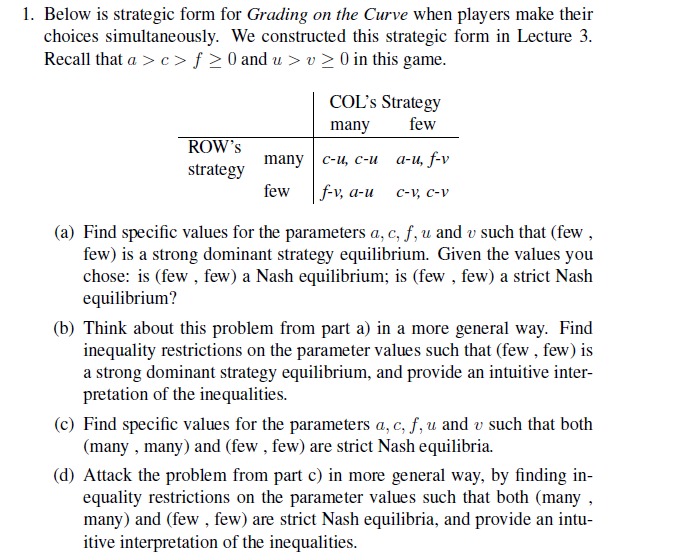
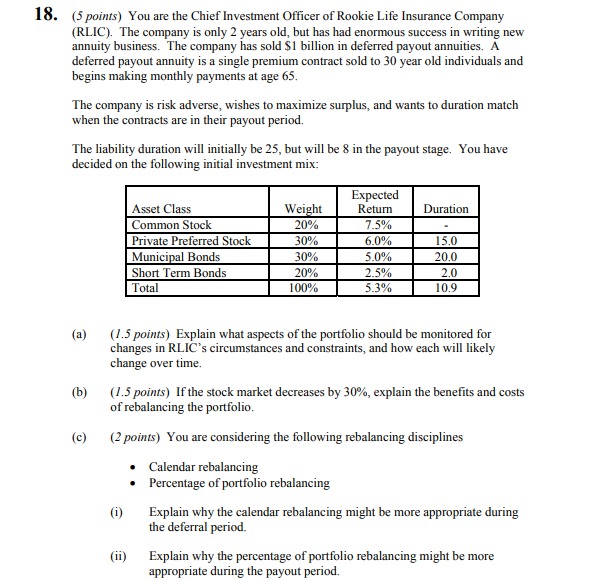


Question 1 (total 15 points}. A rm has a production function: f (K . L} = EDLKD'E + LEE 2 LEKE a. What is its short-run production function if capital is xed at K=4'? b. What are the fum's marginal product of labour and average product of labour in the short run? c. Calculate the elasticity of output with respect to labour. Question 2 (total 16 points} . Do the following functions exhibit increasing, constant, or decreasing retiirns to scale? Explain you: answers. a. The production ftmction Q = Wigwam, where M is materials, K is capital and L is laboUr. b. q = L + 0.5K c. u = DELHI\" I d. if = M.3 + 4K Question 3 (12 points]. Find the Marginal Rate of Technical Substitution for the following production functions: 1 | a. 'FLEKE 11 q = L.5 + [(0.5 o. q = L + K Question 4 (Total It} points). Suppose a fum's cost uiction is C = 2q3 lql + Slq. average cost curve is described by the equation AC = 2432 lq + 94). At what output level does the marginal cost curve cross the average cost curve? Question 5. (16 points, 8 points each} For the following, please answer \"True" or "False" and explain why. a. When buying a piece of equipment, it is always bed for the rm to pay cash instead of borrowing the funds since this mnders the equipment less costly. b. University of Tomato is choosing a location for a new building. The university has campuses at Downtown1 Scarborough and Mississauga. A large parcel of land would have to be purchased at Scarborough or Mississauga if the building were to Jugs L be built there. So it is cost-effective to locate the new building at downtown because the university already owns the land. 1. Below is strategic form for Grading on the Curve when players make their choices simultaneously. We constructed this strategic form in Lecture 3. Recall that a > c > f 2 0 and u > v 2 0 in this game. COL's Strategy many few ROW's many strategy C-u, C-u a-u, f-v few f-v, a-u c-V, C-V (a) Find specific values for the parameters a, c, f, u and v such that (few , few) is a strong dominant strategy equilibrium. Given the values you chose: is (few , few) a Nash equilibrium; is (few , few) a strict Nash equilibrium? (b) Think about this problem from part a) in a more general way. Find inequality restrictions on the parameter values such that (few , few) is a strong dominant strategy equilibrium, and provide an intuitive inter- pretation of the inequalities. (c) Find specific values for the parameters a, c, f, u and v such that both (many , many) and (few , few) are strict Nash equilibria. (d) Attack the problem from part c) in more general way, by finding in- equality restrictions on the parameter values such that both (many , many) and (few , few) are strict Nash equilibria, and provide an intu- itive interpretation of the inequalities.18. (5 points) You are the Chief Investment Officer of Rookie Life Insurance Company (RLIC). The company is only 2 years old, but has had enormous success in writing new annuity business. The company has sold $1 billion in deferred payout annuities. A deferred payout annuity is a single premium contract sold to 30 year old individuals and begins making monthly payments at age 65. The company is risk adverse, wishes to maximize surplus, and wants to duration match when the contracts are in their payout period. The liability duration will initially be 25, but will be 8 in the payout stage. You have decided on the following initial investment mix: Expected Asset Class Weight Return Duration Common Stock 20% 7.5% Private Preferred Stock 30% 5.0% 15.0 Municipal Bonds 30% 5.0% 20.0 Short Term Bonds 20% 2.5% 2.0 Total 100% 5.3% 10.9 (a) (1.5 points) Explain what aspects of the portfolio should be monitored for changes in RLIC's circumstances and constraints, and how each will likely change over time. (b) (1.5 points) If the stock market decreases by 30%, explain the benefits and costs of rebalancing the portfolio. (c) (2 points) You are considering the following rebalancing disciplines . Calendar rebalancing Percentage of portfolio rebalancing (i) Explain why the calendar rebalancing might be more appropriate during the deferral period. (ii) Explain why the percentage of portfolio rebalancing might be more appropriate during the payout period.I. A nn's production function is given by is q = KL 0.8K2 0.2!,2 where q is the quantity of output produced, I. is the amount of labor input used, and K is the amount of capital input used. Suppose the amount of capital is fixed at 10 units of capital in the short run. I. 2. Find this finn's short run (a) total product of labor function, (b) average product of labor function, and (c) marginal product of labor function. At what level of labor input usage does the marginal productivity of labor reach a maximum? How much output is produced at that point? Show and briey discuss how you arrived at your answer. Is the marginal product of labor ever negative for this production function? If so, when. If not why not. Show how you arrived at your answer. At what level of labor input usage does the average productivity of labor reach a maximum? How much output is pmduced at that point? Show and briey discuss how you arrived at your answer. At what level of labor input usage does the total productivity of labor reach a. maximum? How much output is produced at that point? Show and briey discuss how you arrived at your answer. What is the economic region of the short run production function you found in part I? Show and briey explain how you arrived at your answer. Calculate the output elasticity of labor when this rm uses the amount of labor at which its average product of labor is maximum. In a carefully labeled diagram, graphically illustrate this rm's total product of labor in the upper panel and the marginal product of labor and average product of labor in the lower panel using your results in parts 1 to 4. Consider a two-period economy that has at the beginning of period 1 external wealth of 100. GDP in both periods is 120. The interest rate in periods 1 and 2 is 10 percent. In period 1, the country runs a current account decit of 5 percent of GDP. At the end of period 2, the external wealth must be equal to zero. 1. Find the level of the trade balance in period 1, the level of the current account balance in period 1, and the country's external wealth at the beginning of period 2. 2. Is the country living beyond its means? To answer this question, nd the country's current account balance in period 2 and the associated trade balance in period 2. Is this value for the trade balance feasible? [Hintz Keep in mind that since expenditures cannot be negative, the trade balance cannot exceed GDP]. 3. Now assume that in period 1, the country runs instead a much larger current account decit of 10 percent of GDP. Find the country's external wealth at the end of period 1. Is the country living beyond its means? If so, why? 4. What is the maximum current account decit (as a share of GDP) that this country can have at time 1 and still live within its means
















