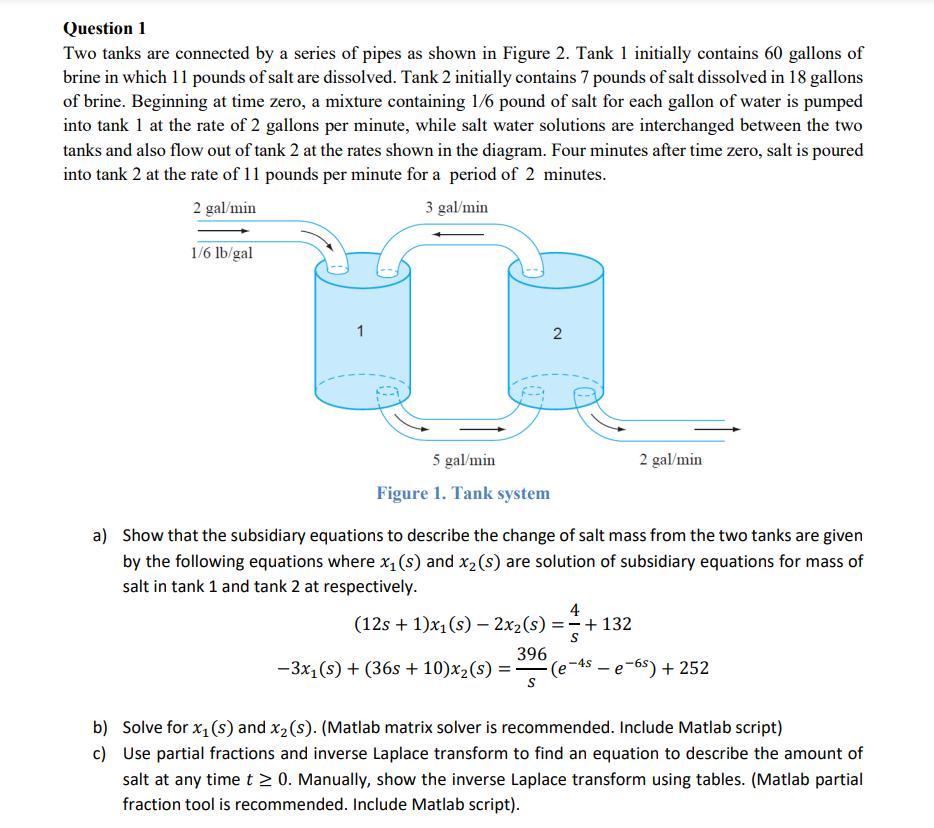
Question 1 Two tanks are connected by a series of pipes as shown in Figure 2. Tank 1 initially contains 60 gallons of brine in which 11 pounds of salt are dissolved. Tank 2 initially contains 7 pounds of salt dissolved in 18 gallons of brine. Beginning at time zero, a mixture containing 1/6 pound of salt for each gallon of water is pumped into tank 1 at the rate of 2 gallons per minute, while salt water solutions are interchanged between the two tanks and also flow out of tank 2 at the rates shown in the diagram. Four minutes after time zero, salt is poured into tank 2 at the rate of 11 pounds per minute for a period of 2 minutes. 2 gal/min 1/6 lb/gal 3 gal/min 1 2 5 gal/min Figure 1. Tank system 2 gal/min a) Show that the subsidiary equations to describe the change of salt mass from the two tanks are given by the following equations where x (s) and x2(s) are solution of subsidiary equations for mass of salt in tank 1 and tank 2 at respectively. 4 == +132 S (12s+1)x1(s) 2x(s) = - 396 (e-4s-e-6s) + 252 S -3x1(s) + (36s+ 10)x2(s) = b) Solve for x1 (s) and x2 (s). (Matlab matrix solver is recommended. Include Matlab script) c) Use partial fractions and inverse Laplace transform to find an equation to describe the amount of salt at any time t 0. Manually, show the inverse Laplace transform using tables. (Matlab partial fraction tool is recommended. Include Matlab script).