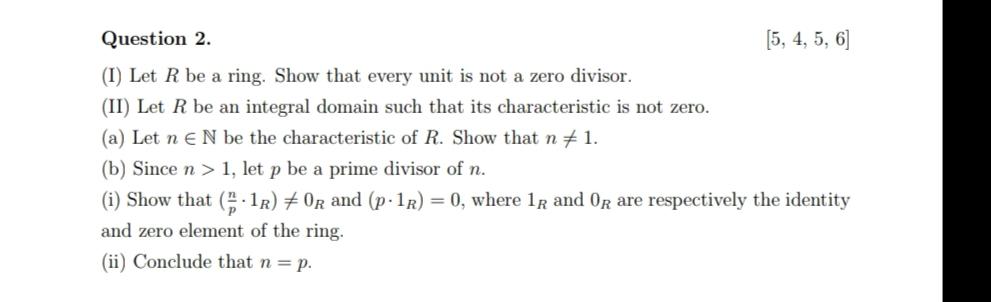
Question 2. [5, 4, 5, 6] (I) Let R be a ring. Show that every unit is not a zero divisor. (II) Let R be an integral domain such that its characteristic is not zero. (a) Let n eN be the characteristic of R. Show that n + 1. (b) Since n > 1, let p be a prime divisor of n. (i) Show that ( 1R) # OR and (p-1R) = 0, where 1R and OR are respectively the identity and zero element of the ring. (ii) Conclude that n = p.