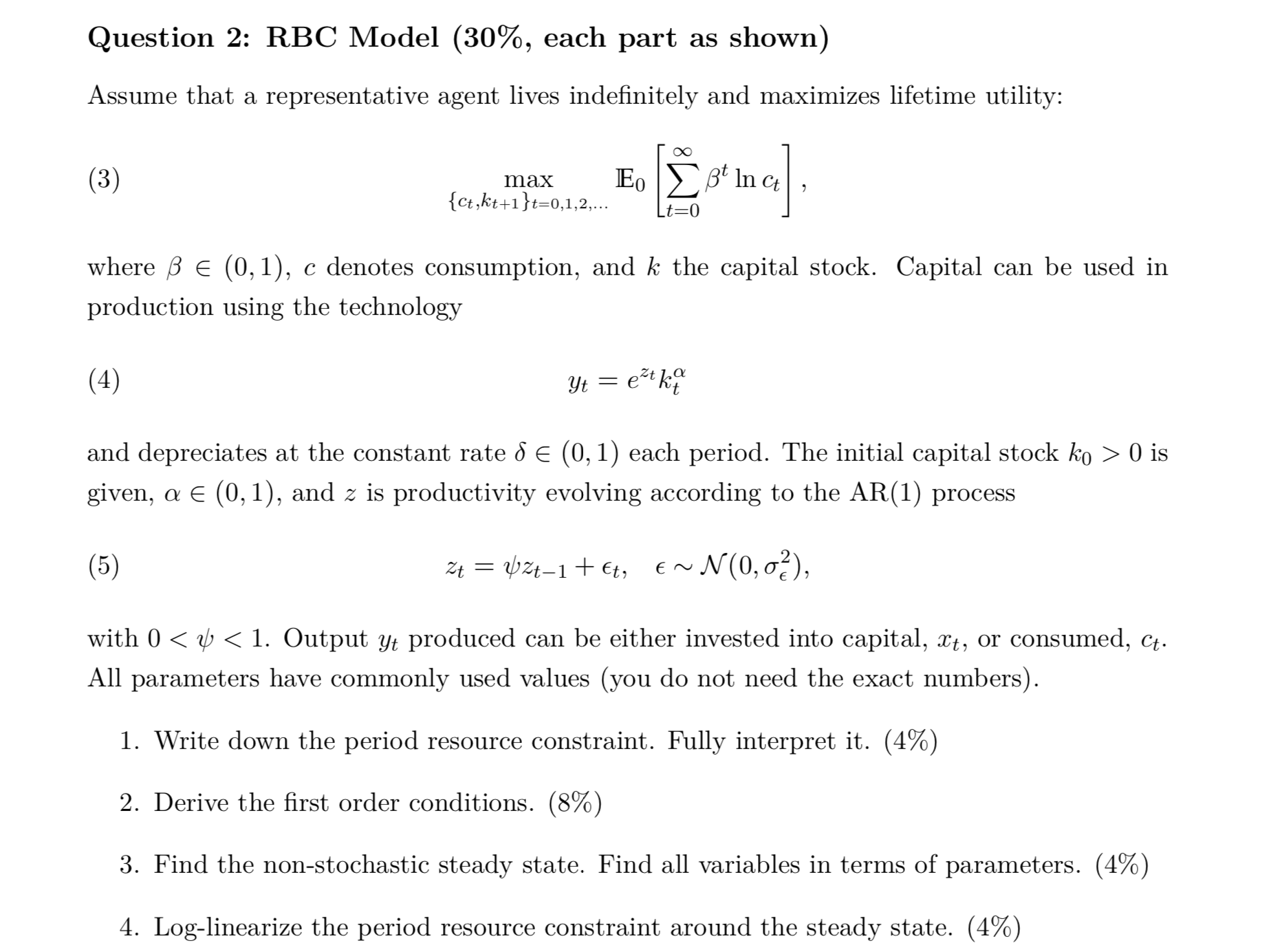
Question 2: RBC Model (30%, each part as shown)

Assume that a representative agent lives indefinitely and maximizes lifetime utility:

??? (3) max E0 ??tlnct ,

{ct ,kt+1 }t=0,1,2,...

where ? ? (0,1), c denotes consumption, and k the capital stock. Capital can be used in

production using the technology

(4) yt =eztkt?

and depreciates at the constant rate ? ? (0, 1) each period. The initial capital stock k0 > 0 is given, ? ? (0, 1), and z is productivity evolving according to the AR(1) process

(5) zt=?zt?1+?t, ??N(0,??2),

with 0

All parameters have commonly used values (you do not need the exact numbers).

1. Write down the period resource constraint. Fully interpret it. (4%)
2. Derive the first order conditions. (8%)
3. Find the non-stochastic steady state. Find all variables in terms of parameters. (4%)
4. Log-linearize the period resource constraint around the steady state. (4%)
5. Assume the economy is in steady state for t 0. Would you expect capital and consumption to deviate from their steady state values in response to this shock? If so, how do you expect them to deviate over time and why? What is the intuition? What happens in the long run? (10%)

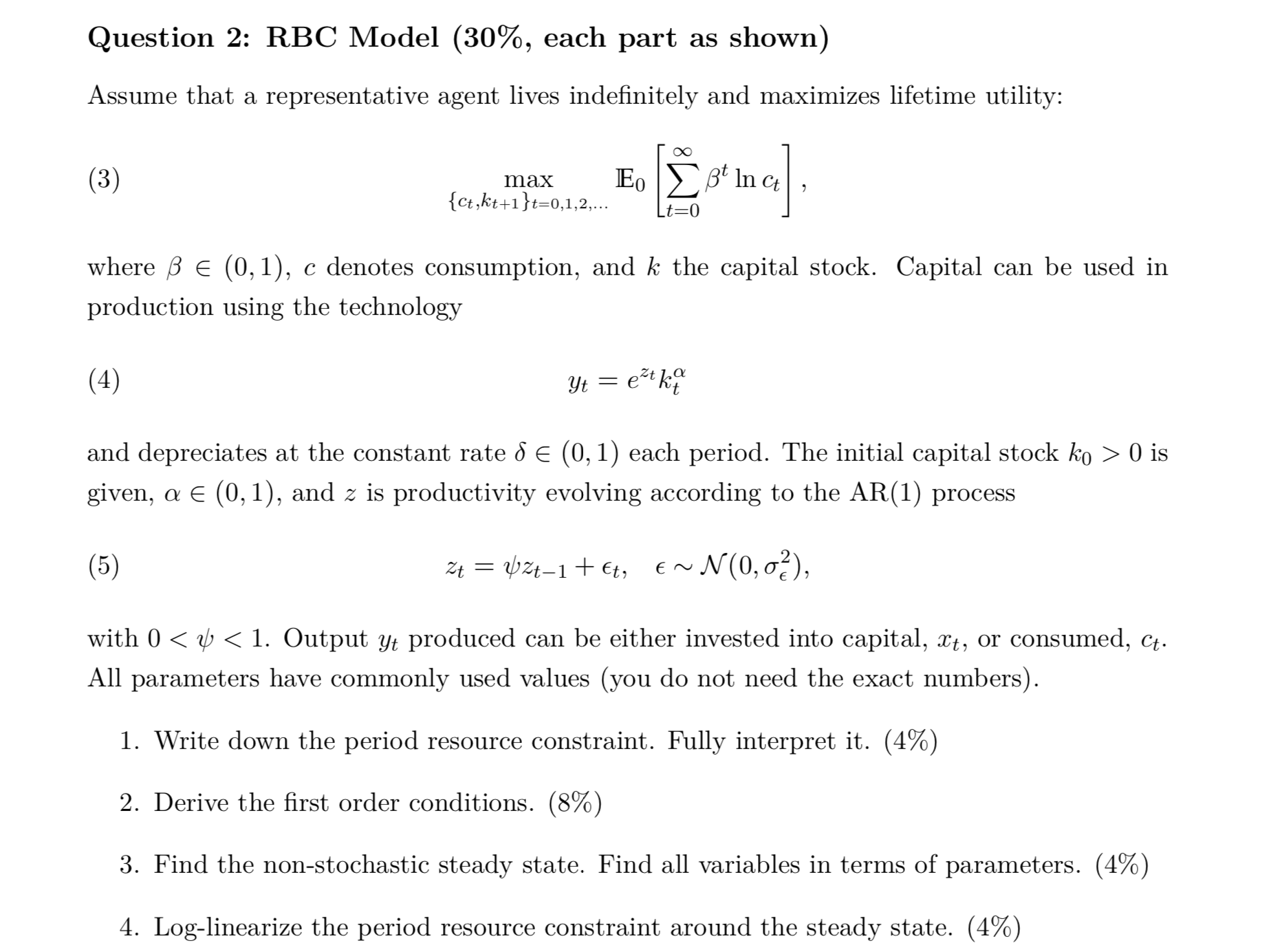Question 2: RBC Model (30%, each part as shown) Assume that a representative agent lives indenitely and maximizes lifetime utility: (3) max IE0 [i t In at) , t=0 {Ct,kt+1}t:o,1,2,... where 3 E (0, 1), c denotes consumption, and k the capital stock. Capital can be used in production using the technology (4) M = 62%? and depreciates at the constant rate 6 E (0, 1) each period. The initial capital stock k0 > 0 is given, a E (0, 1), and z is productivity evolving according to the AR(1) process (5) 2;: = 1/1214 + 6t, 6 N N(0,0'E2 , with 0