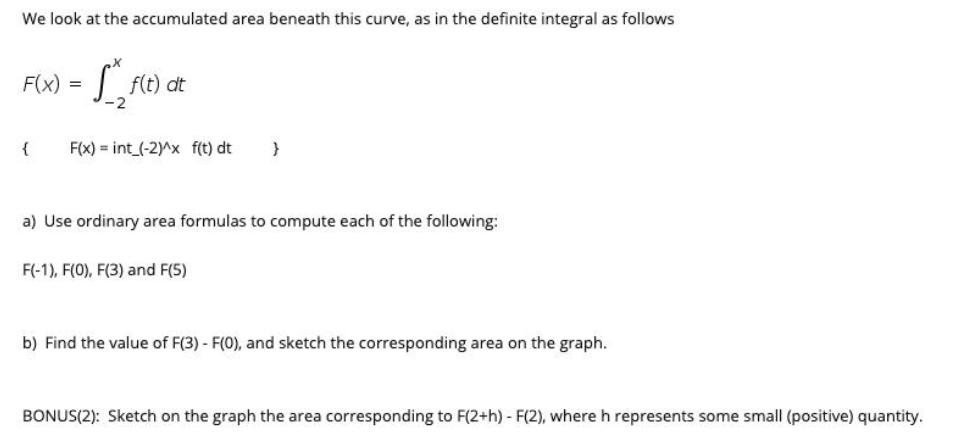
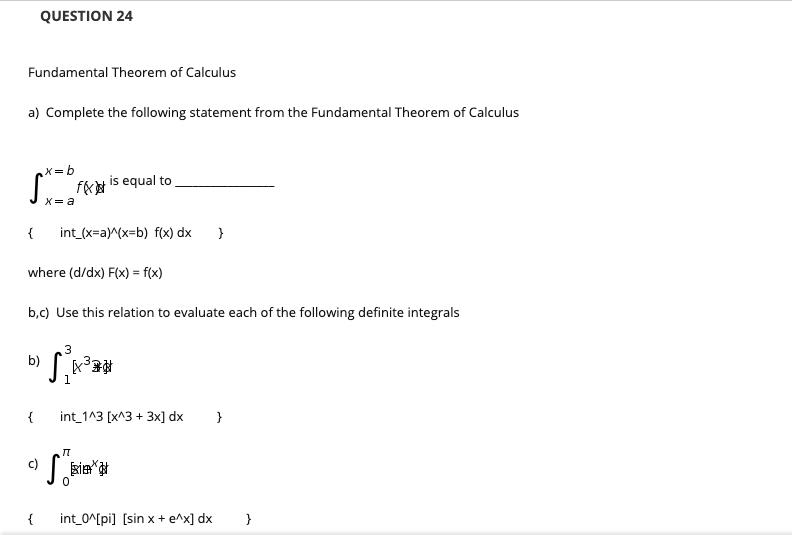
We look at the accumulated area beneath this curve, as in the definite integral as follows F(x) = f(t) dt -2 { F(x) = int_(-2)^x f(t) dt a) Use ordinary area formulas to compute each of the following: F(-1), F(0), F(3) and F(5) b) Find the value of F(3) - F(0), and sketch the corresponding area on the graph. BONUS(2): Sketch on the graph the area corresponding to F(2+h) - F(2), where h represents some small (positive) quantity. QUESTION 24 Fundamental Theorem of Calculus a) Complete the following statement from the Fundamental Theorem of Calculus I fK is equal to X= a { int_(x=a)^(x=b) f(x) dx where (d/dx) F(x) = f(x) %3D b,c) Use this relation to evaluate each of the following definite integrals Sa b) 1 { int_1^3 [x^3 + 3x] dx } c) { int_0^[pi] [sin x + e^x] dx }