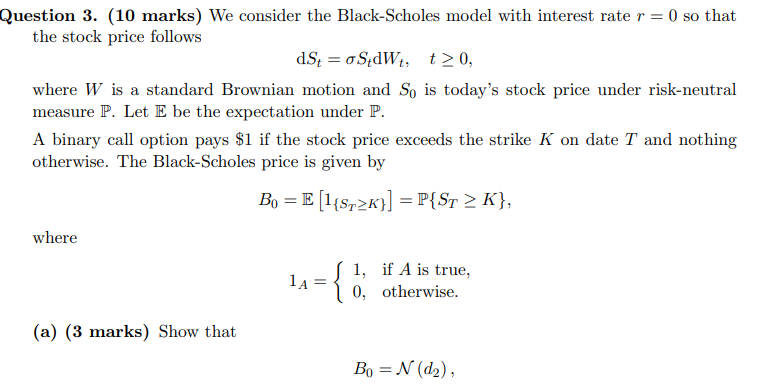
Question 3. (10 marks) We consider the Black-Scholes model with interest rate r=0 so that the stock price follows dSt=StdWt,t0 where W is a standard Brownian motion and S0 is today's stock price under risk-neutral measure P. Let E be the expectation under P. A binary call option pays $1 if the stock price exceeds the strike K on date T and nothing otherwise. The Black-Scholes price is given by B0=E[1{STK}]=P{STK} where 1A={1,0,ifAistrueotherwise. (a) (3 marks) Show that B0=N(d2), where N() is the cumulative normal distribution function and d2:=Tlog(S0/K)212T. (b) (1 mark) A binary log call option pays $1 if logSTlogK and nothing otherwise. Explain why this contract has the same price as the binary call option. (c) (1 mark) Let C0 denote the Black-Scholes price of a regular European call option with strike K. Its payoff is h(ST)=(STK)+,ST>0. Fixing ST, what is the derivative of h with respect to K for KST ? (You can ignore the case when K=ST ). (d) (1 mark) Explain why B0(K)=dKdC0 where B0(K) is the price of a binary with strike K, i.e., B0(K)=N(d2(K)) where d2(K):=Tlog(S0/K)212T. For this question, you are allowed to interchange derivatives over expectations, i.e., dKdE[]=E[dd] (note: it is not necessarily allowed in general). (e) (4 marks) Inverting this relationship and by (a), we can write C0=KB0(x)dx=KN(d2(x))dx. This says that an European call option can be written as an infinite number of binary options with all strikes from K upwards. By integration by parts, C0=KN(d2(x))dx=(i)[xN(d2(x))]K(it)KxdxdN(d2(x))dx. (You do not need to show above). The above should match the Black-Scholes call option price (when r=0 ) C0=S0N(d1(K))KN(d2(K)), where d1(K)=Tlog(S0/K)+212T. (A) (2 marks) Show that limxxN(d2(x))=0 and conclude that (i)=KN(d2(K)) (Hint: Use l'Hpital's rule). (B) (2 marks) Show (ii) =S0N(d1). (Hint: Set y=d2(x) and use change of variables.)