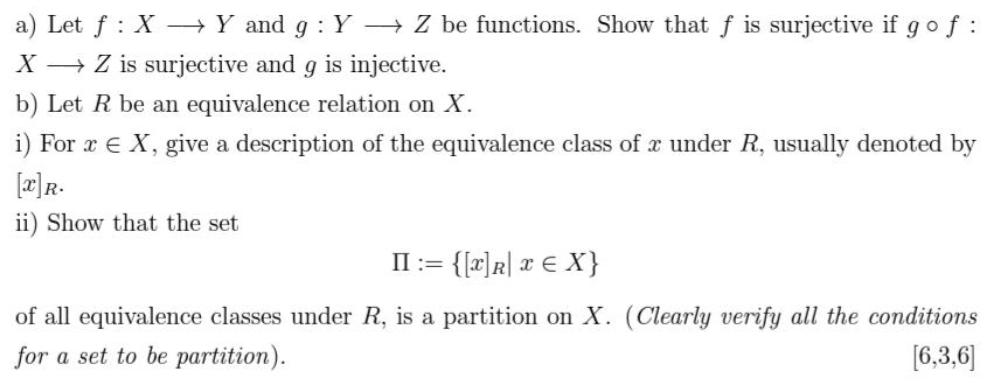
a) Let f : X Y and g : Y Z be functions. Show that f is surjective if go f: X Z is surjective and g is injective. b) Let R be an equivalence relation on X. i) For x E X, give a description of the equivalence class of r under R, usually denoted by []R ii) Show that the set II := {{c]R| x X} of all equivalence classes under R, is a partition on X. (Clearly verify all the conditions for a set to be partition). [6,3,6]