Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Question 3: Explain how to find the limits of integration in spherical coordinates in the order d? d? d?. Notice that just like how we



Question 3: Explain how to find the limits of integration in spherical coordinates in the order d? d? d?. Notice that just like how we have an extra factor of r when integrating in polar or cylindrical coordinates, we have an extra factor of ? 2 sin(?). Note: This font uses the cursive phi ?, which is written as ? in the textbook. These are the same letter. This is for similar, though more complicated, reasons that we had with polar coordinates.
Hint 1: Read the subsection "How to Integrate in Spherical Coordinates" (p. 947 - 949).
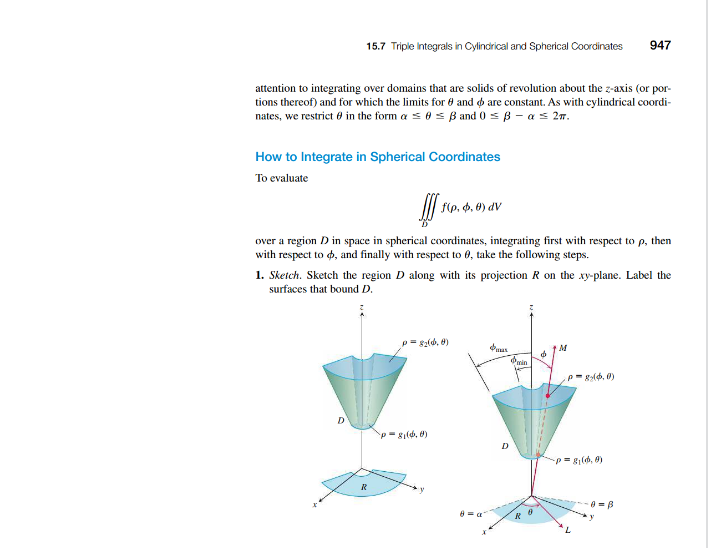
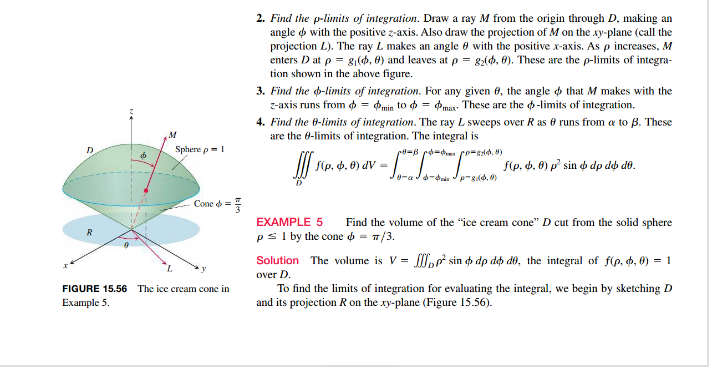

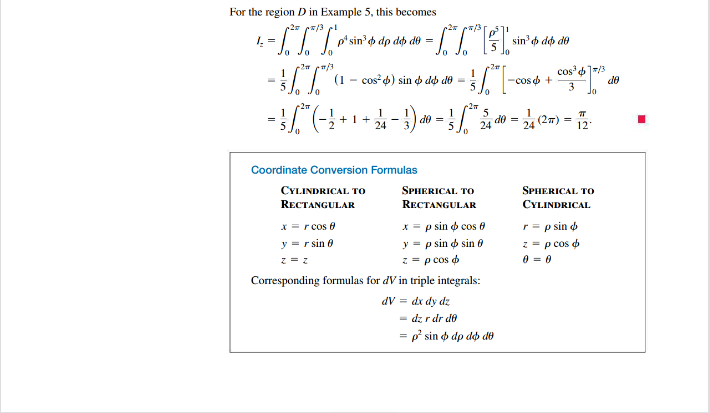

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


