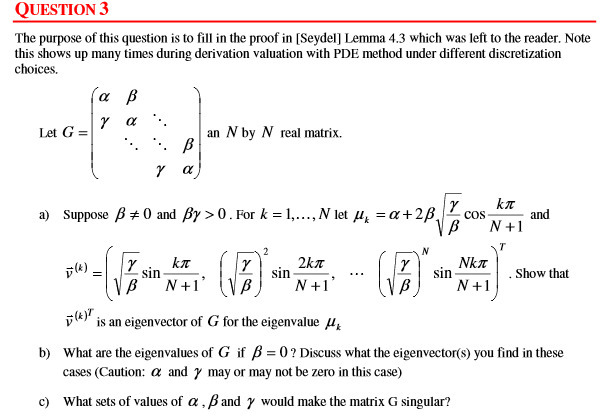
QUESTION 3 The purpose of this question is to fill in the proof in [Seydel] Lemma 4.3 which was left to the reader. Note this shows up many times during derivation valuation with PDE method under different discretization choices. Let G = an N by N real matrix. a) Suppose #0 and By >0. For k = 1,..., N let = a +2//c COS N+1 and 2 Di(k) sin 2 sin B N+1' B N+1 N sin Show that N+1 (k)T is an eigenvector of G for the eigenvalue b) What are the eigenvalues of G if =0? Discuss what the eigenvector(s) you find in these cases (Caution: a and 7 may or may not be zero in this case) c) What sets of values of a, and y would make the matrix G singular?