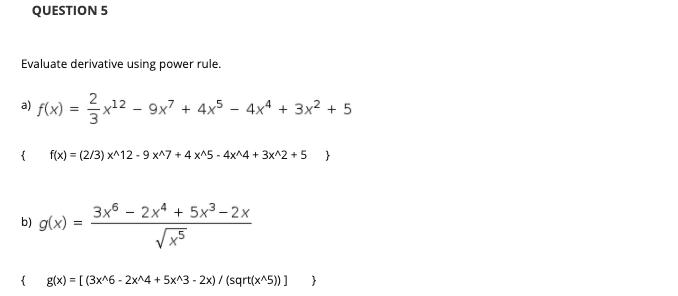
Infinite limit -- cusp or vertical tangent a) Consider the function f(x) defined as follows f(x) = x { fx) = cuberoot(x) } Find the derivative f(x) by the power rule and show f(x) is not defined at x=0. [Note that this case where lim f(x) = +0 and lim f(x) = + o0 x+o+ x+0- corresponds to a vertical tangent line.] b) Consider the function g(x) defined as follows { gx) = cuberoot(x^2) Find the derivative g'(x) by the power rule and show g'(x) is not defined at x-0. [Note that this case where lim g'(x) = +0 and lim g'(x) = x+ o+ - 00 x+0- corresponds to a cusp (which also has infinite slope--and a change in sign).] QUESTION 5 Evaluate derivative using power rule. a) f(x) = x12 - 9x7 + 4x5 - 4x4 + 3x? + 5 { f(x) = (2/3) x^12 -9 x^7 + 4 x^5 - 4x^4 + 3x^2 + 5 } 3x6 - 2x + 5x-2x b) g(x) = { g(x) = [ (3x^6 - 2x^4 + 5x^3 - 2x) / (sqrt(x^5)) ] QUESTION 6 Equation of tangent line. Consider the following function f(x) f(x) = 2x3 - 3x? - 2 { f(x) = 2x^3 - 3x^2 - 2 } %3D a) Evaluate f(3), the derivative of the function f(x) at x=3. b) Find equation of tangent line to the graph of f(x) at the point (3, f(3).