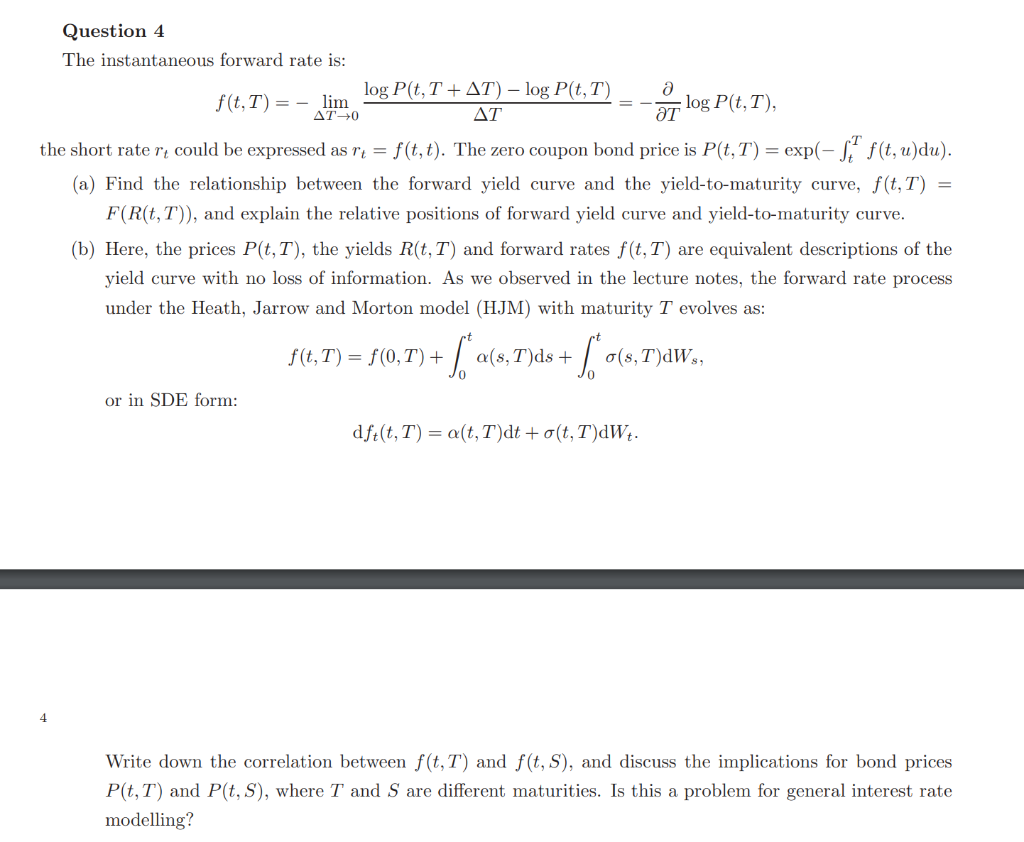
Question 4 The instantaneous forward rate is: a f(t, T) lim T-0 log P(t, T+AT) - log P(t, T) AT log P(t, T), T the short rate rt could be expressed as r = f(t, t). The zero coupon bond price is P(t, T) = exp(- f f(t, u)du). (a) Find the relationship between the forward yield curve and the yield-to-maturity curve, f(t,T) = F(R(t, T)), and explain the relative positions of forward yield curve and yield-to-maturity curve. (b) Here, the prices P(t, T), the yields R(t, T) and forward rates f(t, T) are equivalent descriptions of the yield curve with no loss of information. As we observed in the lecture notes, the forward rate process under the Heath, Jarrow and Morton model (HJM) with maturity T evolves as: f(t, T) = f(0, T) + S a(s, T)ds + -S o(s, T)dWs, or in SDE form: dft(t, T) = a(t, T)dt + o(t, T)dWt. 4 Write down the correlation between f(t, T) and f(t, S), and discuss the implications for bond prices P(t, T) and P(t, S), where T and S are different maturities. Is this a problem for general interest rate modelling? Question 4 The instantaneous forward rate is: a f(t, T) lim T-0 log P(t, T+AT) - log P(t, T) AT log P(t, T), T the short rate rt could be expressed as r = f(t, t). The zero coupon bond price is P(t, T) = exp(- f f(t, u)du). (a) Find the relationship between the forward yield curve and the yield-to-maturity curve, f(t,T) = F(R(t, T)), and explain the relative positions of forward yield curve and yield-to-maturity curve. (b) Here, the prices P(t, T), the yields R(t, T) and forward rates f(t, T) are equivalent descriptions of the yield curve with no loss of information. As we observed in the lecture notes, the forward rate process under the Heath, Jarrow and Morton model (HJM) with maturity T evolves as: f(t, T) = f(0, T) + S a(s, T)ds + -S o(s, T)dWs, or in SDE form: dft(t, T) = a(t, T)dt + o(t, T)dWt. 4 Write down the correlation between f(t, T) and f(t, S), and discuss the implications for bond prices P(t, T) and P(t, S), where T and S are different maturities. Is this a problem for general interest rate modelling