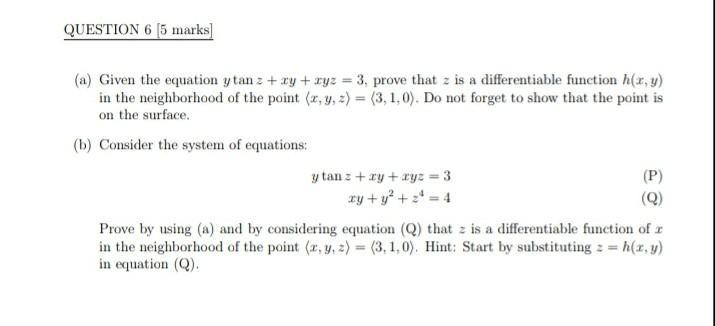
QUESTION 6 [5 marks] (a) Given the equation ytanz + xy + xyz = 3, prove that z is a differentiable function h(x, y) in the neighborhood of the point (x, y, z)=(3, 1.0). Do not forget to show that the point is on the surface. (b) Consider the system of equations: y tanz + xy + xyz = 3 xy + y +2=4 (P) (Q) 2 Prove by using (a) and by considering equation (Q) that z is a differentiable function of in the neighborhood of the point (x, y, z)=(3,1,0). Hint: Start by substituting z=h(x,y) in equation (Q).