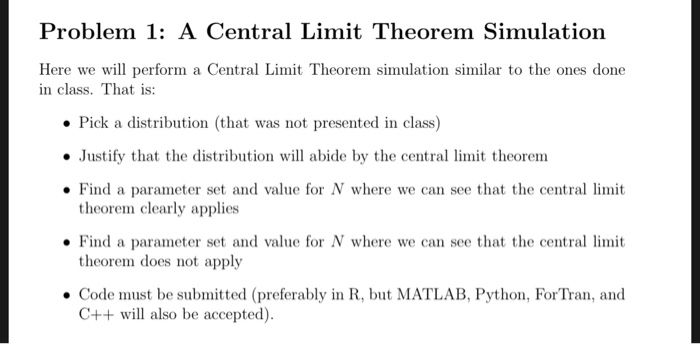
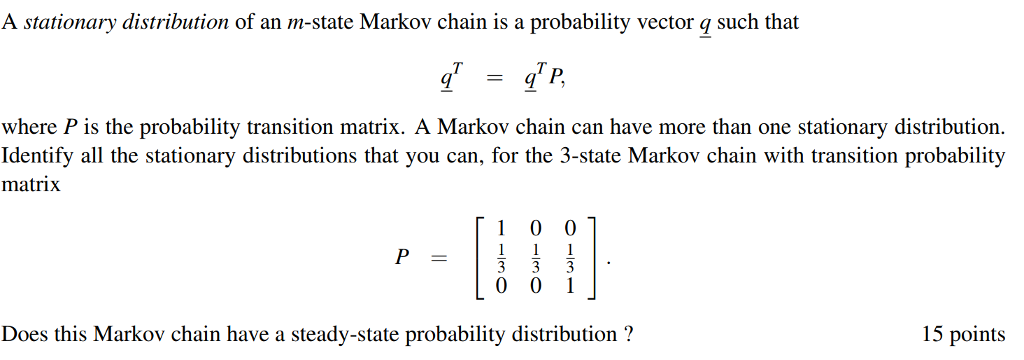
Question:

Airlines constantly monitor the "booking curve" for each flight of their network. Simply put, a booking curve is the time-phased trajectory of the bookings. From one day to the next, bookings follow the following simple relationship:

??+1 = (?? + ??+1) ? (1 ? ?)

where,

??= Total bookings at the end of day i

?? = Incremental bookings that come in on day i

? = Cancellation Rate

For a certain flight an airline operates a plane with 200 seats. Currently it has a total of 160 bookings (?0). It estimates the daily (incremental) bookings to follow a normal distribution with mean = 8 and std deviation = 2. It also estimates its daily cancellation rate to be 4%

(i) What will the total bookings be at the end of ten days?

(ii) The airline has a stated goal of keeping the probability of denied boarding to be no more than 1%. Will this flight satisfy that corporate objective? [Assume there are no transactions (bookings or cancellations) after the end of ten days.]

[Run 1000 iterations. Save results so that grader can check values from your runs]

Which of the following statements are TRUE regarding Central Limit Theorem? CCS The central limit theorem gives the exact probability of estimating the true population mean. Chart / Roll The central limit theorem only applies when the population distribution is normal. The range of values for the sampling distribution of means is larger than the range of values in the population. The central limit theorem requires that all samples are randomly selected from a single population.Question 17 4 pts Which of the following statements is NOT consistent with the Central Limit Theorem? O The Central Limit Theorem indicates that the mean of the sampling distribution will be equal to the population mean. O The Central Limit Theorem indicates that the sampling distribution will be approximately normal when the sample size is sufficiently large. O The Central Limit Theorem applies to non-normal distributions. O The Central Limit Theorem applies without regard to the size of the sample.Problem 1: A Central Limit Theorem Simulation Here we will perform a Central Limit Theorem simulation similar to the ones done in class. That is: . Pick a distribution (that was not presented in class) . Justify that the distribution will abide by the central limit theorem . Find a parameter set and value for / where we can see that the central limit theorem clearly applies . Find a parameter set and value for / where we can see that the central limit theorem does not apply . Code must be submitted (preferably in R, but MATLAB, Python, ForTran, and C++ will also be accepted).A stationary distribution of an m-state Markov chain is a probability vector q such that = q P, where P is the probability transition matrix. A Markov chain can have more than one stationary distribution. Identify all the stationary distributions that you can, for the 3-state Markov chain with transition probability matrix O O P Owl Does this Markov chain have a steady-state probability distribution ? 15 points