Question:

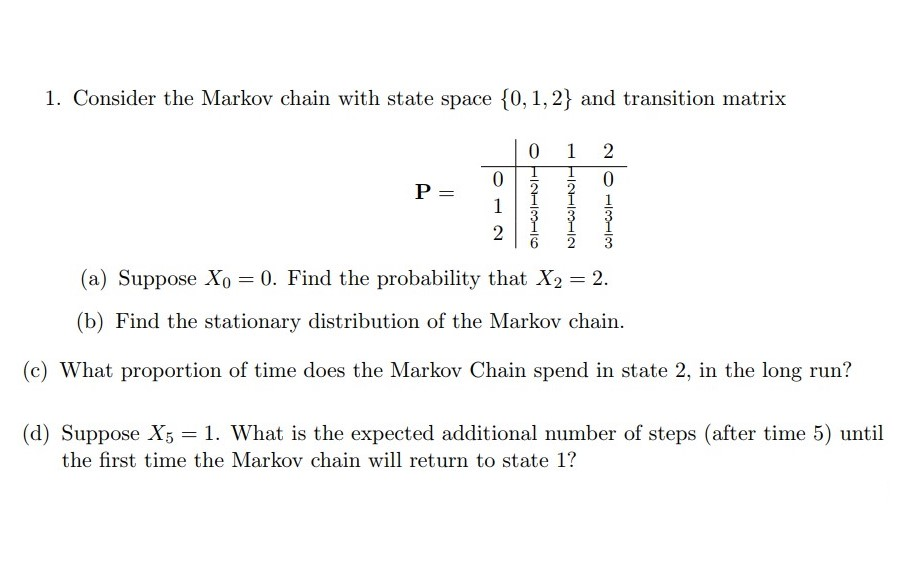
Buses arrive at a bus station with i.i.d. interarrival times following an exponential distribution with intensity ?. Alice arrives at the bus station at a deterministic time t. a. (4pts) What is the expected waiting time for Alice until next bus comes? b. (6pts) Let ? be the time when the last bus arrived before time t. Show that t ? ? follows an exponential distribution with parameter ?. c. (6pts) Show that the expected interarrival time between the last bus which arrived before time t and the first bus which arrives after time t is 2 ? . Explain why it is different from the general expected interarrival time 1 ? .

Question 20 1 pts Let P be the transition matrix of a Markov chain with n states. Which one of the following statements is not always true? If Q is another transition matrix of a Markov chain with n states, then =(P + Q) is the transition matrix of a Markov chain with n states. O P2 is the transition matrix of a Markov chain with n states. If P is invertible, then p-1 is the transition matrix of a Markov chain with n states. If Q is another transition matrix of a Markov chain with n states, then PQ is the transition matrix of a Markov chain with n states.1. Consider the Markov chain with state space {0, 1, 2} and transition matrix 1 2 P = HO 2 (a) Suppose Xo = 0. Find the probability that X2 = 2. (b) Find the stationary distribution of the Markov chain. (c) What proportion of time does the Markov Chain spend in state 2, in the long run? (d) Suppose X5 = 1. What is the expected additional number of steps (after time 5) until the first time the Markov chain will return to state 1?8. (10 points) (The Weak Law of Large Numbers) In order to estimate f, the true fraction of smokers in a large population, Alvin selects n people at random. His estimator M. is obtained by dividing S,, the number of smokers in his sample, by N, i.e., M. = 5,. Alvin chooses the sample size n to be the smallest possible number for which the Chebyshev inequality yields a guarantee that where e and o are some prespecified tolerances. Determine how the value of n recommended by the Chebyshev inequality changes in the following cases. The best guarantee will be when P(IMn - f1 20)= Ane] (a) (5 points) The value of