Question
Question: (Cauchy dissemination.) An arbitrary variable X with thickness ? co 0 1 a f(x) = 1r a2 ?/7)2 is said to have a Cauchy
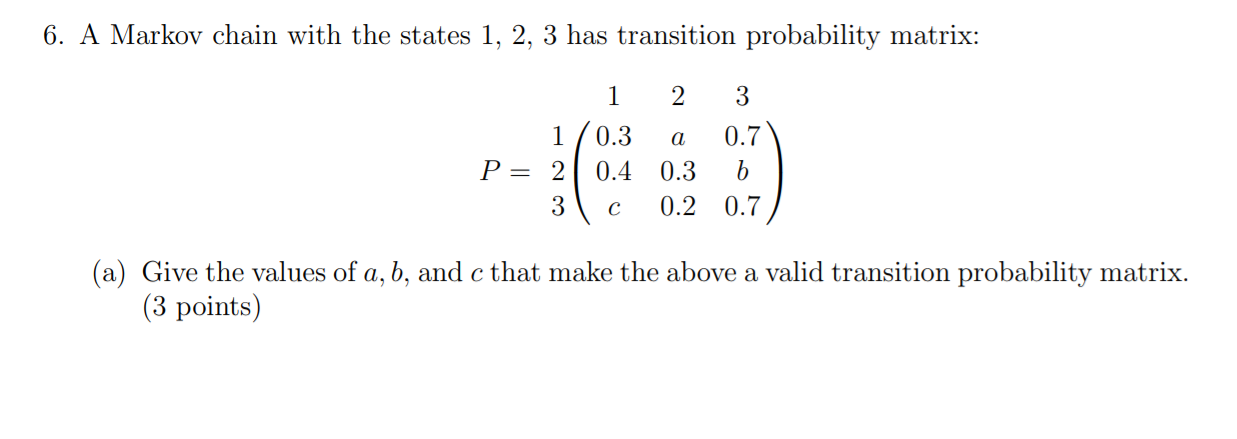



Question:
(Cauchy dissemination.) An arbitrary variable X with thickness ? co 0
1 a f(x) = 1r a2 ?/7)2
is said to have a Cauchy dissemination with boundaries an and b. This dispersion is fascinating in that it gives an illustration of a constant irregular variable whose mean doesn't exist. Let a = 1 and b = 0 to acquire an exceptional instance of the Cauchy conveyance with thickness 1 f(x) = 1 + x= - co
Question 10
Let Xdenote the measure of time in hours that a battery on a sunlight based adding machine will work enough between openings to light adequate to re-energize the battery. Accept that the thickness for X is given by .f(x) = (5016)x-3 2 (b) Discover the articulation for the aggregate circulation work for X, and use it to discover the likelihood that an arbitrarily chosen sunlight based battery will last all things considered 4 hours prior to waiting be re-energized. (c) Figure out the normal time that a battery will last prior to waiting be re-energized. (d) Discover E[X2], and utilize this to discover the fluctuation of X. Question 11 Accept that the increment sought after for electric force in great many kilowatt hours throughout the following 2 years in a specific zone is an irregular variable whose thickness is given by KO= (1/64),0 0 (b) Discover the articulation for the total dispersion for X, and use it to discover the likelihood that the interest will be all things considered 2 million kilowatt hours. (c) If the territory just has the ability to create extra 3 million kilowatt hours, what is the likelihood that request will surpass supply? (d) Track down the normal expansion sought after. Question 12 The mass thickness of soil is characterized as the mass of dry solids per unit mass volume. A high mass thickness suggests a minimized soil with not many pores. Mass thickness is a significant factor in affecting root advancement, seedling development, and air circulation. Allow X to mean the mass thickness of Pima earth soil. Studies show that X is typically dispersed with p = 1.5 and a = .2 g/cm3. (a) What is the thickness for X? Sketch a chart of the thickness work. Show on this chart the likelihood that X lies somewhere in the range of 1.1 and 1.9. Discover this likelihood. (b) Discover the likelihood that a haphazardly chosen test of Pima earth soil will have mass thickness under .9 g/cm3. (c) Would you be shocked if an arbitrarily chosen test of this sort of soil has a mass thickness in overabundance of 2.0 g/cm3? Clarify, in view of the likelihood of this happening. (d) What point has the property that lone 10% of the dirt examples have mass thickness this high or higher? (e) What is the second creating capacity for .X?


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


