Question:

Harwood Medical Instruments PLC

Harwood Medical Instruments PLC (HMI), based just outside of Birmingham, England, manufactured specialty medical instruments and sold them in market niches that were becoming increasingly competitive and price sensitive because of pressures to reduce health care costs. HMI was organized into nine decisions each run by a general manager. Over the years, HMI had grown both organically and by acquisition. Six of the divisions had been acquired by HMI within the past decade.

All of HMI's divisions sold medical products to hospitals, laboratories, and/or doctors, so the need for product quality and reliability was high. The divisions varied significantly, however, in terms of the degree to which their success depended on, for example, development of new products, efficiency of production, and/or customer service.

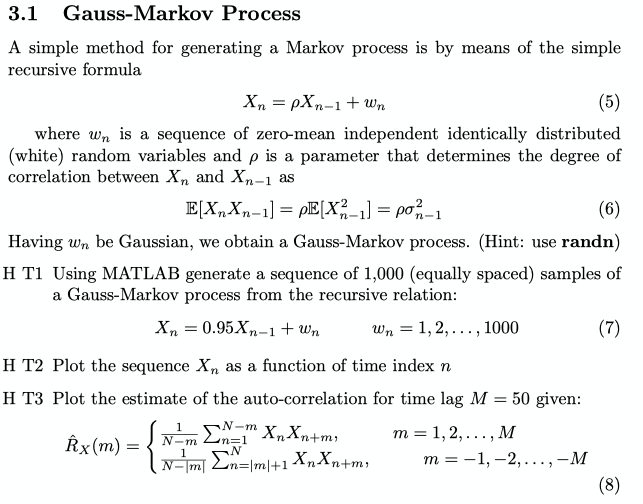
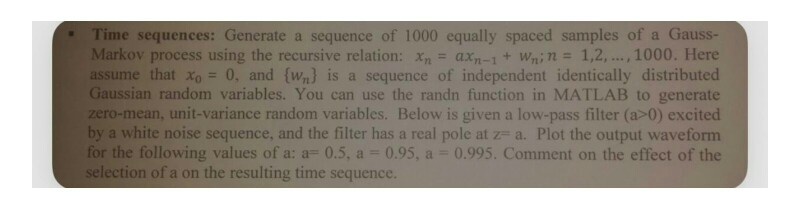
Bonuses for division general managers were paid semi-annually. Up to the year 2009, these bonuses were calculated as 1% of division operating profits.

HMI's managing director, Andy Guthrie, had concerns though that the operating profit measure was too narrowly focused. He had been reading articles about performance measurement and decided to a "more balanced" scorecard. In November 2009, just before introducing a new bonus plan, Mr. Guthrie explained to his chief financial officer that he was willing to pay out higher bonuses than had been paid historically if improved performance warranted doing so.

The new plan provided a base bonus for division general managers of 1% of division operating profits for the half-year period. This base bonus was adjusted as follows:

Increased by 5,000 if over 99% of deliveries were on time; by 2,000 if 95-99% of deliveries were on time; or by zero is less than 95% of deliveries were on time.

Increased by 5,000 if sales returns were less than or equal to1% of sales, or decreased by 50% of the excess of sales returns over 1% of sales.

Increased by 1,000 for every patent application filed with the UK Intellectual Property Office.

Reduced by the excess of scrap and rework costs over 1% of operating profit.

Reduced by 5,000 if average customer satisfaction ratings were below 90%.

If the bonus calculation resulted in a negative amount for a particular period, the manager received no bonus. Negative amounts were not carried forward to the next period.

Exhibit 1 shows results for two representative HMI divisions for the year 2010, the first year under the new bonus plan. The Surgical Instruments Division (SID), one of HMI's original businesses, sold a variety of surgical instruments, including scissors, scapels, retractors, and clamps. The markets for these products were mature, so growth was relatively slow. Not much innovation was needed, but controlling costs was critical. The Ultrasound Diagnostic Equipment Division (Ultrasound), which was acquired in 2007, sold and serviced ultrasound probes, transducers, and diagnostic imaging systems. The ultrasound market promised excellent growth and profits if the division could keep its sophisticated products on the cutting edge technologically and control both product development and product costs effectively.

In 2009, the total annual bonuses for the year earned by the managers of SID and Ultrasound were approximately 85,000 and 74,000, respectively.

Exhibit 1 Harwood Medical Instruments PLC

Operating results for the surgical Instruments and Ultrasound Diagnostic Equipment Divisions, 2010 ( in 000s)

Surgical Instruments Division

Ultrasound Diagnostic Equipment Division

1st half of 2010

2nd half of 2010

1st half of 2010

2nd half of 2010

Sales

42,000

44,000

28,600

29,000

Operating profit

4,620

4,400

3,420

4,060

On-time deliveries

95.4%

97.3%

98.2%

94.6%

Sales returns

450

420

291

289

Patent applications filed

0

1

4

8

Scrap and rework costs

51.1

45.0

39.7

28.2

Customer satisfaction (average)

78%

89%

81%

91%

1. Critically analyze the current reward system that Harwood Medical Instruments PLC uses to award the bonus.

2. Calculate the bonus earned by each manager of the two Divisions for each 6-month period and for the year 2010 (i.e. total bonus) [Note: the figures are in thousands; ('000s)].

3. (i) Design a performance measurement reward system that would encapsulate a rather "more balanced" approach in awarding of bonuses.

(ii) Briefly explain how the reward system you design in 3(i) can be used to award bonus.

1.76. Consider a branching process as defined in Example 1.8, in which each family has exactly three children, but invert Galton and Watson's original mo- tivation and ignore male children. In this model a mother will have an average of 1.5 daughters. Compute the probability that a given woman's descendents will die out. Example 1.8. Branching processes. These processes arose from Francis Galton's statistical investigation of the extinction of family names. Consider a population in which each individual in the nth generation independently gives birth, producing k children (who are members of generation n + 1) with proba- bility px. In Galton's application only male children count since only they carry on the family name. To define the Markov chain, note that the number of individuals in genera- tion n, X,, can be any nonnegative integer, so the state space is {0, 1, 2, .. . ). If we let Y1, Y2. .. . be independent random variables with P(Ym = k) = p, then we can write the transition probability as p(i, j) = P(Yi + . . . + Mi=j) for i > 0 and j 2 0 When there are no living members of the population, no new ones can be born, so p(0, 0) = 1. Galton's question, originally posed in the Educational Times of 1873, is Q. What is the probability that the line of a man becomes extinct?, i.e., the branching process becomes absorbed at 0? Reverend Henry William Watson replied with a solution. Together, they then wrote an 1874 paper entitled On the probability of extinction of families. For this reason, these chains are often called Galton-Watson processes.2. Let {X : n 2 0} be a Galton-Watson branching process with Xo = 1, and suppose that the family size distribution has generating function G(s) = 1 - a(1 -$)1/2 where 0 0) excited by a white noise sequence, and the filter has a real pole at z- a. Plot the output waveform for the following values of a: a= 0.5, a = 0.95, a = 0.995. Comment on the effect of the selection of a on the resulting time sequence