Question
Question: use ibm as400 series to answer the following question (the output should shown in screenshot) 1 - develop a new Library called MnnLIB1 (NN
Question:
use ibm as400 series to answer the following question (the output should shown in screenshot)
1 - develop a new Library called MnnLIB1 (NN is your student login id number) using the command CRTLIB
2 - develop a new Jobq MnnJOBQ1 and Outq MnnOUTQ1 in the Library MnnLIB1 using the command crtjobq and crtoutq
3 - Grant *PUBLIC *USE Authority to your MnnLIB1 library using the command wrkobj MnnLIB1 and then option 2
4 - Submit 3 jobs using sbmjob in your MnnJOBQ1, these jobs will not run, since they are not attached to any sub-system. Check it out by using wrkjobq command
5 - Change the 3 jobs submitted in step #4 to run in sub-system QINTER and change the out to MnnOUTQ1. These jobs will run, and the results will be in MnnOUTQ1. Check it out by using wrkoutq command
6 - develop a new Source File called MnnSRC in your library MnnLIB1 using command crtsrcpf
7 - develop a new Save File called MnnSAVF in your library MnnLIB1 using command crtsavf
8 - Run DSPJOBLOG command and save the output in your spool file
9 - develop the menu command steps to navigate to the command DSPLIB from GO MAIN menu
10 - develop the menu command steps to navigate to the command SBMJOB from GO MAIN menu

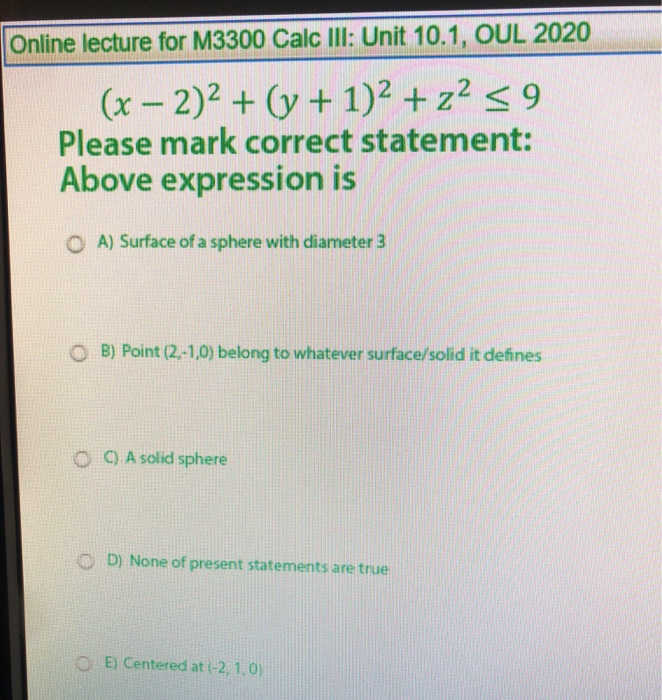

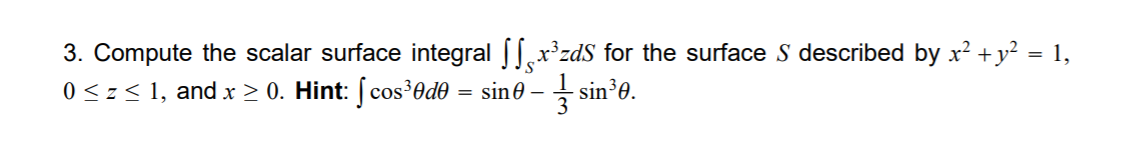
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


