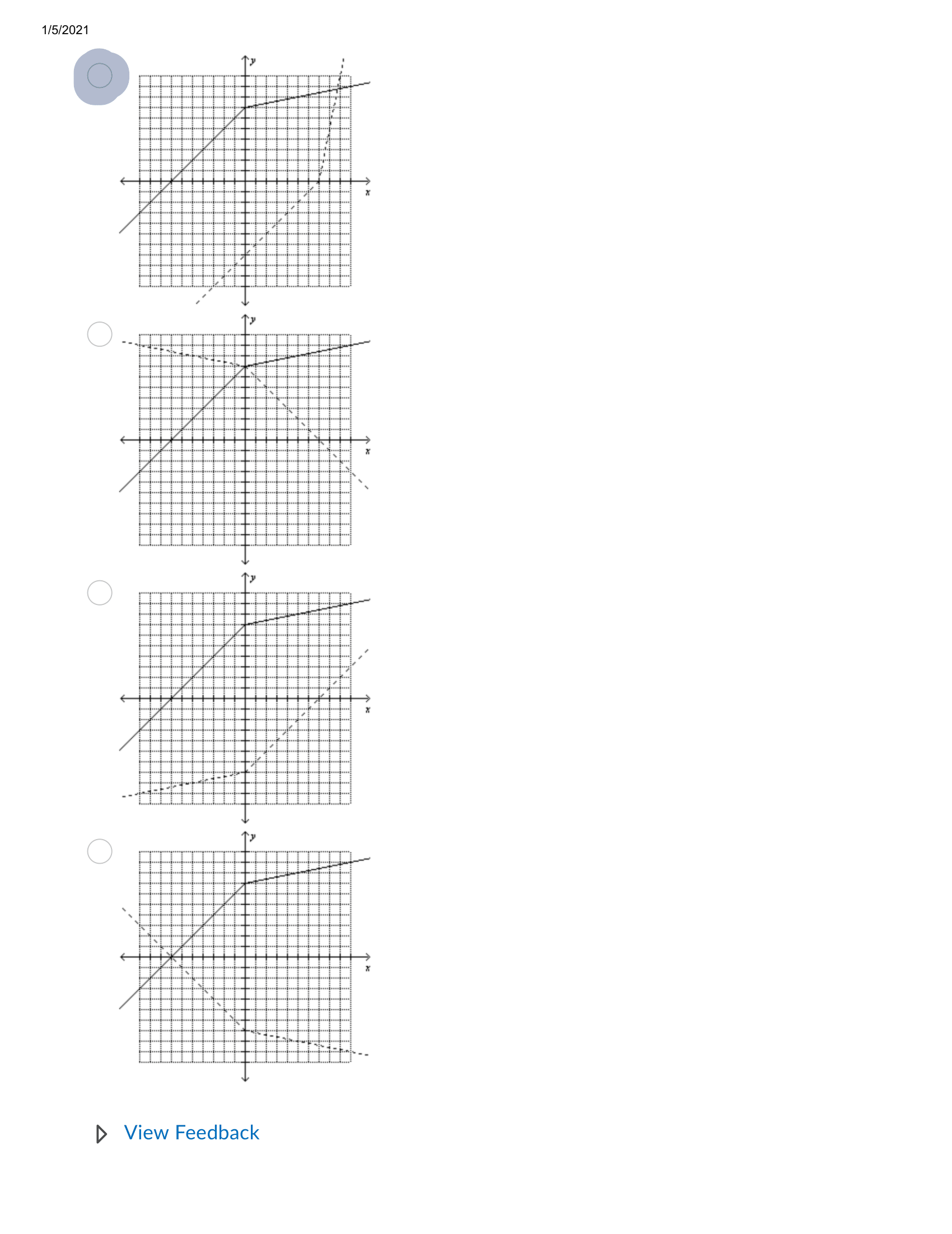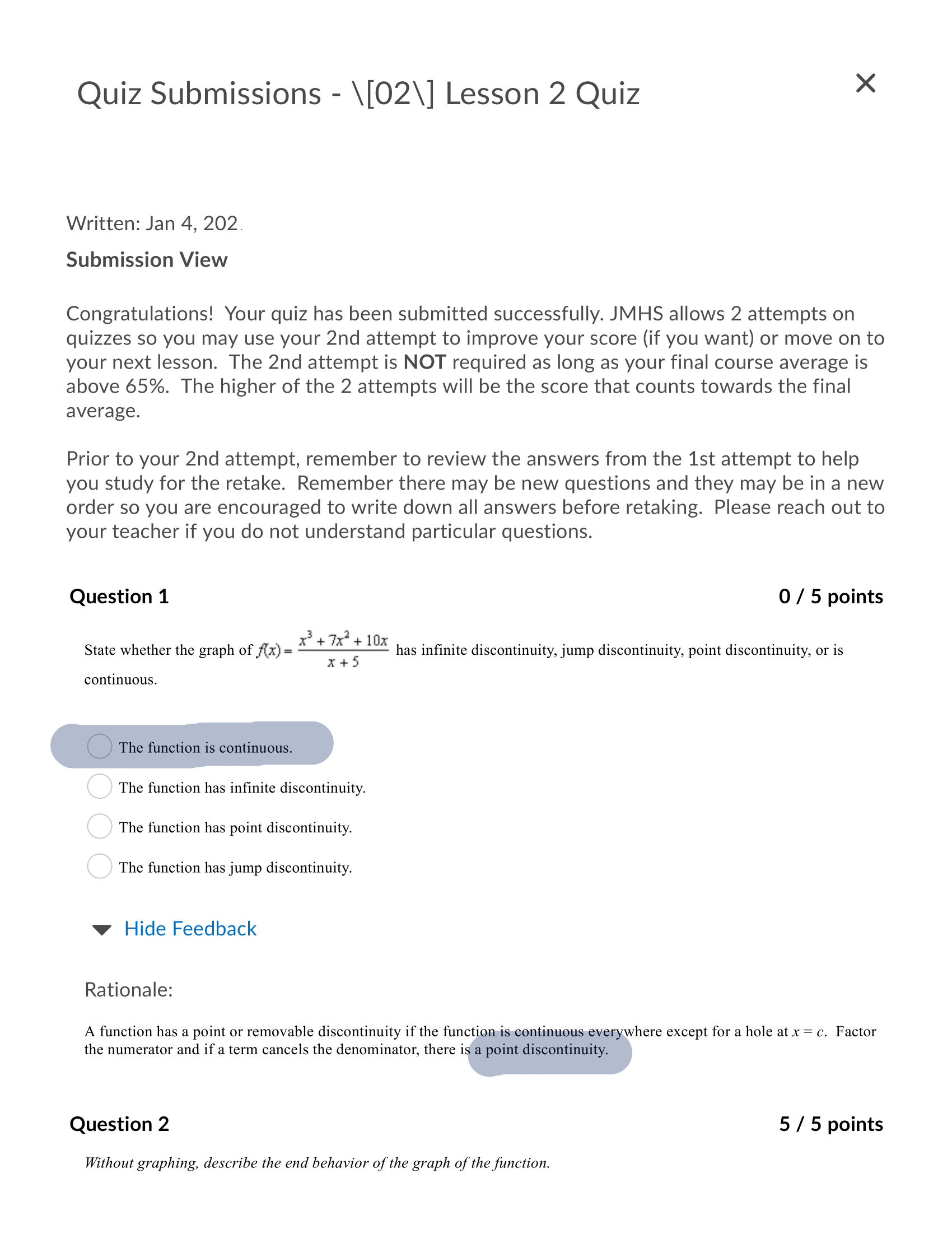
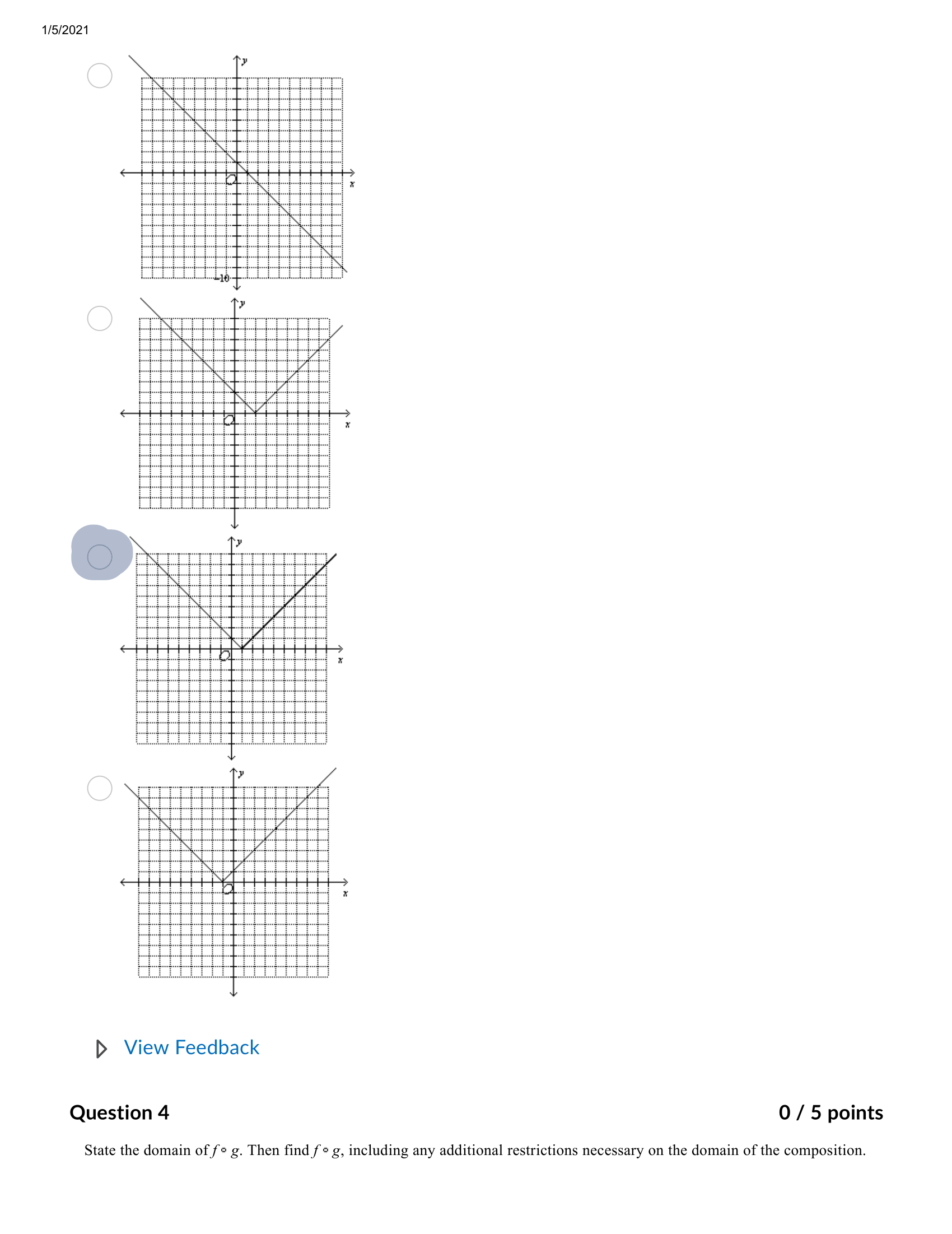
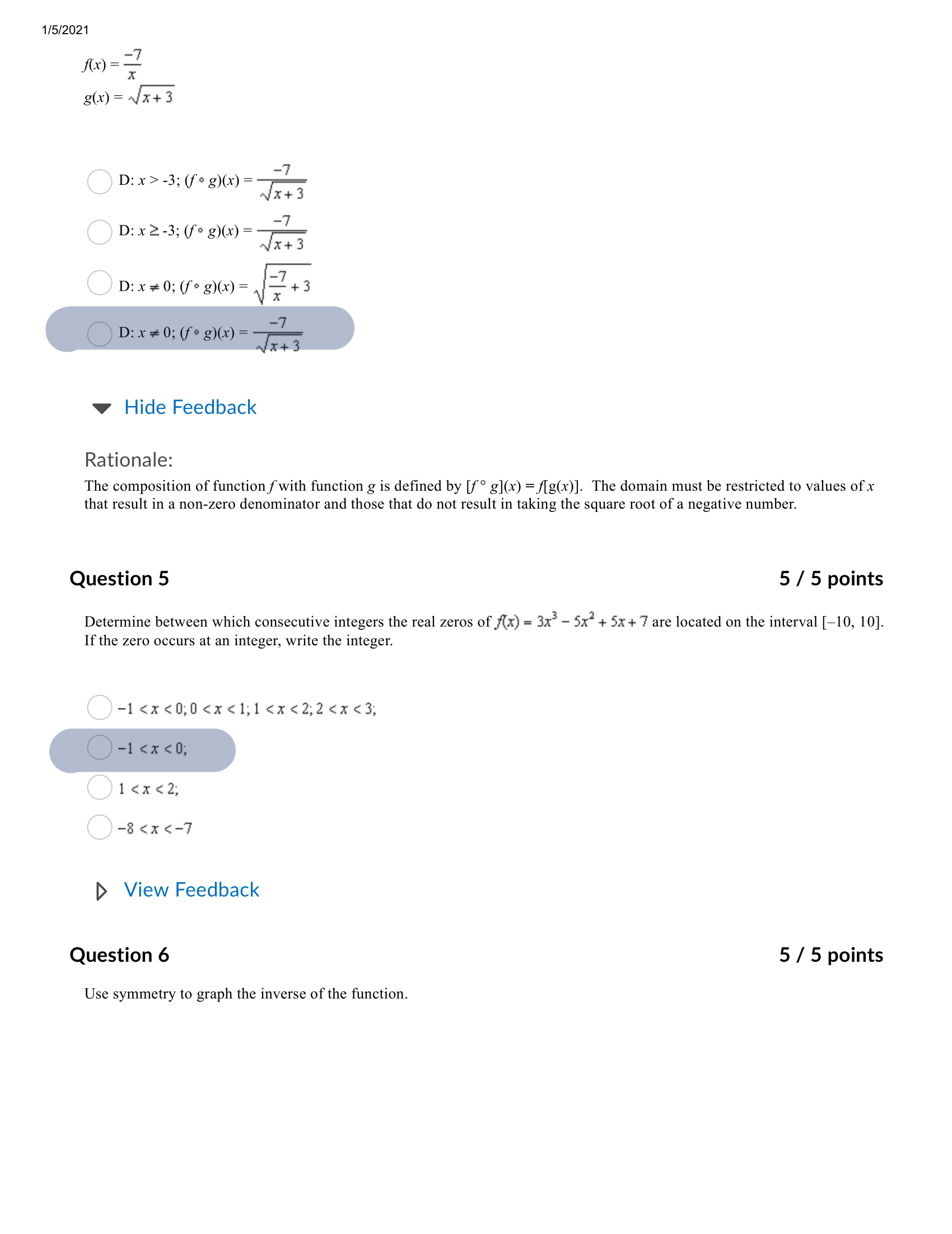
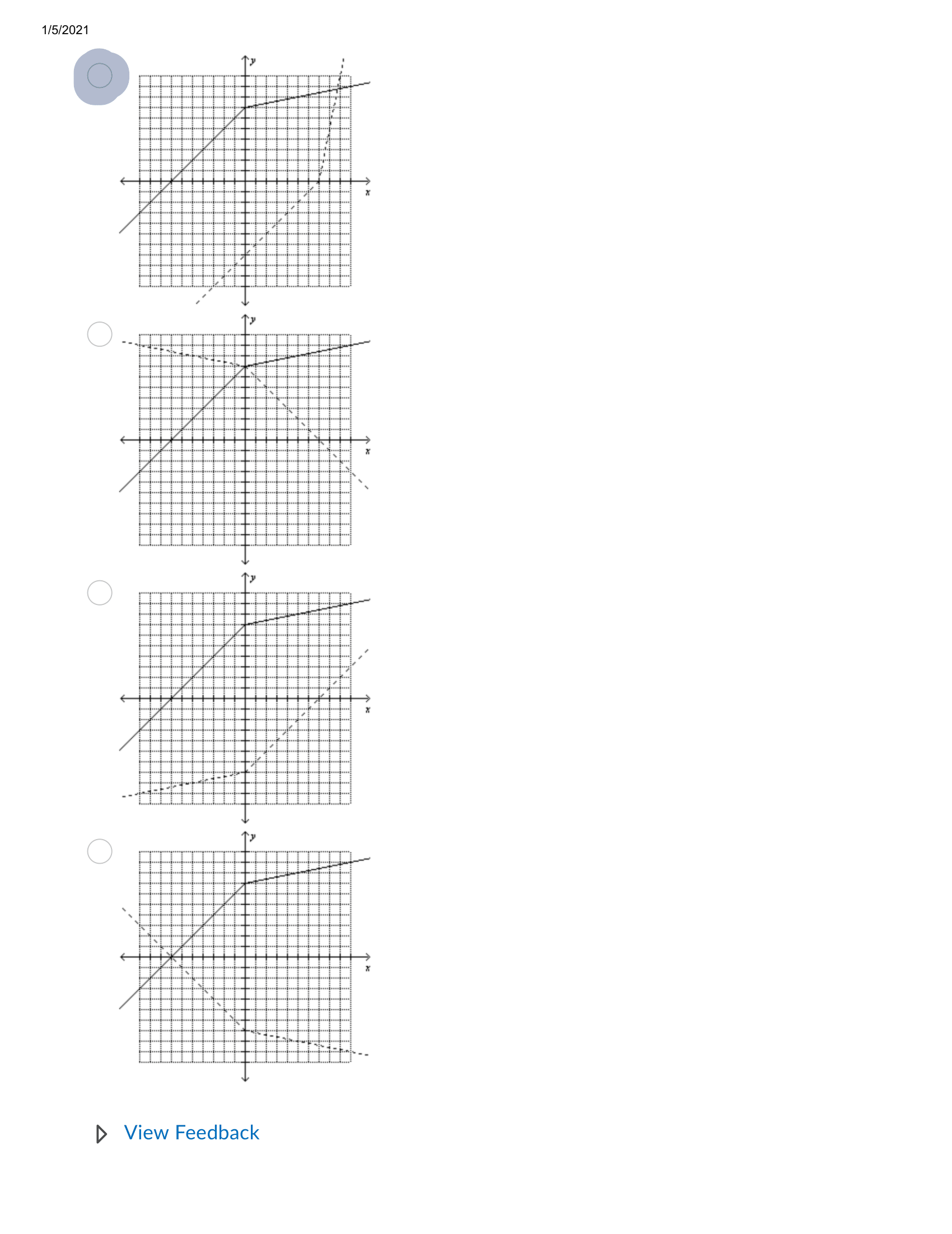
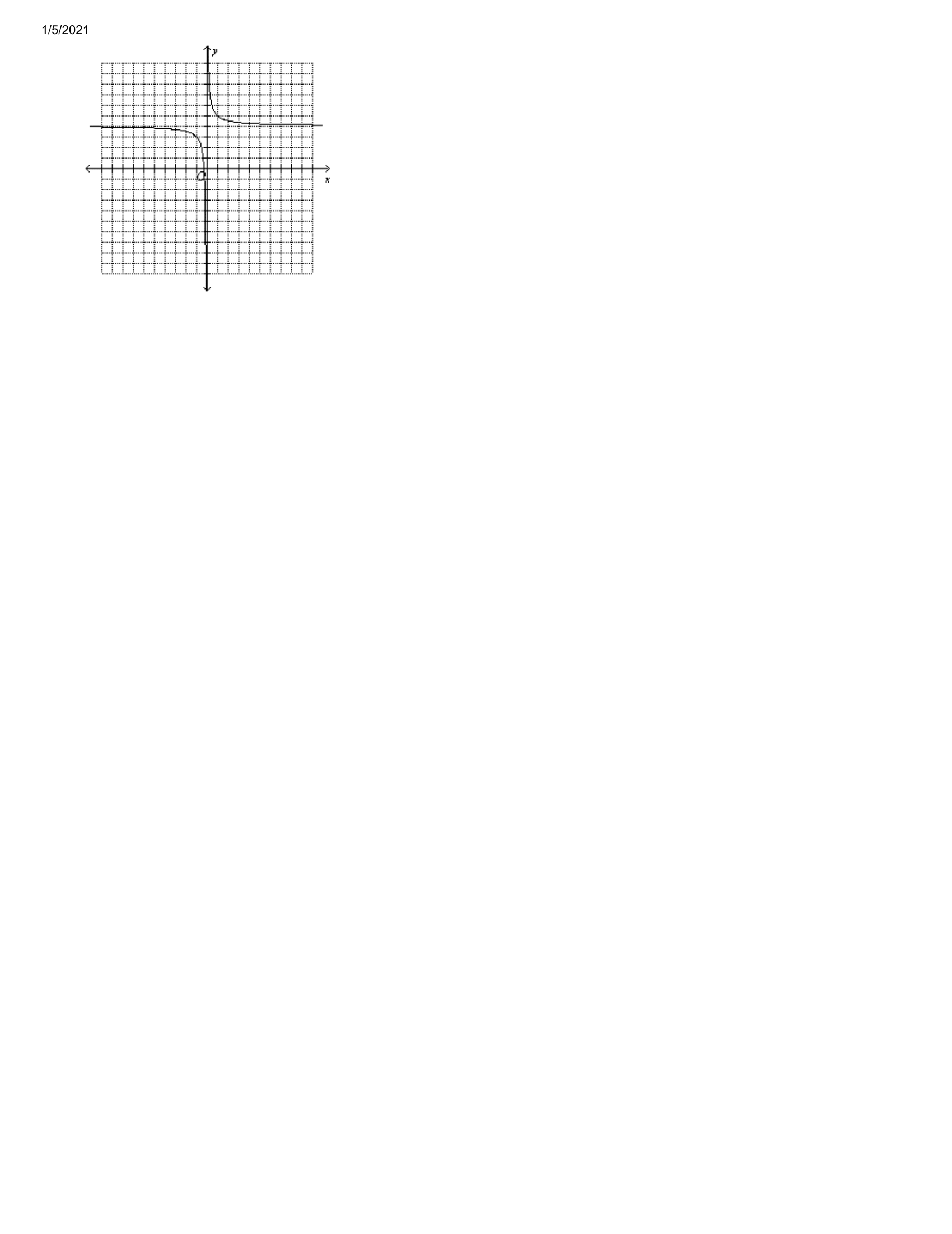
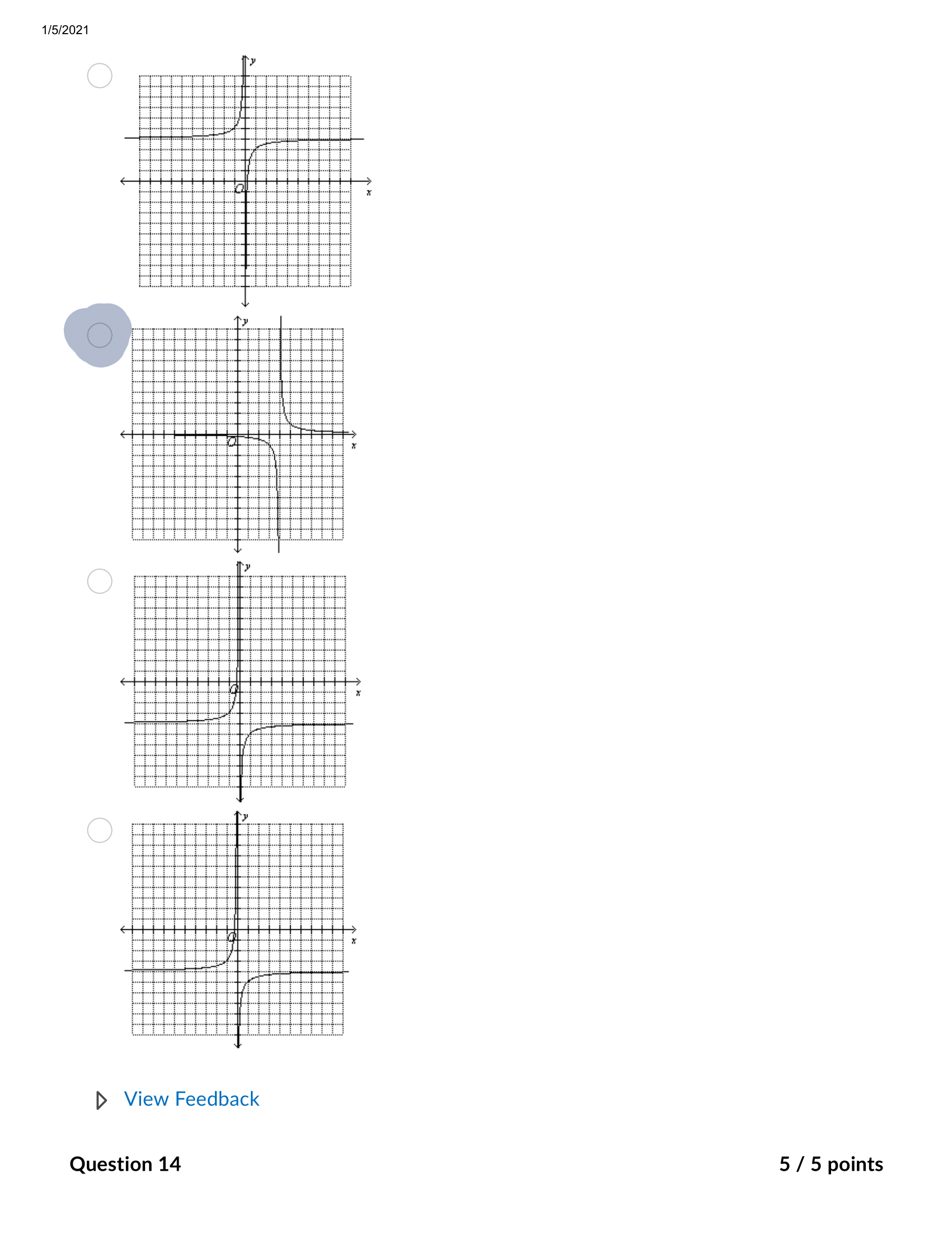
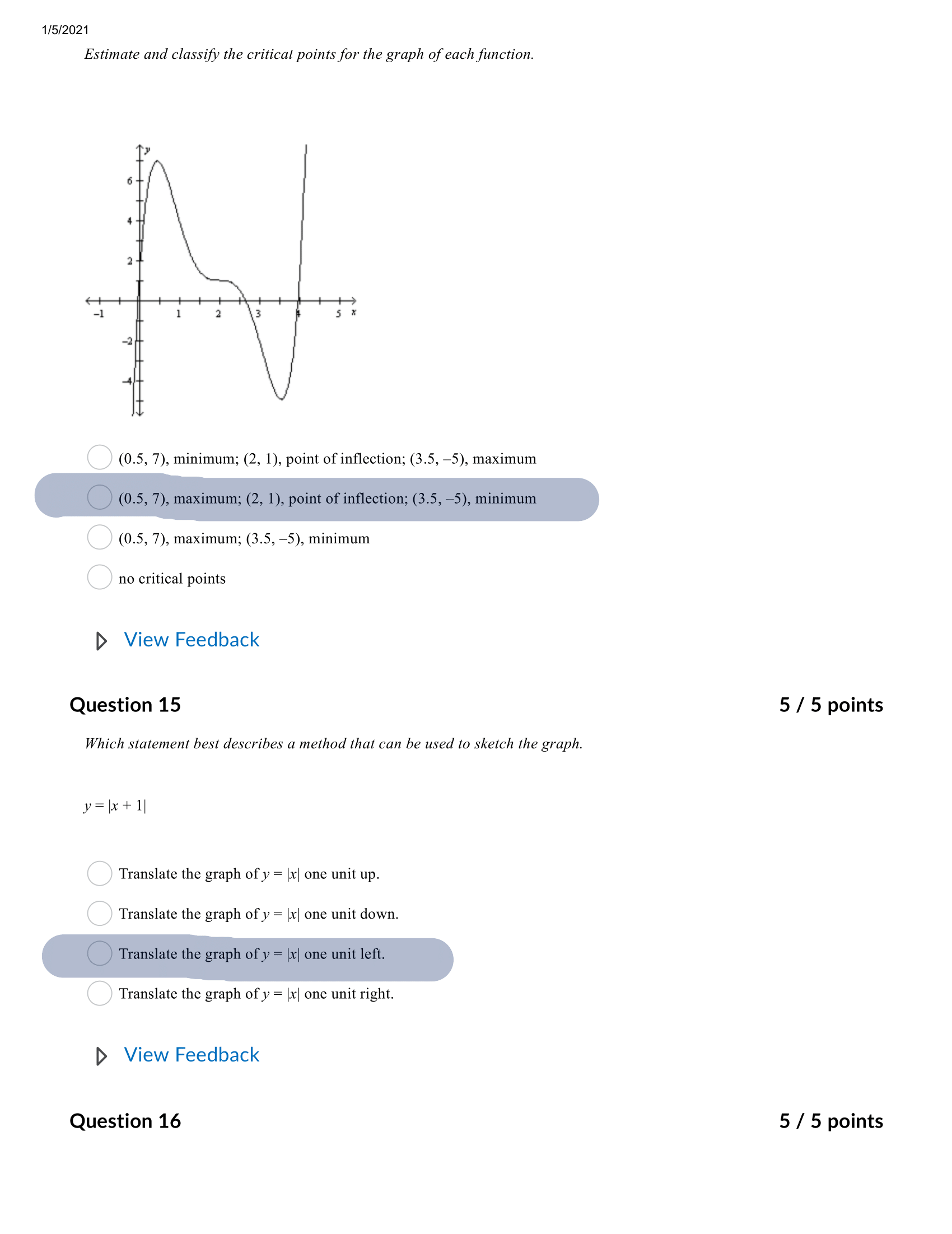
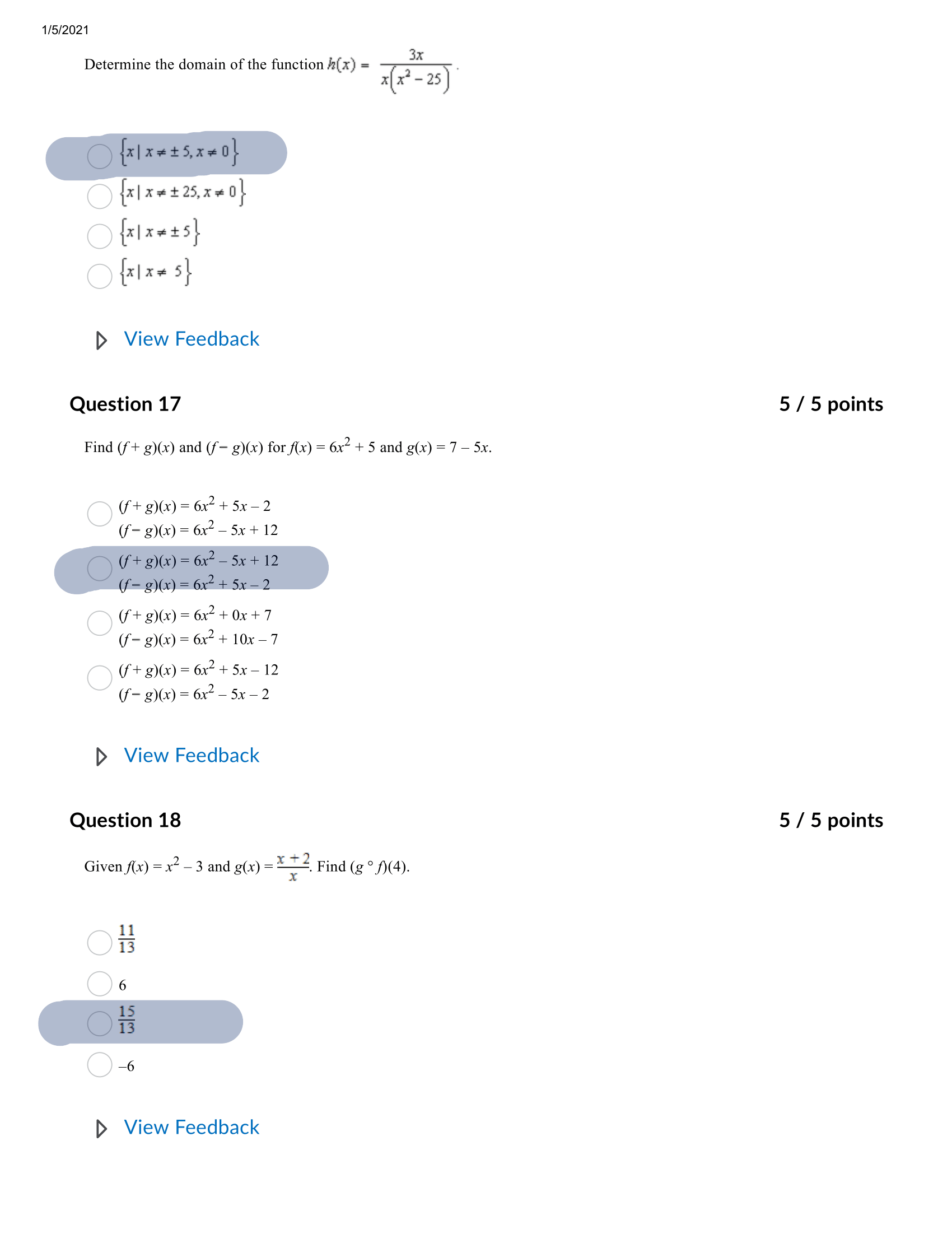
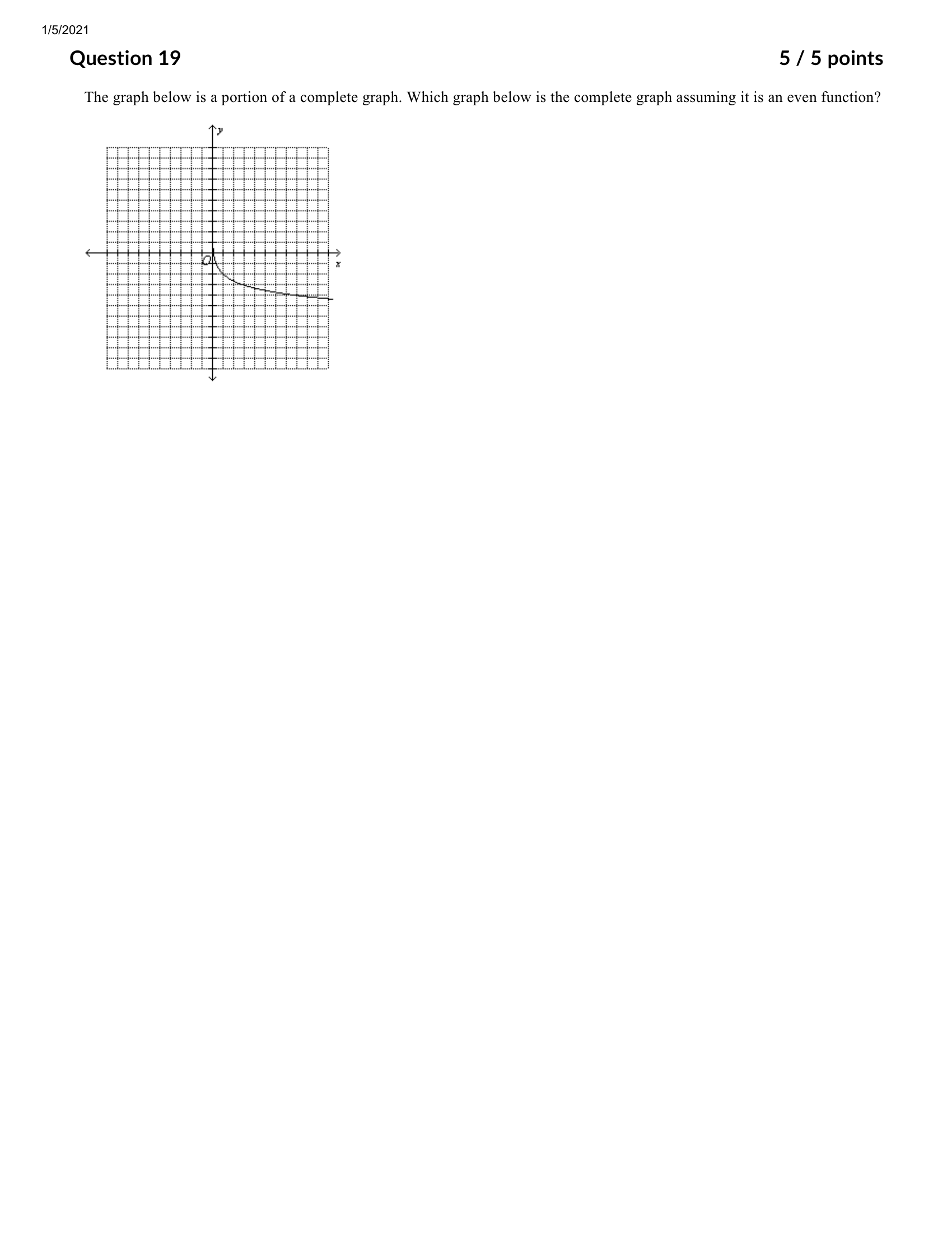
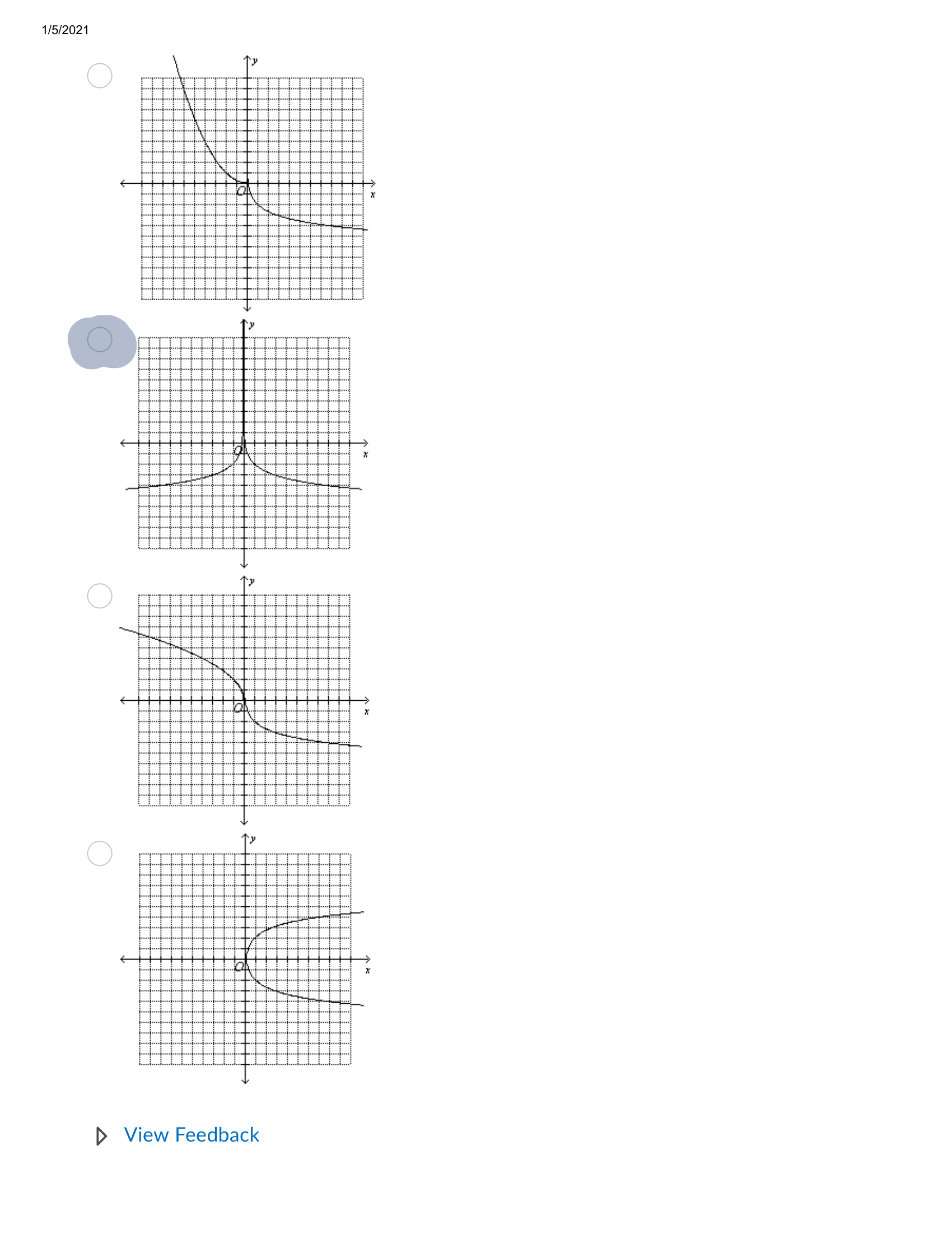
Quiz Submissions - \\[02\\] Lesson 2 Quiz x Written: Jan 4, 202. Submission View Congratulations! Your quiz has been submitted successfully. JMHS allows 2 attempts on quizzes so you may use your 2nd attempt to improve your score (if you want) or move on to your next lesson. The 2nd attempt is NOT required as long as your final course average is above 65%. The higher of the 2 attempts will be the score that counts towards the final average. Prior to your 2nd attempt, remember to review the answers from the lst attempt to help you study for the retake. Remember there may be new questions and they may be in a new order so you are encouraged to write down all answers before retaking. Please reach out to your teacher if you do not understand particular questions. Question 1 0 / 5 points x3 + 7X] + 10x State whether the graph of J((16) - 5 has innite discontinuity, jump discontinuity, point discontinuity, or is x + continuous. O The function has innite discontinuity. O The mction has point discontinuity. O The mction has jump discontinuity. v Hide Feedback Rationale: A function has a point or removable discontinuity if the funct' ' ' where except for a hole at x = c. Factor the numerator and if a term cancels the denominator, there in Question 2 5 / 5 points Without graphing, describe the end behavior of the graph of the function. 1/5/2021 Ax) = 1- 2x2 - x3 ASx -+ 0o, f (x) - -00. Asx - -0o, f (x) - co. As x - co, f (x) - -00. Asx - -0o, f (x) - -co. Asx - 00, f (x) - 0. As x - - co, f (x) - -00. O Asx - co, f (x) - 00. As x - - 0o, f (x) - 0. D View Feedback Question 3 5 / 5 points Graph the function defined by f(x) = |-x + 1|.1/512021 D View Feedback Question 4 0 / 5 points State the domain of f o g. Then nd f o g, including any additional restrictions necessary on the domain of the composition. 1/5/2021 ackY7 g(x) = hm 3 v Hide Feedback Rationale: The composition of function f with function g is dened by [f g](x) =f[g(x)]. The domain must be restricted to values of x that result in a non-zero denominator and those that do not result in taking the square root of a negative number. Question 5 5 / 5 points Determine between which consecutive integers the real zeros of x) - 313 - 5x2 + 5x+ 7 are located on the interval [10, 10]. If the zero occurs at an integer, write the integer. 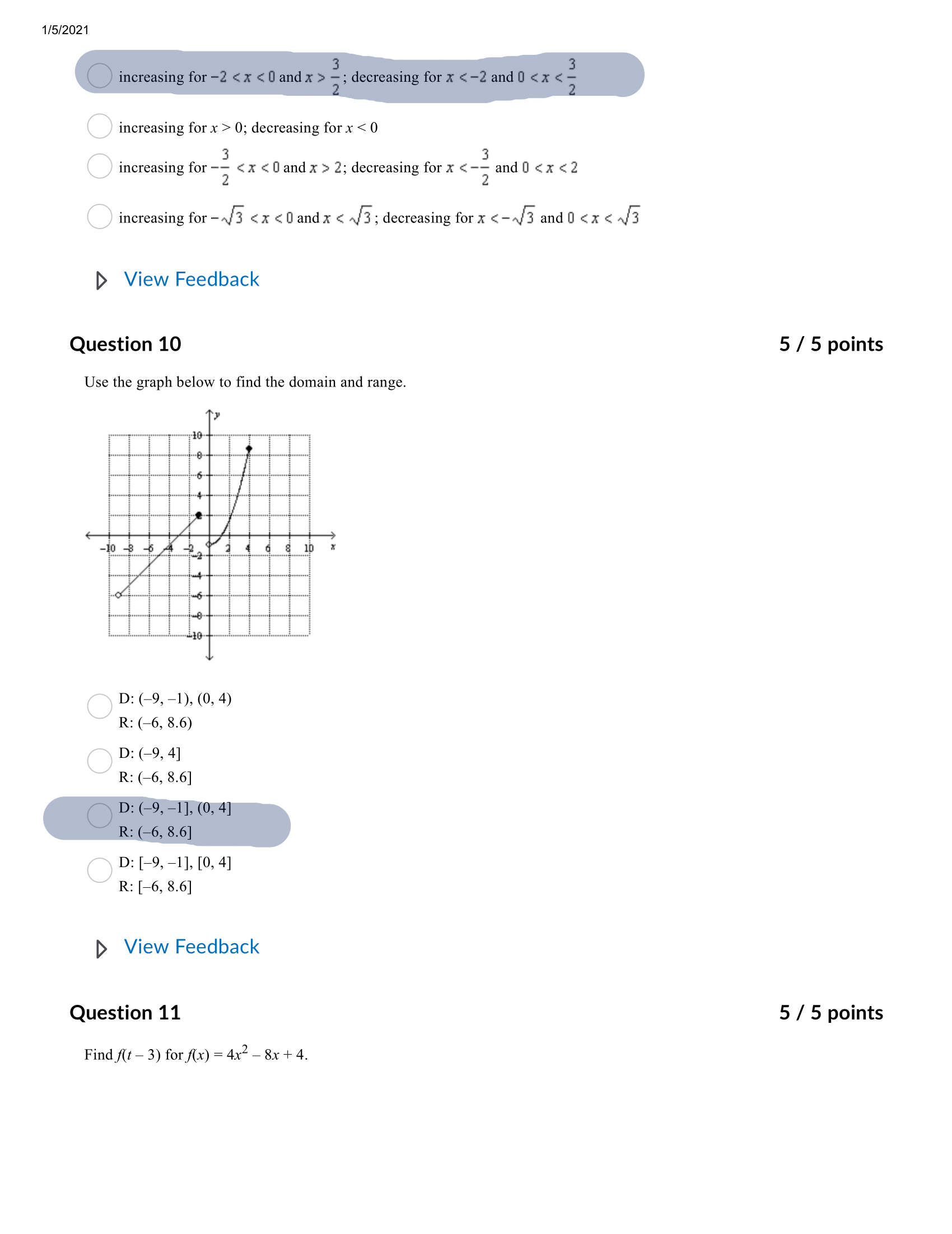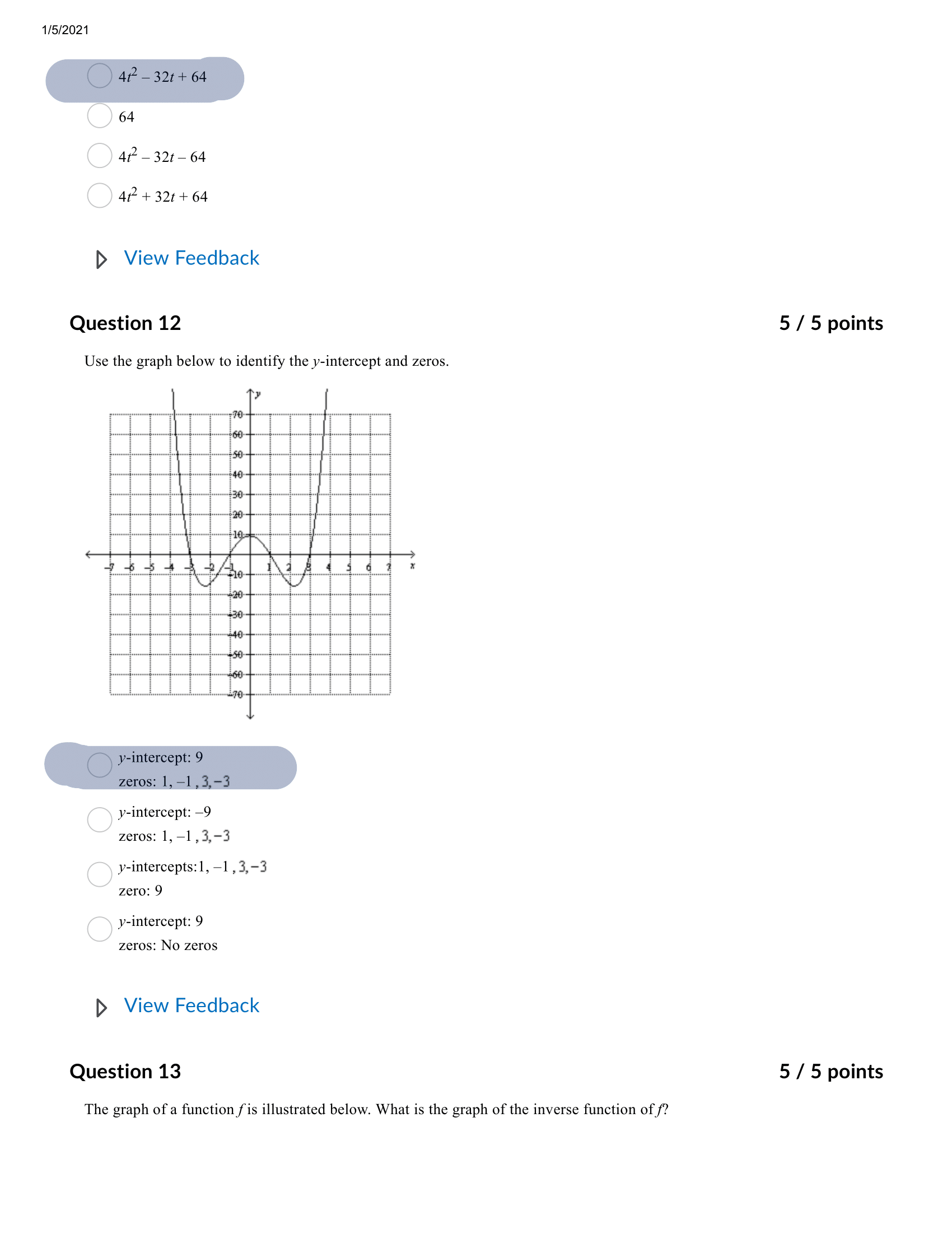Oi View Feedback Question 8 For which interval(s) is the function increasing and decreasing? y - 3x3 - 161+ 2 0 increasing for 2.31 2.31 0 increasing for x 2.31; decreasing for -2.31 E [> View Feedback Question 9 For which interval(s) is the function increasing and decreasing? y- I2x'+x-6| 5 / 5 points 5 / 5 points 5 / 5 points 1/5/2021 increasing for -2 -; decreasing for x 0; decreasing for x 2; decreasing for x