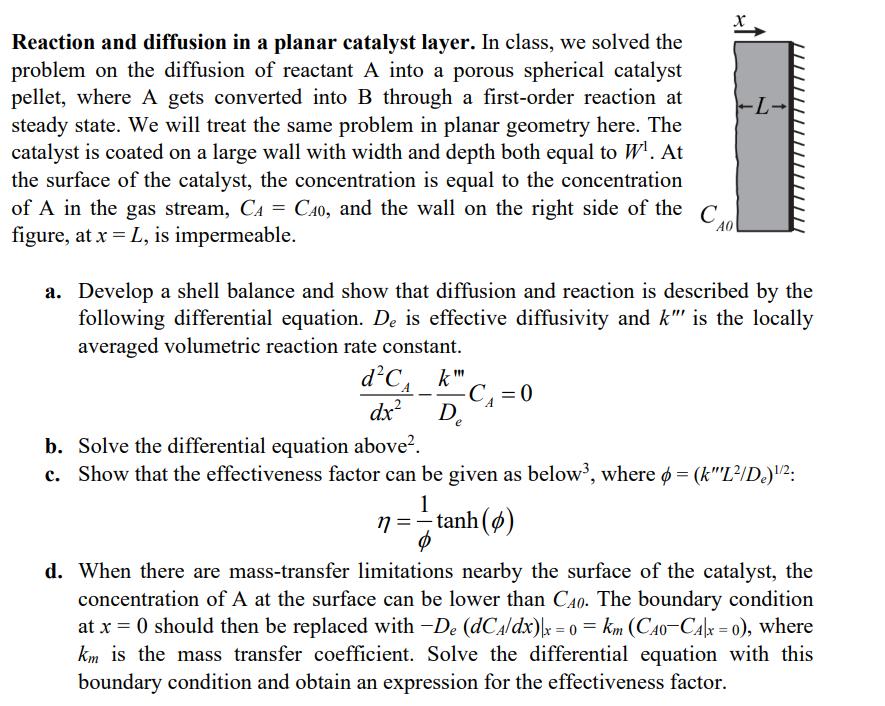
Reaction and diffusion in a planar catalyst layer. In class, we solved the problem on the diffusion of reactant A into a porous spherical catalyst pellet, where A gets converted into B through a first-order reaction at steady state. We will treat the same problem in planar geometry here. The catalyst is coated on a large wall with width and depth both equal to W. At the surface of the catalyst, the concentration is equal to the concentration of A in the gas stream, CA = CA0, and the wall on the right side of the figure, at x=L, is impermeable. == AO -7- a. Develop a shell balance and show that diffusion and reaction is described by the following differential equation. De is effective diffusivity and k" is the locally averaged volumetric reaction rate constant. dC dx b. Solve the differential equation above. - k" -C = 0 . e c. Show that the effectiveness factor can be given as below, where = (k"L/De)"/: 1 n=-tanh(4) d. When there are mass-transfer limitations nearby the surface of the catalyst, the concentration of A at the surface can be lower than C40. The boundary condition at x = 0 should then be replaced with -De (dCA/dx)|x = 0 = km (CAO-CA|x = 0), where km is the mass transfer coefficient. Solve the differential equation with this boundary condition and obtain an expression for the effectiveness factor.