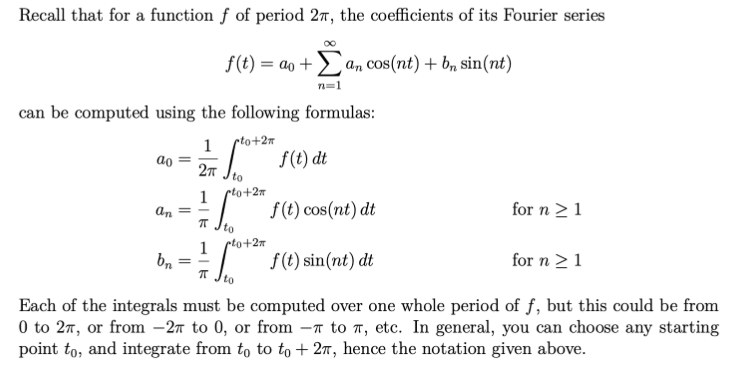
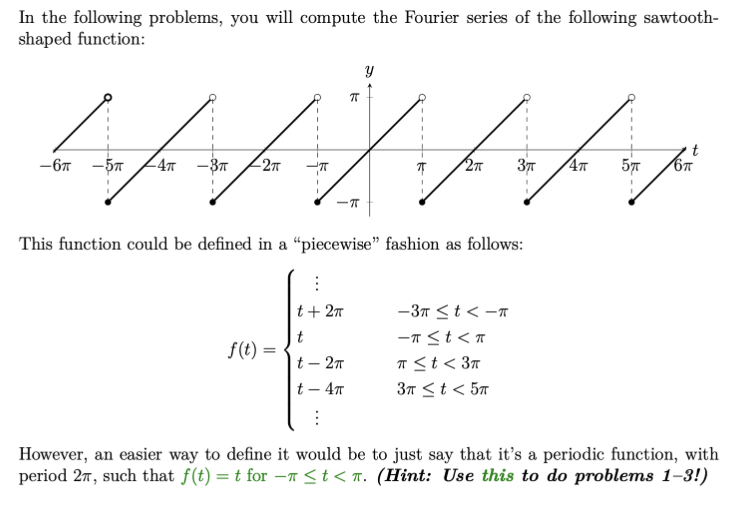
Recall that for a function f of period 2, the coefficients of its Fourier series f(t) = a + an cos(nt) + b, sin(nt) can be computed using the following formulas: 1 to+2 ao f(t) dt 2T to to+2 an = f(t) cos(nt) dt for n 1 to to+2 1 bn f(t) sin(nt) dt for n 1 to Each of the integrals must be computed over one whole period of f, but this could be from 0 to 2, or from -2 to 0, or from - to , etc. In general, you can choose any starting point to, and integrate from to to to +2, hence the notation given above. In the following problems, you will compute the Fourier series of the following sawtooth- shaped function: Y -6-5 -4-3 -2T - t 2T 4 57 6 This function could be defined in a "piecewise" fashion as follows: B t + 2 -3 t