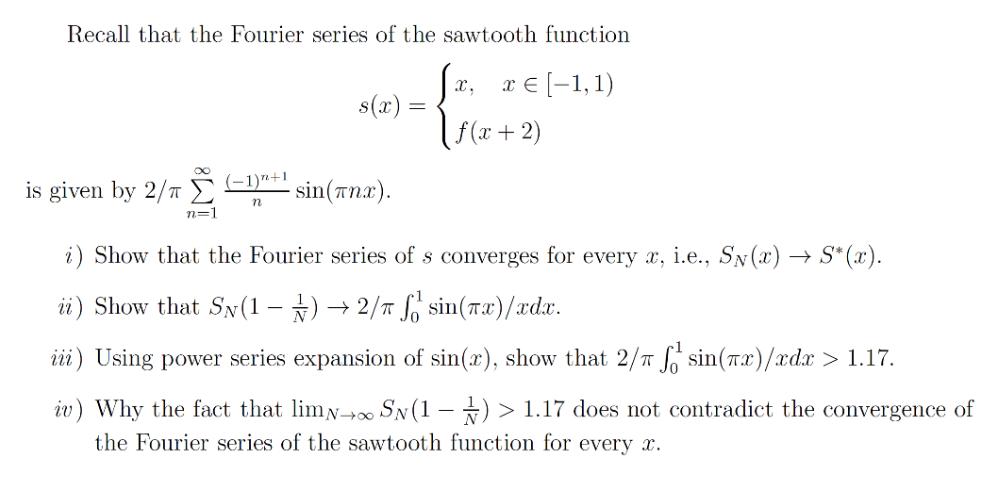
Recall that the Fourier series of the sawtooth function x, x = [-1, 1) f(x+2) s(x) = = is given by 2/(-1)n+1 n=1 -sin(nx). i) Show that the Fourier series of s converges for every x, i.e., Sy(x) S*(x). ii) Show that Sw(1 - ) 2/T [sin(Tx)/d. iii) Using power series expansion of sin(x), show that 2/ f sin(x)/xdx > 1.17. iv) Why the fact that limx SN (1) > 1.17 does not contradict the convergence of the Fourier series of the sawtooth function for every x.