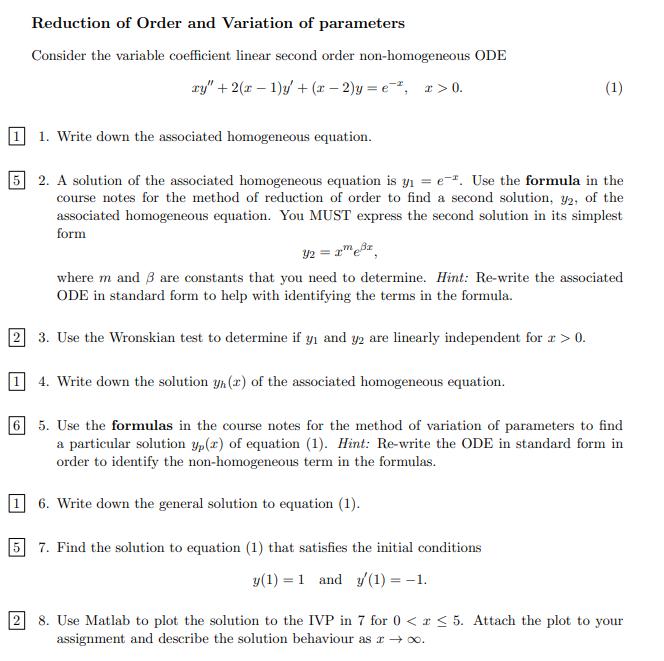
Reduction of Order and Variation of parameters Consider the variable coefficient linear second order non-homogeneous ODE ry" + 2(x - 1)y + (x-2)y=e , x>0. 1. Write down the associated homogeneous equation. 2. A solution of the associated homogeneous equation is y = e. Use the formula in the course notes for the method of reduction of order to find a second solution, 32, of the associated homogeneous equation. You MUST express the second solution in its simplest form (1) 32 = x, where m and 3 are constants that you need to determine. Hint: Re-write the associated ODE in standard form to help with identifying the terms in the formula. 2 3. Use the Wronskian test to determine if y and y2 are linearly independent for a > 0. 4. Write down the solution yn (r) of the associated homogeneous equation. 6 5. Use the formulas in the course notes for the method of variation of parameters to find a particular solution yp(x) of equation (1). Hint: Re-write the ODE in standard form in order to identify the non-homogeneous term in the formulas. 6. Write down the general solution to equation (1). 7. Find the solution to equation (1) that satisfies the initial conditions y(1) = 1 and (1) = -1. 8. Use Matlab to plot the solution to the IVP in 7 for 0 < < 5. Attach the plot to your assignment and describe the solution behaviour as a .