Refer to Table 14.13 (a tabulation of all ways three dice can fall, ignoring order). Now lets define a new game where a success occurs when the three dice rolled are consecutive numbers (for example: (4, 3, 2)). (a) Using the table, count the number of ways one can have a success. In one round of this game, what is the probability of a success? (b) Use Cardanos argument (section 14.3.1) or similarly Bernoullis argument (section 18.1.1) in counting the number of ways to succeed in n repeated trials of this game. Then, follow Bernoullis argument in Ars conjectandi (18.1.1) to answer the question: how many rounds should this roll be done so that two people have even chances of winning (or as close to this as possible)?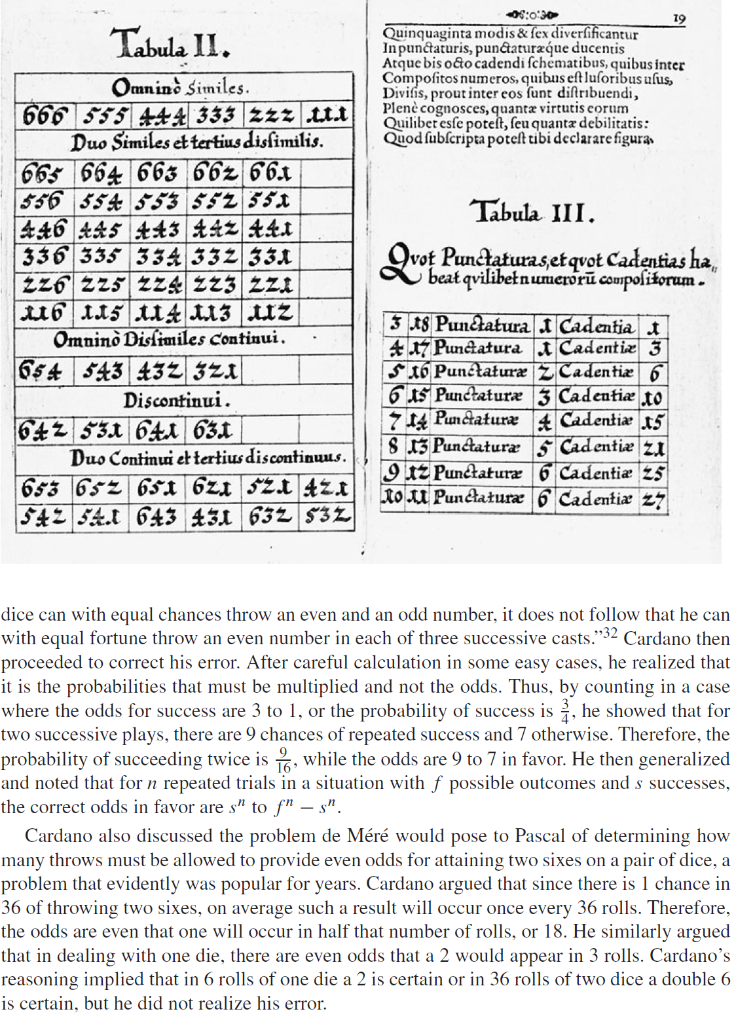

79 08:0:30 Quinquaginta modis & fex diverificantur In pun&aturis, pun&aturque ducentis Atque bis o&o cadendi chematibus, quibus inter Compoitos numeros, quibus eft luoribus uus, Diviis, prout inter eos unt diftribuendi, Plen cognosces, quant virtutis corum Quilibet ese poteft, feu quant debilitatis: Quod ubcripta potelt tibi declarare figura, Tabula III. Tabula II. Omnin Similes. 666 555 444 333 Zzz .utt Duo Similes et tertius disimilis. 663 664 663 662 66.4 556 554 553 552 351 446 445 443 444 44.1 336 335 334 332 33.1 226 225 Zz& ZZ3 ZZI 16 115 114 113 uz Omnin Disfimiles continui. 654 543 432 321 Discontinui. 642 53.1 64.1 63. Duo Continui et tertius discontinuus. 653 652 651 6z. 2. Azt 542 54.0 643 431 632 53Z vot beat quilibet numeror compoitorum. Cadentias ha, 3.18 Puntatura I Cadentia t 4.17 Punctatura i Cadentiz 3 5 16 Puntatur z Cadenti 6 6 15 Punctatur 3 Cadenti to 7 14 Punctatur 4 Cadentia 15 8 13 Punctatur 5 Cadenti zi I iz Punctatura 6 Cadenti 25 tout Puncatura 6 Cadenti dice can with equal chances throw an even and an odd number, it does not follow that he can with equal fortune throw an even number in each of three successive casts."32 Cardano then proceeded to correct his error. After careful calculation in some easy cases, he realized that it is the probabilities that must be multiplied and not the odds. Thus, by counting in a case where the odds for success are 3 to 1, or the probability of success is , he showed that for two successive plays, there are 9 chances of repeated success and 7 otherwise. Therefore, the probability of succeeding twice is 16, while the odds are 9 to 7 in favor. He then generalized and noted that for n repeated trials in a situation with f possible outcomes and s successes, the correct odds in favor are s" to fh - s". Cardano also discussed the problem de Mr would pose to Pascal of determining how many throws must be allowed to provide even odds for attaining two sixes on a pair of dice, a problem that evidently was popular for years. Cardano argued that since there is 1 chance in 36 of throwing two sixes, on average such a result will occur once every 36 rolls. Therefore, the odds are even that one will occur in half that number of rolls, or 18. He similarly argued that in dealing with one die, there are even odds that a 2 would appear in 3 rolls. Cardano's reasoning implied that in 6 rolls of one die a 2 is certain or in 36 rolls of two dice a double 6 is certain, but he did not realize his error. 79 08:0:30 Quinquaginta modis & fex diverificantur In pun&aturis, pun&aturque ducentis Atque bis o&o cadendi chematibus, quibus inter Compoitos numeros, quibus eft luoribus uus, Diviis, prout inter eos unt diftribuendi, Plen cognosces, quant virtutis corum Quilibet ese poteft, feu quant debilitatis: Quod ubcripta potelt tibi declarare figura, Tabula III. Tabula II. Omnin Similes. 666 555 444 333 Zzz .utt Duo Similes et tertius disimilis. 663 664 663 662 66.4 556 554 553 552 351 446 445 443 444 44.1 336 335 334 332 33.1 226 225 Zz& ZZ3 ZZI 16 115 114 113 uz Omnin Disfimiles continui. 654 543 432 321 Discontinui. 642 53.1 64.1 63. Duo Continui et tertius discontinuus. 653 652 651 6z. 2. Azt 542 54.0 643 431 632 53Z vot beat quilibet numeror compoitorum. Cadentias ha, 3.18 Puntatura I Cadentia t 4.17 Punctatura i Cadentiz 3 5 16 Puntatur z Cadenti 6 6 15 Punctatur 3 Cadenti to 7 14 Punctatur 4 Cadentia 15 8 13 Punctatur 5 Cadenti zi I iz Punctatura 6 Cadenti 25 tout Puncatura 6 Cadenti dice can with equal chances throw an even and an odd number, it does not follow that he can with equal fortune throw an even number in each of three successive casts."32 Cardano then proceeded to correct his error. After careful calculation in some easy cases, he realized that it is the probabilities that must be multiplied and not the odds. Thus, by counting in a case where the odds for success are 3 to 1, or the probability of success is , he showed that for two successive plays, there are 9 chances of repeated success and 7 otherwise. Therefore, the probability of succeeding twice is 16, while the odds are 9 to 7 in favor. He then generalized and noted that for n repeated trials in a situation with f possible outcomes and s successes, the correct odds in favor are s" to fh - s". Cardano also discussed the problem de Mr would pose to Pascal of determining how many throws must be allowed to provide even odds for attaining two sixes on a pair of dice, a problem that evidently was popular for years. Cardano argued that since there is 1 chance in 36 of throwing two sixes, on average such a result will occur once every 36 rolls. Therefore, the odds are even that one will occur in half that number of rolls, or 18. He similarly argued that in dealing with one die, there are even odds that a 2 would appear in 3 rolls. Cardano's reasoning implied that in 6 rolls of one die a 2 is certain or in 36 rolls of two dice a double 6 is certain, but he did not realize his error