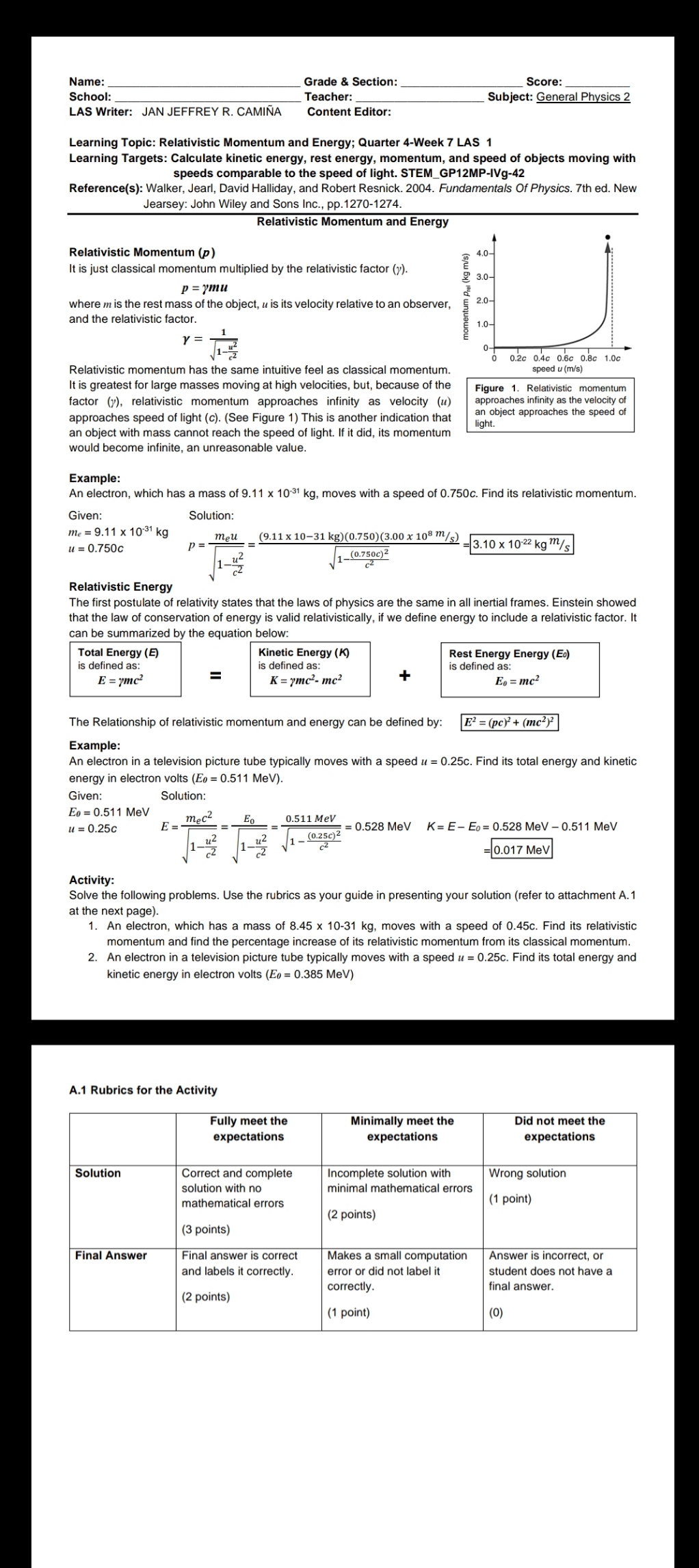
Relativistic Momentum and Energy
Name: Grade It Section: Score: School: Teacher: Subject: General Physics 2 LAS Writer: JAN JEFFREY R. CAMINA Content Editor: Learning Topic: Relativistic Momentum and Energy; Quarter 4-ka 1 LAs 1 Learning Targets: Calculate kinetic energy. rest energy. momentum, and speed of objects moving with speeds comparable to the speed of light. STEM_GPI2MP-lVg-42 Reterencqs): Walker, Jearl. David Halliday. and Robert Resnick. 2004. Fundamentals Of Physics. 7th ed. New Jearsey: John Wiley and Sons Inc.. pp.1270-1274. Relativistic Momentum and Energy Relativistic Momentum (p) It is just classical momentum multiplied by the relativistic factor (3:). 1' F TM" where m is the rest mass of lhe chisel, u is its velocity relativeio an observer, and the relativistic factor. 7N0? acne nun-awhile: min) a 1' a use ow can ca: 1.0: Relativistic momentum has the same intuitive feel as classical momentum. weed vim) It is greatest for large masses moving at high velocities. but. because of the Flnure 1' \"whim mmenwm factor (y), relativistic momentum approaches infinity as velocity (u) Hmenhasinlinilv as the velocity 0' approaches speed of light (c). (See Figure 1)This is another indie-tion that a?\" \"mac\": "'5 \"a" a an object with mass cannot reach the speed of light. If it did. its momentum would become infinite. an unreasonable value. Example: An electron, whid-i has a mass of 9.11 x 100' kg. moves with a speed of 0.7506. Find its relativistic momentum. Given: Solution: in.- = 9.11 x10'3' kg u = (17506 P (arson)? PT i: Relativistic Energy The rst postulate of relativity states that the laws of physics are the same in all inertial frames. Einstein showed that the law of conservaon of energy is valid relativistically. if we deline energy to include a relativistic factor. It can be summarized by the equation below: Total Energy (E) Kinetic Energy {K} Rut Energy Energy (Ea) is dened as: is dened as: is dened as: E = rmc' K = ymcz- mc' Ea = rut-z The Relationship of relativistic momentum and energy can be defined by: E' : (pclz+ (rim?)z Example: An electron in a television picture tube typically moves with a speed it = 0.25c. Find its total energy and kinetic energy in electron volts (Eu = 0.511 MeV}. Given: Solution: Eve 0.511 MeV u = 0.25:.- LEE =i =% = 0.523 MeV K: 5 Eu: 0.525 raw0.511 MeV \"2 It2 1 ' E [17 is J \"a n Activity: Solve the following problems. Use the rubrics as your guide in presenting your solution (refer to attachment A.1 at the next page). 1, An electron. which has a mass of 8.45 x 10-31 kg. moves with a speed of 0.45m Find its relativistic momentum and find the percentage increase at its relativistic momentum from its classical momentum. 2. An electron in a television picture tube typically moves with a speed it = 0.25s. Find its total energy and kinetic energy in electron volts (En - 0.385 MeV) A.1 Rubrlu for the Activity Fully meet the Minimally meet the Did not meet the expectations expectations expectetlonl Solution Correct and complete Incomplete solution with Wrong solution solution with no minimal mathematical errors _ mathematical errors '1 mm\" (2 points) (3 points) Final Answer Final answer is correct Makes a small computation Answer is incorrect. or and labels it correctly. error or did not label it student does not have a correctly. final answer. (2 points) (1 Wit\") (0)