Right click and open image in new tab.

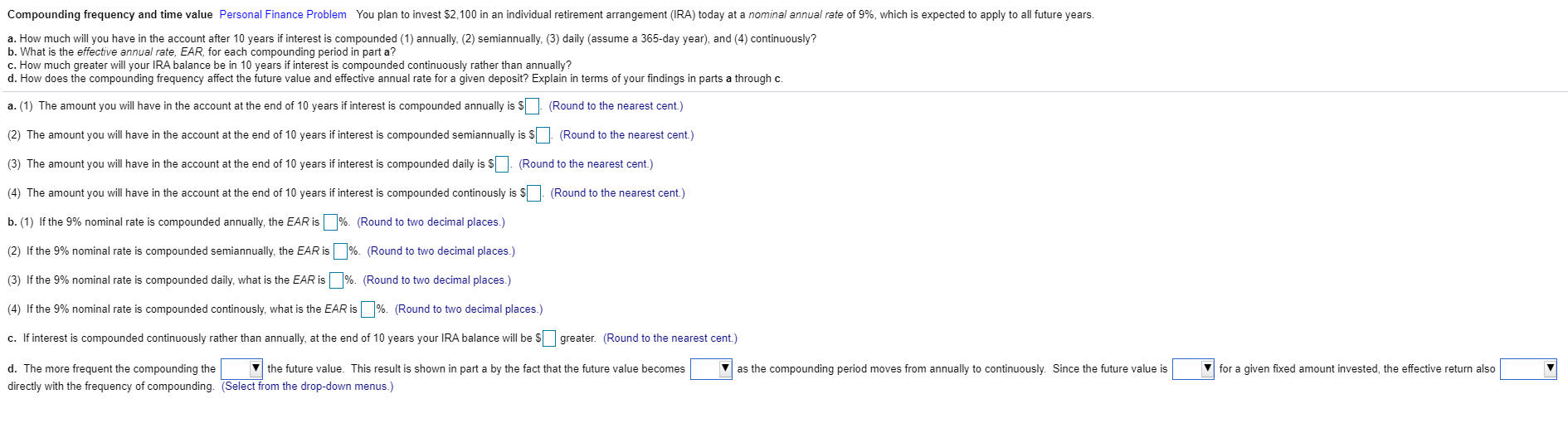
Compounding frequency and time value Personal Finance Problem you plan to invest $2,100 in an individual retirement arrangement (IRA) today at a nominal annual rate of 9%, which is expected to apply to all future years. a. How much will you have in the account after 10 years if interest is compounded (1) annually, (2) semiannually, (3) daily (assume a 365-day year), and (4) continuously? b. What is the effective annual rate, EAR, for each compounding period in part a? c. How much greater will your IRA balance be in 10 years if interest is compounded continuously rather than annually? d. How does the compounding frequency affect the future value and effective annual rate for a given deposit? Explain in terms of your findings in parts a through c. a. (1) The amount you will have in the account at the end of 10 years if interest is compounded annually is $ (Round to the nearest cent.) (2) The amount you will have in the account at the end of 10 years if interest is compounded semiannually is $ (Round to the nearest cent.) (3) The amount you will have in the account at the end of 10 years if interest is compounded daily is $ . (Round to the nearest cent.) (4) The amount you will have in the account at the end of 10 years if interest is compounded continously is $ . (Round to the nearest cent.) b. (1) If the 9% nominal rate is compounded annually, the EAR is %. (Round to two decimal places.) (2) If the 9% nominal rate is compounded semiannually, the EAR is %. (Round to two decimal places.) (3) If the 9% nominal rate is compounded daily, what is the EAR is %. (Round to two decimal places.) (4) If the 9% nominal rate is compounded continously, what is the EAR is %. (Round to two decimal places.) c. If interest is compounded continuously rather than annually, at the end of 10 years your IRA balance will be greater. (Round to the nearest cent.) as the compounding period moves from annually to continuously. Since the future value is for a given fixed amount invested, the effective return also d. The more frequent the compounding the the future value. This result is shown in part a by the fact that the future value becomes directly with the frequency of compounding. (Select from the drop-down menus.)