
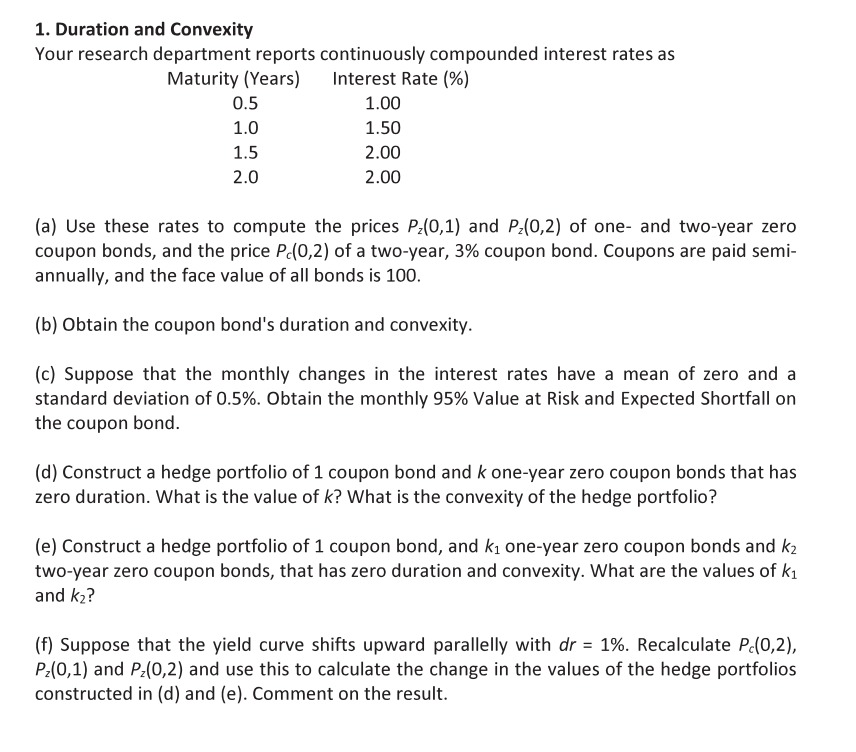

Solvethefollowing.:
Attachment.,
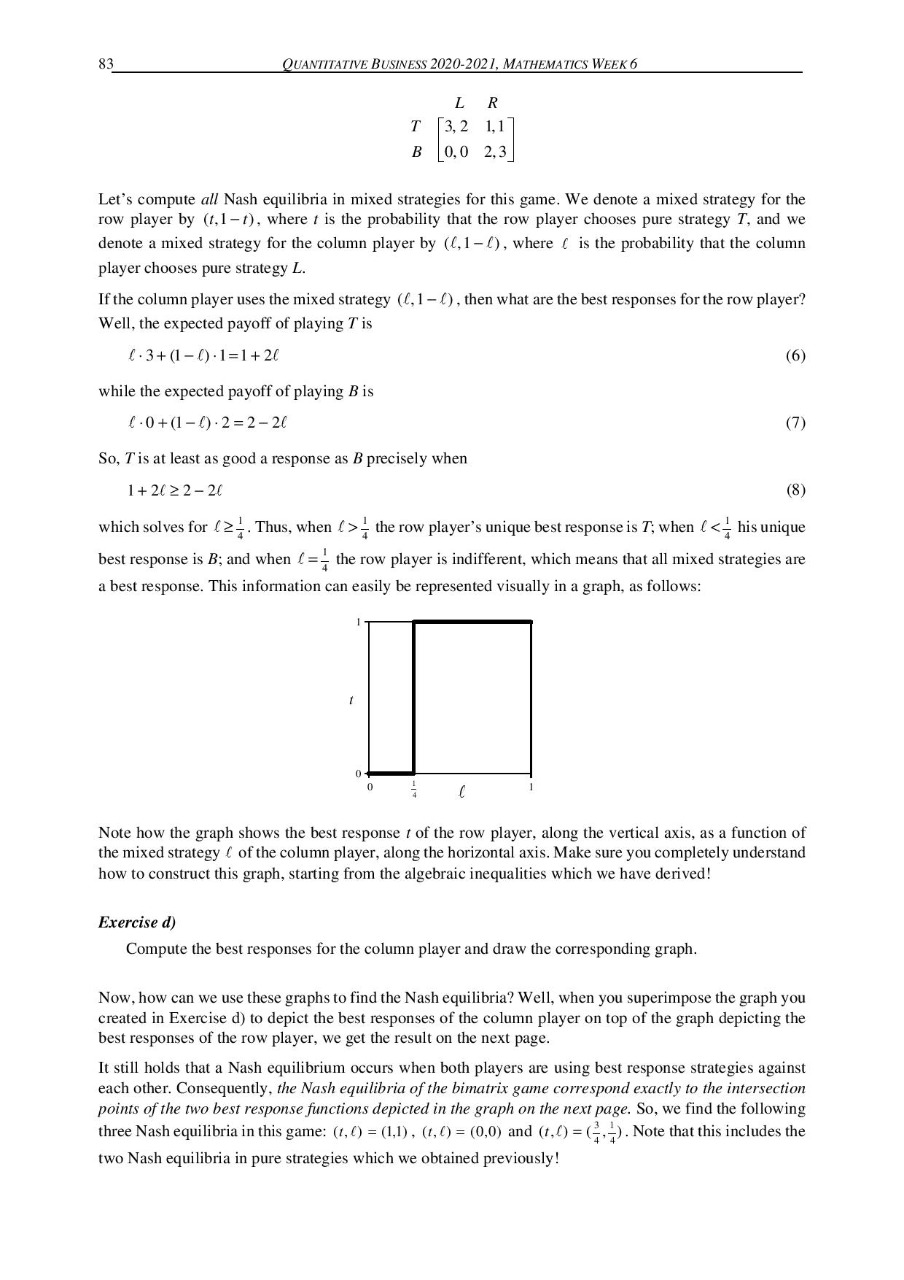
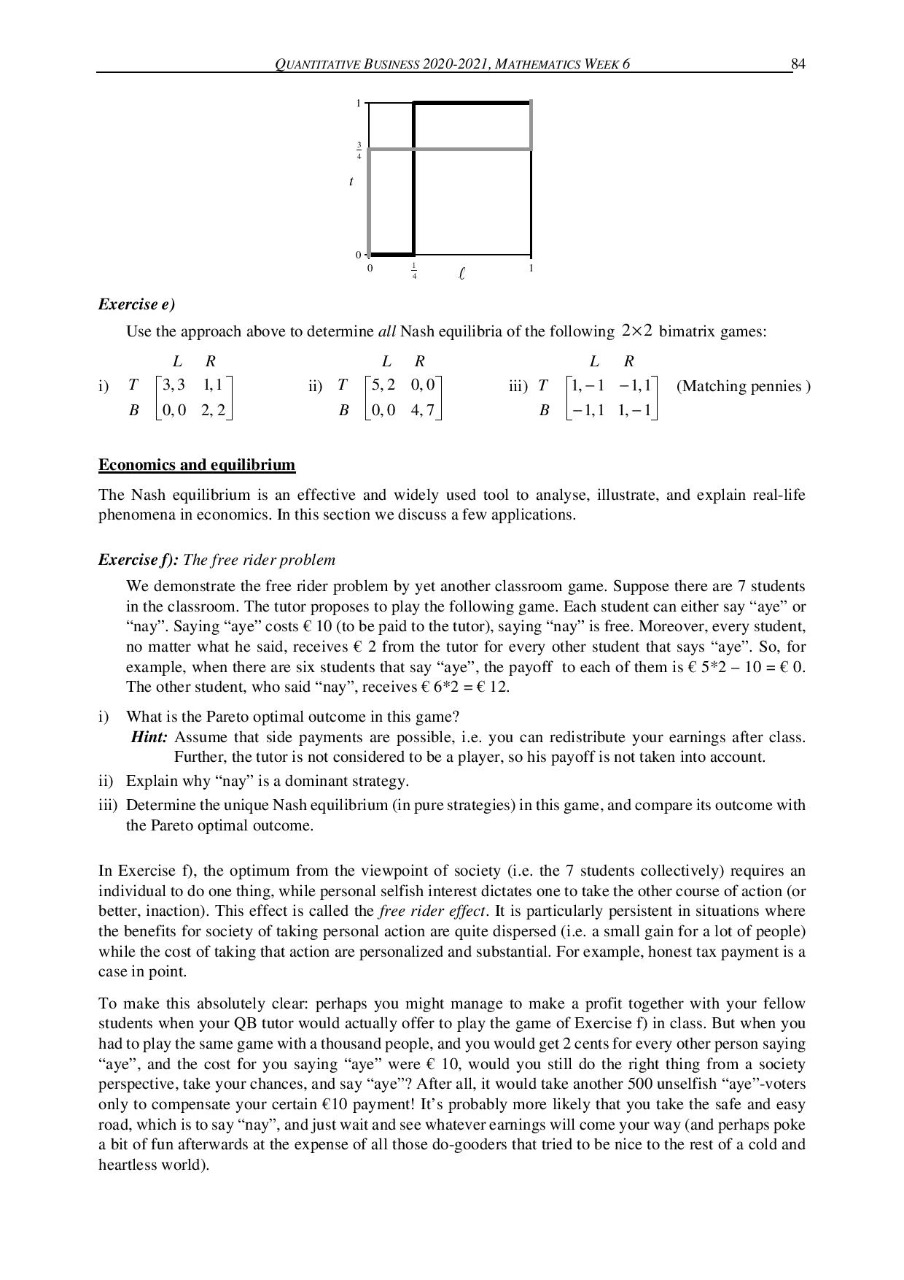
Problem 2. Investor A has a square root utility function (i.e., U(W) = VW), whereas Investor B has a logarithmic utility function (i.e., U(W) = In W). Both investors have initial wealth Wo = $1, 000 and must decide how much how much to invest in a bond and how much to invest in a stock. The current (t = 0) prices of the bond and stock are Bo and So respectively. Both investors expect to receive income from selling these securities at their t = 1 prices, which are B, for the bond and S, for the stock. Since the bond is riskless, its t = 1 price is known with certainty to be B1 = Bo(1+r), where r is the riskless rate of interest. The price of the stock at t = 1 can be high or low; i.e., it will be So(1+s) with probability .6 and it will be So(1-s) with probability .4. Furthermore, assume that r = .02 and s = .12. A. How much (in dollars and percentages) of Investor A's initial wealth should be invested in the stock and in the bond? B. How much (in dollars and percentages) of Investor B's initial wealth should be invested in the stock and in the bond? C. Who is more risk averse - Investor A or Investor B? Explain why.1. Duration and Convexity Your research department reports continuously compounded interest rates as Maturity [Years] Interest Rate (96] 0.5 1.00 1.0 1.50 1.5 2.00 2.0 2.00 (3} Use these rates to compute the prices le0,1} and No.2) of one- and taro-year zero coupon bonds, and the price .0403] of a two-year, 3% coupon bond. Coupons are paid semi- annually, and the face value of all bonds is 100. (b) Obtain the coupon bond's duration and convexity. (cl Suppose that the monthly changes in the interest rates have a mean of zero and a standard deviation of 0.5%. Obtain the monthly 95% Value at Risk and Expected Shortfall on the coupon bond. (d) Construct a hedge portfolio of 1 coupon bond and k one-year zero coupon bonds that has zero duration. What is the value of it? What is the convexity of the hedge portfolio? is) Construct a hedge portfolio of 1 coupon bond, and k1 one-year zero coupon bonds and k2 two-year zero coupon bonds, that has zero duration and convexity. What are the values of in and k2? (f) Suppose that the yield curve shifts upward parallelly with dr = 1%. Recalculate P(0,2), le0,1) and Pz[0,2] and use this to calculate the change in the values of the hedge portfolios constructed in [cl] and {e}. Comment on the result. 83 QUANTITATIVE BUSINESS 2020-2021. MATHEMATICS WEEK 6 L R T 3,2 1,1 B Lo,0 2.3 Let's compute all Nash equilibria in mixed strategies for this game. We denote a mixed strategy for the row player by (t. 1-1), where i is the probability that the row player chooses pure strategy 7, and we denote a mixed strategy for the column player by (6,1-(), where ( is the probability that the column player chooses pure strategy L. If the column player uses the mixed strategy ((, 1- (), then what are the best responses for the row player? Well, the expected payoff of playing T is ( .3+ (1-0).1=1+20 (6) while the expected payoff of playing B is ( .0+ (1-().2=2-20 (7) So, T is at least as good a response as B precisely when 1+2(22-20 (8) which solves for (2-. Thus, when (> - the row player's unique best response is 7; when (
















