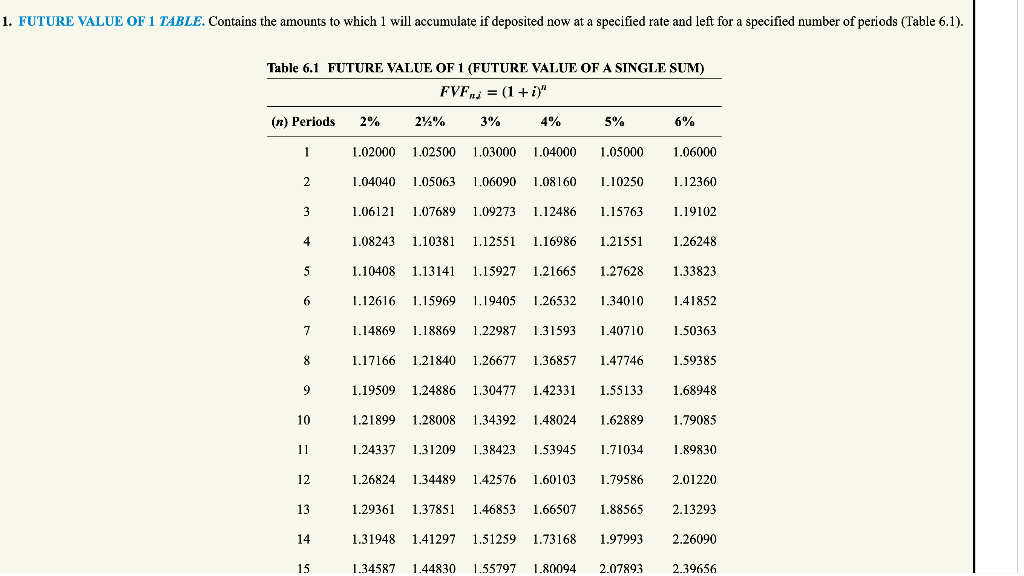
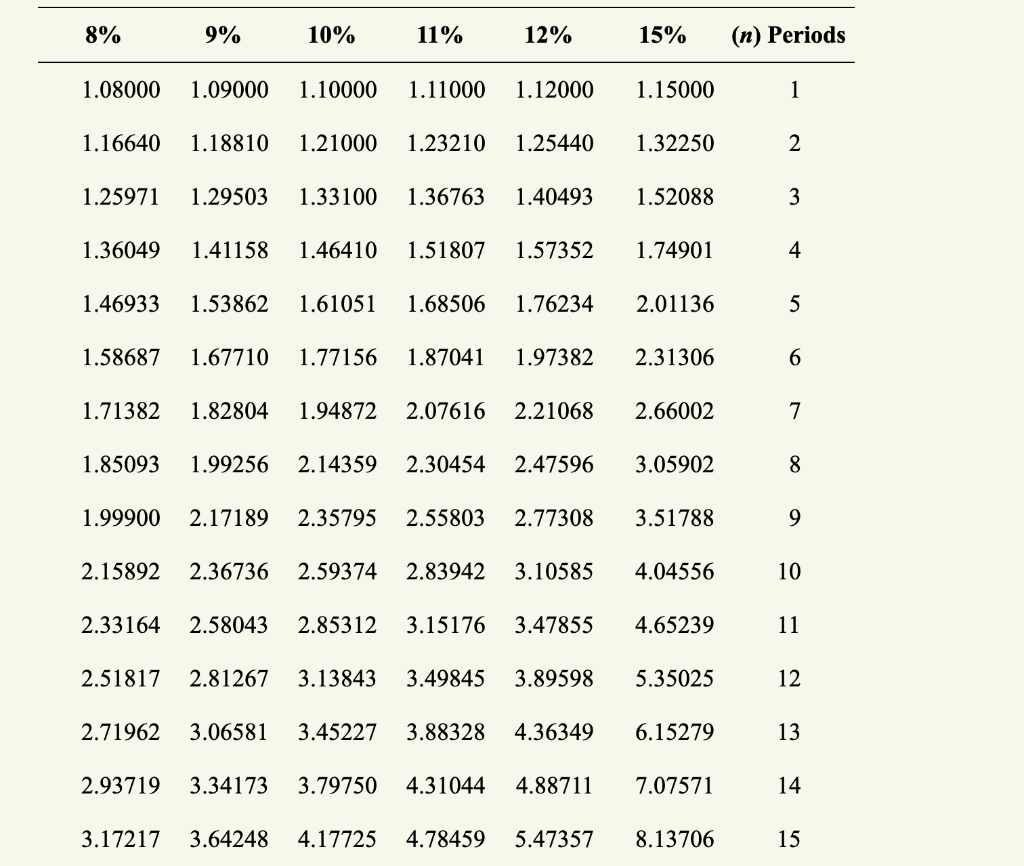
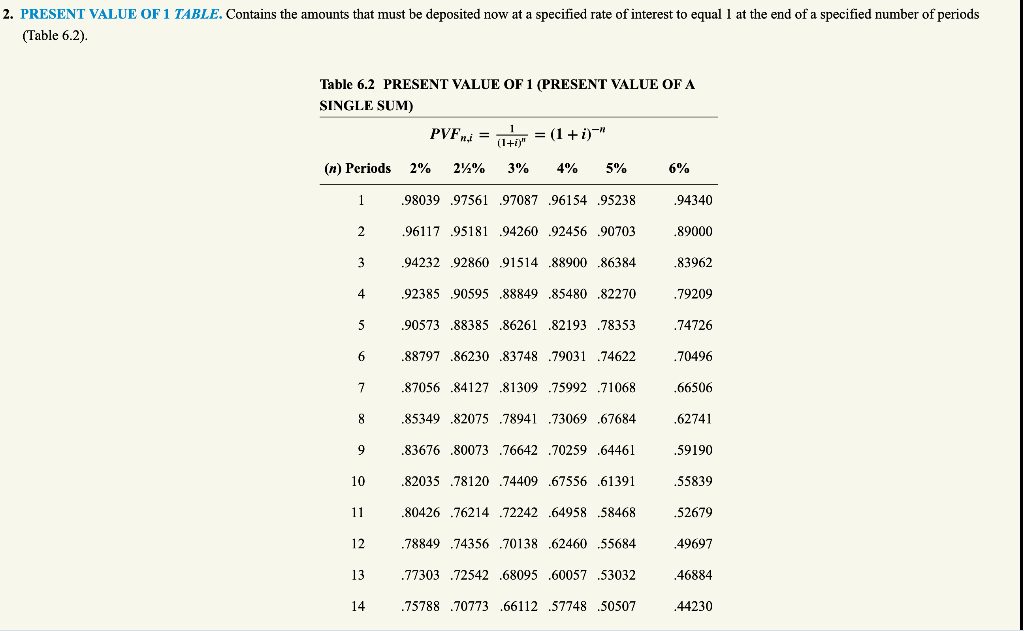
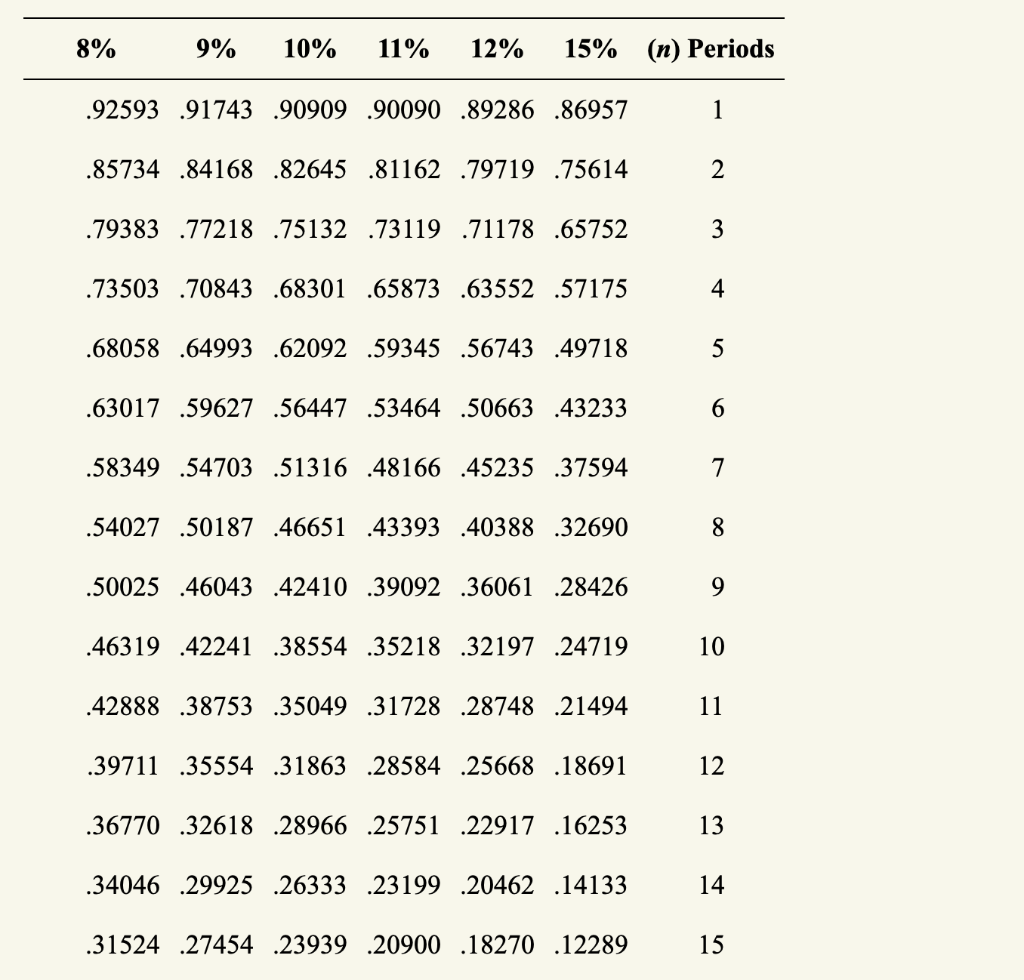
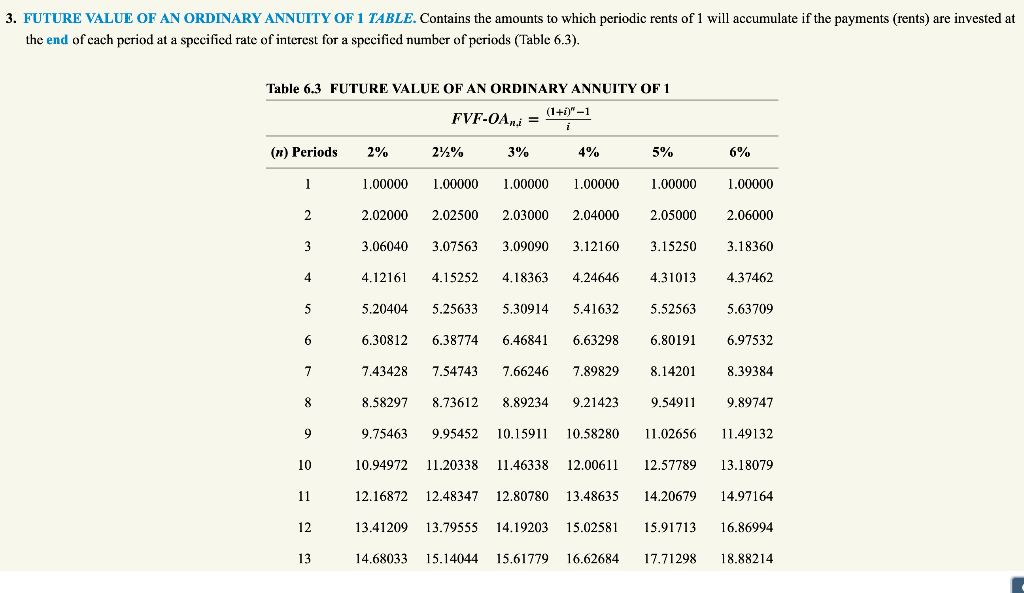
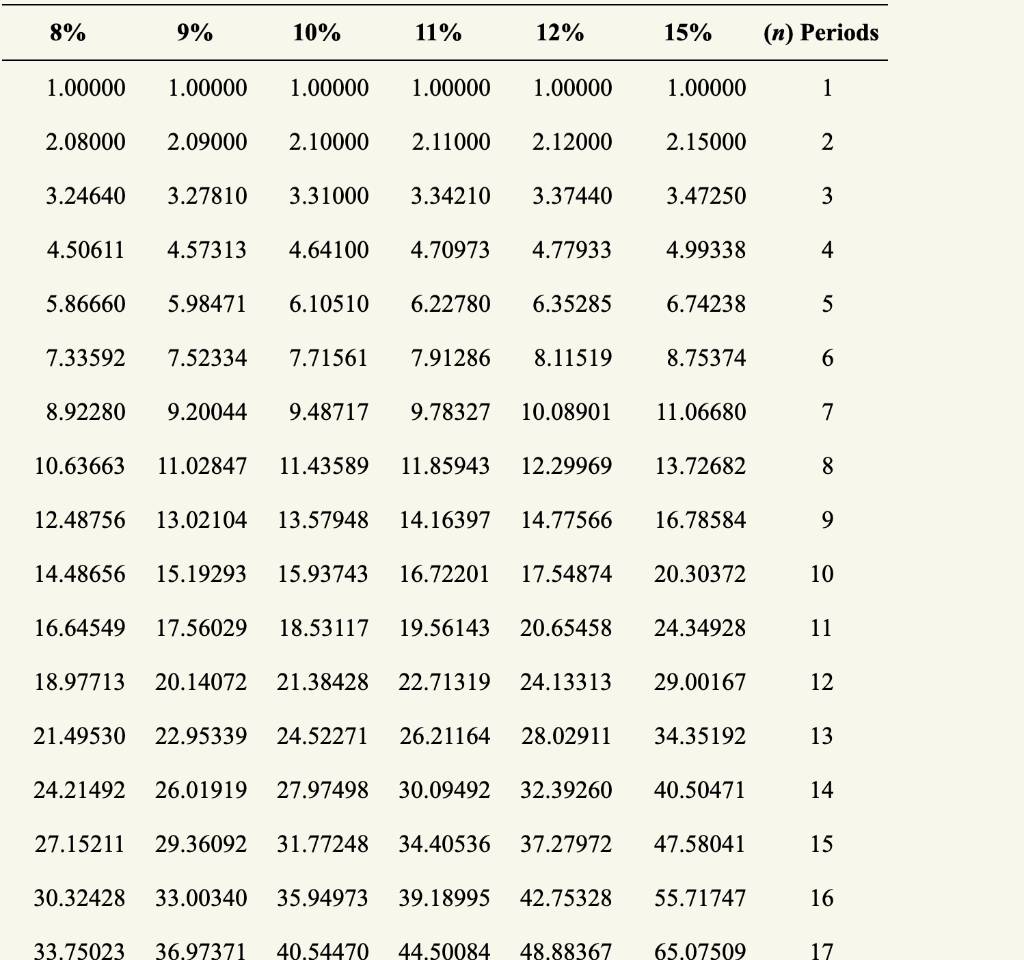
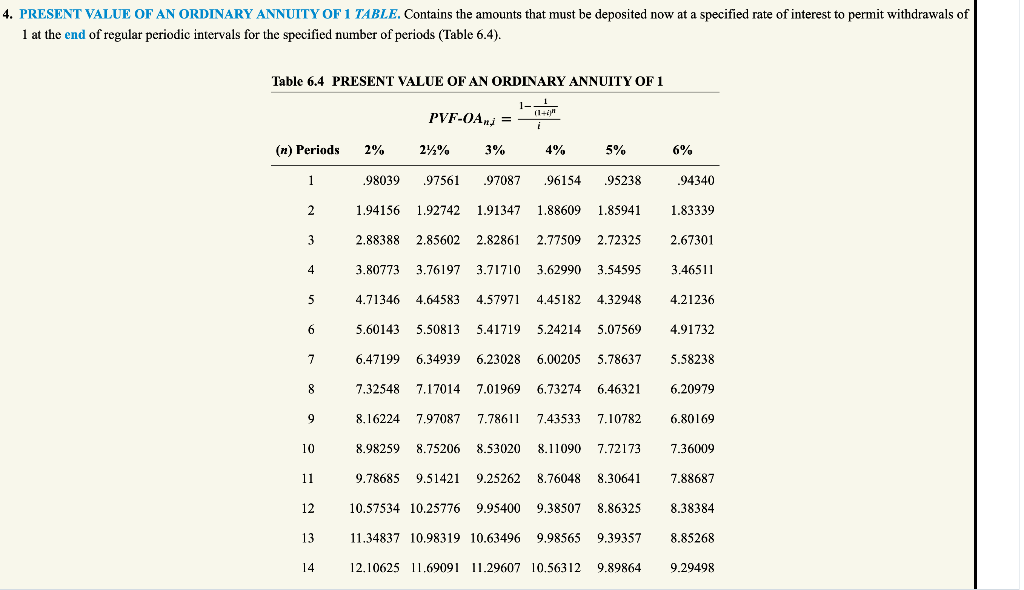
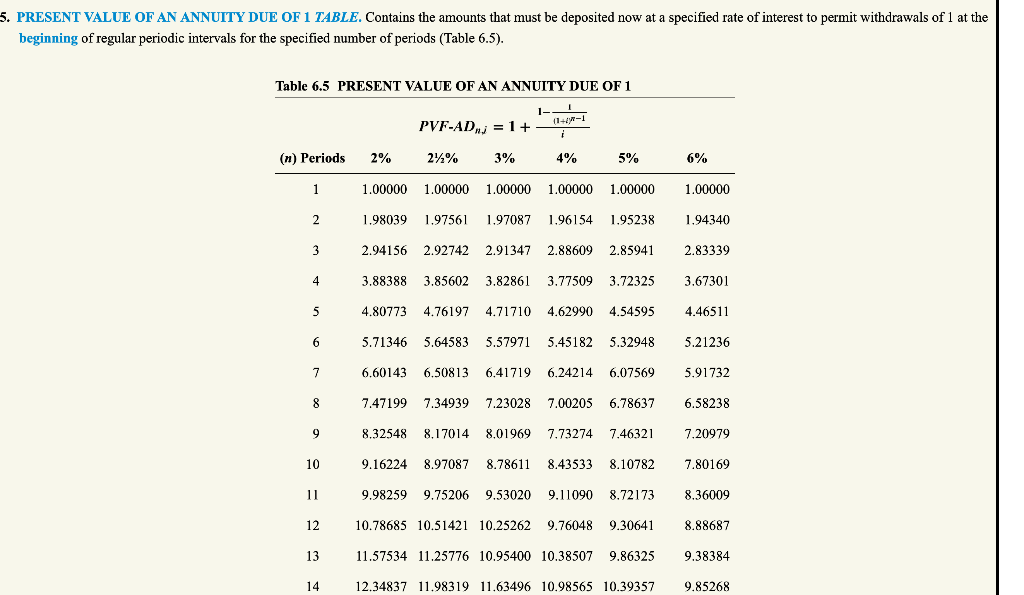
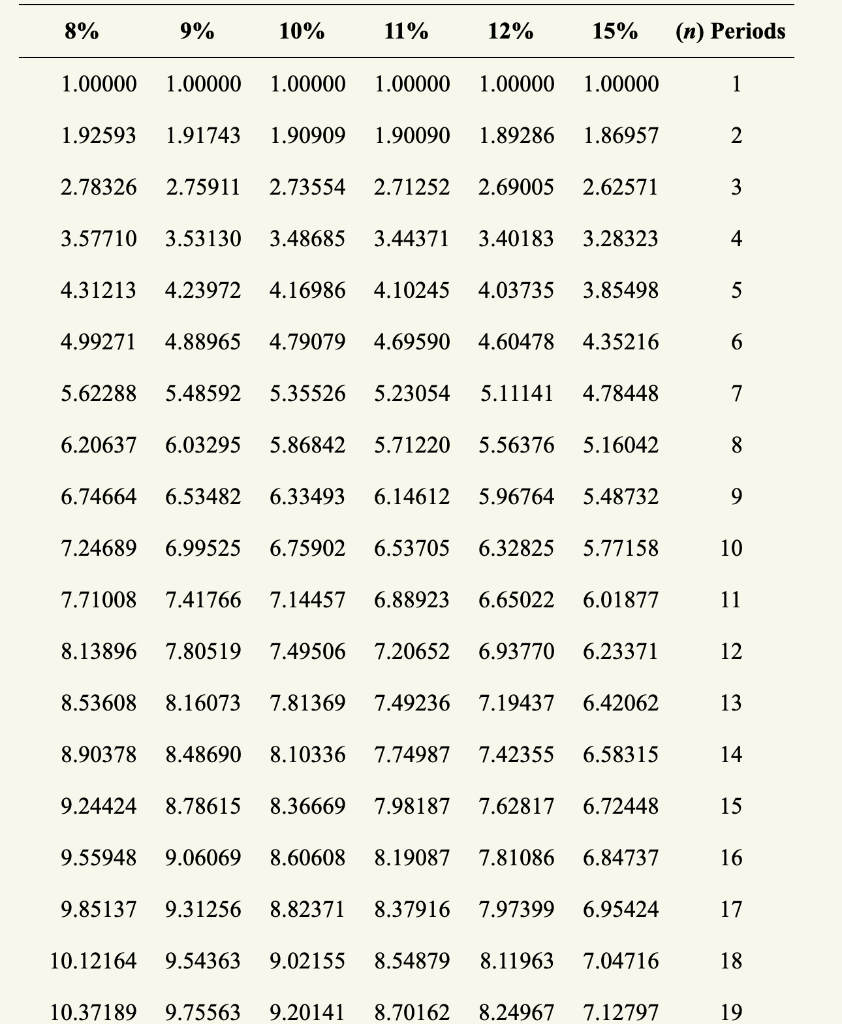
Sage Inc. manufactures an X-ray machine with an estimated life of 12 years and leases it to Pronghorn Medical Center for a period of 10 years. The normal selling price of the machine is $528, 109, and its guaranteed residual value at the end of the non-cancelable lease term is estimated to be $15,000. The hospital will pay rents of $64,000 at the beginning of each year. Sage incurred costs of $263,000 in manufacturing the machine and $14,200 in legal fees directly related to the signing of the lease. Sage has determined that the collectibility of the lease payments is probable and that the implicit interest rate is 5%. Pronghorn Medical Center has an incremental borrowing rate of 5% and an expected residual value at the end of the lease of $10,000. Click here to view factor tables (a2) x Your answer is incorrect. Try again. Compute the amount of the initial lease liability. (Round present value factor calculations to 5 decimal places, e.g. 1.25124 and the final answer to o decimal places e.g. 5,275.) Initial Lease Liability 503399 Click if you would like to Show Work for this question: Open Show Work 1. FUTURE VALUE OF 1 TABLE. Contains the amounts to which 1 will accumulate if deposited now at a specified rate and left for a specified number of periods (Table 6.1). Table 6.1 FUTURE VALUE OF 1 (FUTURE VALUE OF A SINGLE SUM) FVFnj = (1 + i)" (n) Periods 2% 2%2% 3% 4% 5% 6% 1.02000 1.02500 1.03000 1.04000 1.05000 1.06000 1.04040 1.05063 1.06090 1.08 160 1.10250 1.12360 1.06121 1.07689 1.09273 1.12486 1.15763 1.19102 1.21551 1.26248 1.27628 1.33823 1.08243 1.10408 1.12616 1.14869 1.17166 1.10381 1.13141 1.15969 1.18869 1.21840 1.12551 1.15927 1.19405 1.22987 1.26677 1.16986 1.21665 1.26532 1.31593 1.36857 1.41852 1.50363 1.40710 1.47746 1.59385 1.19509 1.24886 1.30477 1.42331 1.551331.68948 1.21899 1.28008 1.34392 1.48024 1.62889 1.79085 1.24337 1.31209 1.38423 1.53945 1.71034 1.89830 1.26824 1.34489 1.42576 1.60103 1.79586 2.01220 1.29361 1.37851 1.46853 1.66507 1.31948 1.41297 1.51259 1.73168 1.34587 1.44830 1.55797 1.80094 1.88565 2.13293 1.979932.26090 2.078932.39656 8% 9% 10% 11% 12% 15% (n) Periods 1.08000 1.09000 1.10000 1.11000 1.12000 1.15000 1 1.16640 1.18810 1.21000 1.23210 1.25440 1.32250 1.25971 1.29503 1.33100 1.36763 1.40493 1.52088 1.36049 1.41158 1.46410 1.51807 1.57352 1.74901 1.46933 1.53862 1.61051 1.68506 1.76234 2.01136 1.58687 1.67710 1.77156 1.87041 1.97382 1.71382 1.82804 1.94872 2.07616 2.21068 1.85093 1.99256 2.14359 2.30454 2.47596 2.31306 2.66002 3.05902 1.99900 2.17189 2.35795 2.55803 2.77308 3.51788 2.15892 2.36736 2.59374 2.83942 3.10585 4.04556 2.33164 2.58043 2.85312 3.15176 3.47855 4.65239 2.51817 2.71962 2.81267 3.06581 3.13843 3.49845 3.89598 3.45227 3.88328 4.36349 5.35025 6.15279 2.93719 3.34173 3.79750 4.31044 4.88711 3.17217 3.64248 4.17725 4.78459 5.47357 7.07571 8.13706 15 2. PRESENT VALUE OF 1 TABLE. Contains the amounts that must be deposited now at a specified rate of interest to equal 1 at the end of a specified number of periods (Table 6.2). Table 6.2 PRESENT VALUE OF 1 (PRESENT VALUE OF A SINGLE SUM) PVFn,i = tx = (1+i)" (n) Periods 2% 22% 3% 4% 5% 6% .94340 .98039 97561 97087 96154 95238 .96117 95181 94260 92456 90703 .89000 94232 92860 91514 88900 86384 ,83962 .79209 92385 90595 88849 .85480 82270 .90573 .88385 .86261 82193 78353 .88797 86230 83748 79031 74622 .74726 .70496 87056 .84127 81309 75992 71068 .66506 .62741 .59190 .55839 .85349 82075 78941 73069 67684 .83676 80073 76642 70259 .64461 .82035 78120 74409 67556 61391 .80426 76214 72242 64958 58468 .78849 .74356 70138 .62460 55684 .77303 72542 .68095 .60057 .53032 .75788 70773 66112 57748 50507 .52679 ,49697 46884 44230 | 8% 9% 10% 11% 12% 15% (n) Periods 1.92593 91743.90909 ,90090 .89286 86957 85734.84168 .82645 81162.79719.756142 1.79383.77218.75132 73119 71178.65752 3 173503.70843.68301 .65873.63552 .57175 .68058 .64993.62092 .59345 .56743 .49718 163017 .59627.56447 .53464 .50663 43233 158349 .54703 .51316 48166 45235 37594 154027.50187 46651 .43393 40388 .32690 150025 46043 42410 .39092 36061 .28426 146319 .42241 38554 .35218 .32197.24719 142888 .38753.35049 31728 28748 21494 139711.35554 .31863 .28584 .25668.18691 136770 .32618 28966 .25751.22917.16253 134046 .29925 26333 .23199 .20462 .14133 1.31524 27454 23939.20900 .18270 .12289 15 3. FUTURE VALUE OF AN ORDINARY ANNUITY OF 1 TABLE. Contains the amounts to which periodic rents of 1 will accumulate if the payments (rents) are invested at the end of each period at a specified rate of interest for a specified number of periods (Table 6.3). Table 6.3 FUTURE VALUE OF AN ORDINARY ANNUITY OF 1 FVF-OAni = "+"= (x) Periods 2% 24% 3% 4% 5% 1.00000 1.00000 100000 1.00000 1.00000 2.02000 2.02500 2.03000 2.04000 2.05000 6% 1.00000 2.06000 3.06040 3.07563 3.09090 3.12160 3.15250 3.18360 4.12161 4.15252 4.18363 4.31013 4.37462 5.20404 5.25633 5.30914 5.41632 5.52563 5.63709 6.30812 7.43428 6.38774 7.54743 6.46841 7.66246 6.63298 7.89829 6.80191 8.14201 6.97532 8.39384 8.89234 9.21423 9.54911 9.89747 8.58297 9.75463 8.73612 9.95452 10.15911 10.58280 11.02656 12.57789 11.49132 13.18079 10.94972 11.20338 11.46338 12.00611 12.16872 12.48347 12.80780 13.48635 14.20679 14.97164 13.41209 13.79555 14.19203 15.02581 15.91713 16.86994 13 14.68033 15.14044 15.61779 16.62684 17.71298 18.88214 8% 9% 10% 11% 12% 15% (n) Periods 1.00000 1.00000 1.00000 1.00000 1.00000 1.00000 2.08000 2.09000 2.10000 2.11000 2.12000 2.15000 2 3.24640 3.27810 3.31000 3.34210 3.37440 3.47250 4.99338 4.50611 4.57313 4.64100 4.70973 4.77933 5.86660 5.98471 6.10510 .22780 6.35285 6.74238 7.33592 7.52334 7.71561 7.91286 8.11519 8.75374 8.92280 9.20044 9.48717 9.78327 10.08901 11.06680 10.63663 11.02847 11.43589 11.85943 12.29969 13.72682 10 12.48756 13.02104 13.57948 14.48656 15.19293 15.93743 16.64549 17.56029 18.53117 18.97713 20.14072 21.38428 21.49530 22.95339 24.52271 24.21492 26.01919 27.97498 14.16397 16.72201 19.56143 22.71319 26.21164 30.09492 14.77566 17.54874 20.65458 24.13313 28.02911 32.39260 16.78584 20.30372 24.34928 29.00167 34.35192 40.50471 47.58041 55.71747 13 27.15211 29.36092 31.77248 34.40536 37.27972 30.32428 33.00340 35.94973 39.18995 42.75328 16 33.75023 36,97371 40,54470 44.50084 48.88367 65,07509 4. PRESENT VALUE OF AN ORDINARY ANNUITY OF 1 TABLE. Contains the amounts that must be deposited now at a specified rate of interest to permit withdrawals of 1 at the end of regular periodic intervals for the specified number of periods (Table 6.4). Table 6.4 PRESENT VALUE OF AN ORDINARY ANNUITY OF 1 1- PVF-OAnj = (1- (n) Periods 2% 24% 3% 4% 5% 6% 9803997561 97087 96154 95238 .94340 2 1.83339 2.67301 3.46511 1.94156 2.88388 3.80773 4.71346 5.60143 6.47199 1.92742 1.91347 1.88609 1.85941 2.85602 2.82861 2.77509 2.72325 3.76197 3.71710 3.62990 3.54595 4.64583 4.57971 4.45182 4.32948 5.508135.41719 5.24214 5.07569 6.34939 6.23028 6.00205 5.78637 4.21236 4.91732 5.58238 7.32548 7.17014 7.01969 6.73274 6.46321 6.20979 8,16224 797087 7.78611 7.43533 7.10782 6.80169 8.98259 8.75206 8.53020 8.11090 7.72173 7.36009 7.88687 9.78685 9.51421 9.25262 10.57534 10.25776 9.95400 8.76048 8.30641 9.38507 8.86325 8.38384 11.34837 10.98319 10.63496 9.98565 9.39357 8.85268 14 12.10625 11.69091 11.29607 10.56312 9.89864 9.29498 8% 9% 10% 11% 12% 15% (n) Periods 1.92593 91743 190909 90090 89286 86957 1.78326 1.75911 1.73554 1.71252 1.69005 1.62571 2.57710 2.53130 2.48685 2.44371 2.40183 2.28323 3.31213 3.23972 3.16986 3.10245 3.03735 2.85498 3.99271 3.88965 3.79079 3.69590 3.60478 3.35216 14.62288 4.48592 4.35526 4.23054 4.11141 3.78448 5.20637 5.03295 4.86842 4.71220 4.56376 4.16042 5.74664 5.53482 5.33493 5.14612 4.96764 4.48732 6.24689 5.99525 5.75902 5.53705 5.32825 4.77158 16.71008 6.41766 6.14457 5.88923 5.65022 5.01877 7.13896 6.80519 6.49506 6.20652 5.93770 5.23371 7.53608 7.16073 6.81369 6.49236 6.19437 5.42062 7.90378 7.48690 7.10336 6.74987 6.42355 5.58315 8.24424 7.78615 7.36669 6.98187 6.62817 5.72448 8.55948 8.06069 7.60608 7.19087 6.81086 5.84737 15 5. PRESENT VALUE OF AN ANNUITY DUE OF 1 TABLE. Contains the amounts that must be deposited now at a specified rate of interest to permit withdrawals of 1 at the beginning of regular periodic intervals for the specified number of periods (Table 6.5). Table 6.5 PRESENT VALUE OF AN ANNUITY DUE OF 1 PVF-AD 24% = 1+ 3% (n) Periods 2% 4% 5% 6% 1.00000 1.00000 1.00000 1.00000 1.00000 1.00000 1.98039 1.97561 1.97087 1.96154 1.95238 1.94340 2.83339 2.94156 2.92742 2.91347 2.88609 2.85941 3.88388 3.85602 3.82861 3.77509 3.72325 4.80773 4.76197 4.71710 4.62990 4.54595 3.67301 4.46511 5.71346 5.64583 5.57971 5.45182 5.32948 5.21236 6,60143 6.50813 6,41719 6.24214 6.07569 5.91732 6.58238 7.20979 7.80169 7.471997.349397 .23028 7.00205 6.78637 8.32548 8.17014 8.01969 7.73274 7.46321 9.16224 8.97087 8.78611 8.43533 8.10782 9.98259 9.75206 9.53020 9.11090 8.72173 10.78685 10.51421 10.25262 9.76048 9.30641 11.57534 11.25776 10.95400 10.38507 9.86325 12.34837 11.98319 11.63496 10.98565 10.39357 8.36009 8.88687 9.38384 14 9.85268 8% 9% 10% 11% 12% 15% (n) Periods 1.00000 1.00000 1.0000 1.00000 1.00000 1.0000 1.92593 1.91743 1.90909 1.90090 1.89286 1.86957 2 2.78326 2.75911 2.73554 2.71252 2.69005 2.62571 3.57710 3.53130 3.48685 3.44371 3.40183 3.28323 4.31213 4.23972 4.16986 4.10245 4.03735 3.85498 4.99271 4.88965 4.79079 4.69590 4.60478 4.35216 5.62288 5.48592 5.35526 5.23054 5.11141 4.78448 6.20637 6.03295 5.86842 5.71220 5.56376 5.16042 6.74664 6.53482 6.33493 6.14612 5.96764 | 5.48732 7.24689 6.99525 6.75902 6.53705 6.32825 5.77158 7.71008 7.41766 7.14457 6.88923 6.65022 6.01877 12 8.13896 7.80519 7.49506 7.20652 6.93770 6.23371 8.53608 8.16073 7.81369 7.49236 7.19437 6.42062 8.90378 8.48690 8.10336 7.74987 7.42355 6.58315 9.24424 8.78615 8.36669 7.98187 7.62817 6.72448 9.55948 9.06069 8.60608 8.19087 7.81086 6.84737 19.85137 9.31256 8.82371 8.37916 7.97399 6.95424 10.12164 9.54363 9.02155 8.54879 8.11963 7.04716 | 10.37189 9.75563 9.20141 8.70162 8.24967 7.12797 19 Sage Inc. manufactures an X-ray machine with an estimated life of 12 years and leases it to Pronghorn Medical Center for a period of 10 years. The normal selling price of the machine is $528, 109, and its guaranteed residual value at the end of the non-cancelable lease term is estimated to be $15,000. The hospital will pay rents of $64,000 at the beginning of each year. Sage incurred costs of $263,000 in manufacturing the machine and $14,200 in legal fees directly related to the signing of the lease. Sage has determined that the collectibility of the lease payments is probable and that the implicit interest rate is 5%. Pronghorn Medical Center has an incremental borrowing rate of 5% and an expected residual value at the end of the lease of $10,000. Click here to view factor tables (a2) x Your answer is incorrect. Try again. Compute the amount of the initial lease liability. (Round present value factor calculations to 5 decimal places, e.g. 1.25124 and the final answer to o decimal places e.g. 5,275.) Initial Lease Liability 503399 Click if you would like to Show Work for this question: Open Show Work 1. FUTURE VALUE OF 1 TABLE. Contains the amounts to which 1 will accumulate if deposited now at a specified rate and left for a specified number of periods (Table 6.1). Table 6.1 FUTURE VALUE OF 1 (FUTURE VALUE OF A SINGLE SUM) FVFnj = (1 + i)" (n) Periods 2% 2%2% 3% 4% 5% 6% 1.02000 1.02500 1.03000 1.04000 1.05000 1.06000 1.04040 1.05063 1.06090 1.08 160 1.10250 1.12360 1.06121 1.07689 1.09273 1.12486 1.15763 1.19102 1.21551 1.26248 1.27628 1.33823 1.08243 1.10408 1.12616 1.14869 1.17166 1.10381 1.13141 1.15969 1.18869 1.21840 1.12551 1.15927 1.19405 1.22987 1.26677 1.16986 1.21665 1.26532 1.31593 1.36857 1.41852 1.50363 1.40710 1.47746 1.59385 1.19509 1.24886 1.30477 1.42331 1.551331.68948 1.21899 1.28008 1.34392 1.48024 1.62889 1.79085 1.24337 1.31209 1.38423 1.53945 1.71034 1.89830 1.26824 1.34489 1.42576 1.60103 1.79586 2.01220 1.29361 1.37851 1.46853 1.66507 1.31948 1.41297 1.51259 1.73168 1.34587 1.44830 1.55797 1.80094 1.88565 2.13293 1.979932.26090 2.078932.39656 8% 9% 10% 11% 12% 15% (n) Periods 1.08000 1.09000 1.10000 1.11000 1.12000 1.15000 1 1.16640 1.18810 1.21000 1.23210 1.25440 1.32250 1.25971 1.29503 1.33100 1.36763 1.40493 1.52088 1.36049 1.41158 1.46410 1.51807 1.57352 1.74901 1.46933 1.53862 1.61051 1.68506 1.76234 2.01136 1.58687 1.67710 1.77156 1.87041 1.97382 1.71382 1.82804 1.94872 2.07616 2.21068 1.85093 1.99256 2.14359 2.30454 2.47596 2.31306 2.66002 3.05902 1.99900 2.17189 2.35795 2.55803 2.77308 3.51788 2.15892 2.36736 2.59374 2.83942 3.10585 4.04556 2.33164 2.58043 2.85312 3.15176 3.47855 4.65239 2.51817 2.71962 2.81267 3.06581 3.13843 3.49845 3.89598 3.45227 3.88328 4.36349 5.35025 6.15279 2.93719 3.34173 3.79750 4.31044 4.88711 3.17217 3.64248 4.17725 4.78459 5.47357 7.07571 8.13706 15 2. PRESENT VALUE OF 1 TABLE. Contains the amounts that must be deposited now at a specified rate of interest to equal 1 at the end of a specified number of periods (Table 6.2). Table 6.2 PRESENT VALUE OF 1 (PRESENT VALUE OF A SINGLE SUM) PVFn,i = tx = (1+i)" (n) Periods 2% 22% 3% 4% 5% 6% .94340 .98039 97561 97087 96154 95238 .96117 95181 94260 92456 90703 .89000 94232 92860 91514 88900 86384 ,83962 .79209 92385 90595 88849 .85480 82270 .90573 .88385 .86261 82193 78353 .88797 86230 83748 79031 74622 .74726 .70496 87056 .84127 81309 75992 71068 .66506 .62741 .59190 .55839 .85349 82075 78941 73069 67684 .83676 80073 76642 70259 .64461 .82035 78120 74409 67556 61391 .80426 76214 72242 64958 58468 .78849 .74356 70138 .62460 55684 .77303 72542 .68095 .60057 .53032 .75788 70773 66112 57748 50507 .52679 ,49697 46884 44230 | 8% 9% 10% 11% 12% 15% (n) Periods 1.92593 91743.90909 ,90090 .89286 86957 85734.84168 .82645 81162.79719.756142 1.79383.77218.75132 73119 71178.65752 3 173503.70843.68301 .65873.63552 .57175 .68058 .64993.62092 .59345 .56743 .49718 163017 .59627.56447 .53464 .50663 43233 158349 .54703 .51316 48166 45235 37594 154027.50187 46651 .43393 40388 .32690 150025 46043 42410 .39092 36061 .28426 146319 .42241 38554 .35218 .32197.24719 142888 .38753.35049 31728 28748 21494 139711.35554 .31863 .28584 .25668.18691 136770 .32618 28966 .25751.22917.16253 134046 .29925 26333 .23199 .20462 .14133 1.31524 27454 23939.20900 .18270 .12289 15 3. FUTURE VALUE OF AN ORDINARY ANNUITY OF 1 TABLE. Contains the amounts to which periodic rents of 1 will accumulate if the payments (rents) are invested at the end of each period at a specified rate of interest for a specified number of periods (Table 6.3). Table 6.3 FUTURE VALUE OF AN ORDINARY ANNUITY OF 1 FVF-OAni = "+"= (x) Periods 2% 24% 3% 4% 5% 1.00000 1.00000 100000 1.00000 1.00000 2.02000 2.02500 2.03000 2.04000 2.05000 6% 1.00000 2.06000 3.06040 3.07563 3.09090 3.12160 3.15250 3.18360 4.12161 4.15252 4.18363 4.31013 4.37462 5.20404 5.25633 5.30914 5.41632 5.52563 5.63709 6.30812 7.43428 6.38774 7.54743 6.46841 7.66246 6.63298 7.89829 6.80191 8.14201 6.97532 8.39384 8.89234 9.21423 9.54911 9.89747 8.58297 9.75463 8.73612 9.95452 10.15911 10.58280 11.02656 12.57789 11.49132 13.18079 10.94972 11.20338 11.46338 12.00611 12.16872 12.48347 12.80780 13.48635 14.20679 14.97164 13.41209 13.79555 14.19203 15.02581 15.91713 16.86994 13 14.68033 15.14044 15.61779 16.62684 17.71298 18.88214 8% 9% 10% 11% 12% 15% (n) Periods 1.00000 1.00000 1.00000 1.00000 1.00000 1.00000 2.08000 2.09000 2.10000 2.11000 2.12000 2.15000 2 3.24640 3.27810 3.31000 3.34210 3.37440 3.47250 4.99338 4.50611 4.57313 4.64100 4.70973 4.77933 5.86660 5.98471 6.10510 .22780 6.35285 6.74238 7.33592 7.52334 7.71561 7.91286 8.11519 8.75374 8.92280 9.20044 9.48717 9.78327 10.08901 11.06680 10.63663 11.02847 11.43589 11.85943 12.29969 13.72682 10 12.48756 13.02104 13.57948 14.48656 15.19293 15.93743 16.64549 17.56029 18.53117 18.97713 20.14072 21.38428 21.49530 22.95339 24.52271 24.21492 26.01919 27.97498 14.16397 16.72201 19.56143 22.71319 26.21164 30.09492 14.77566 17.54874 20.65458 24.13313 28.02911 32.39260 16.78584 20.30372 24.34928 29.00167 34.35192 40.50471 47.58041 55.71747 13 27.15211 29.36092 31.77248 34.40536 37.27972 30.32428 33.00340 35.94973 39.18995 42.75328 16 33.75023 36,97371 40,54470 44.50084 48.88367 65,07509 4. PRESENT VALUE OF AN ORDINARY ANNUITY OF 1 TABLE. Contains the amounts that must be deposited now at a specified rate of interest to permit withdrawals of 1 at the end of regular periodic intervals for the specified number of periods (Table 6.4). Table 6.4 PRESENT VALUE OF AN ORDINARY ANNUITY OF 1 1- PVF-OAnj = (1- (n) Periods 2% 24% 3% 4% 5% 6% 9803997561 97087 96154 95238 .94340 2 1.83339 2.67301 3.46511 1.94156 2.88388 3.80773 4.71346 5.60143 6.47199 1.92742 1.91347 1.88609 1.85941 2.85602 2.82861 2.77509 2.72325 3.76197 3.71710 3.62990 3.54595 4.64583 4.57971 4.45182 4.32948 5.508135.41719 5.24214 5.07569 6.34939 6.23028 6.00205 5.78637 4.21236 4.91732 5.58238 7.32548 7.17014 7.01969 6.73274 6.46321 6.20979 8,16224 797087 7.78611 7.43533 7.10782 6.80169 8.98259 8.75206 8.53020 8.11090 7.72173 7.36009 7.88687 9.78685 9.51421 9.25262 10.57534 10.25776 9.95400 8.76048 8.30641 9.38507 8.86325 8.38384 11.34837 10.98319 10.63496 9.98565 9.39357 8.85268 14 12.10625 11.69091 11.29607 10.56312 9.89864 9.29498 8% 9% 10% 11% 12% 15% (n) Periods 1.92593 91743 190909 90090 89286 86957 1.78326 1.75911 1.73554 1.71252 1.69005 1.62571 2.57710 2.53130 2.48685 2.44371 2.40183 2.28323 3.31213 3.23972 3.16986 3.10245 3.03735 2.85498 3.99271 3.88965 3.79079 3.69590 3.60478 3.35216 14.62288 4.48592 4.35526 4.23054 4.11141 3.78448 5.20637 5.03295 4.86842 4.71220 4.56376 4.16042 5.74664 5.53482 5.33493 5.14612 4.96764 4.48732 6.24689 5.99525 5.75902 5.53705 5.32825 4.77158 16.71008 6.41766 6.14457 5.88923 5.65022 5.01877 7.13896 6.80519 6.49506 6.20652 5.93770 5.23371 7.53608 7.16073 6.81369 6.49236 6.19437 5.42062 7.90378 7.48690 7.10336 6.74987 6.42355 5.58315 8.24424 7.78615 7.36669 6.98187 6.62817 5.72448 8.55948 8.06069 7.60608 7.19087 6.81086 5.84737 15 5. PRESENT VALUE OF AN ANNUITY DUE OF 1 TABLE. Contains the amounts that must be deposited now at a specified rate of interest to permit withdrawals of 1 at the beginning of regular periodic intervals for the specified number of periods (Table 6.5). Table 6.5 PRESENT VALUE OF AN ANNUITY DUE OF 1 PVF-AD 24% = 1+ 3% (n) Periods 2% 4% 5% 6% 1.00000 1.00000 1.00000 1.00000 1.00000 1.00000 1.98039 1.97561 1.97087 1.96154 1.95238 1.94340 2.83339 2.94156 2.92742 2.91347 2.88609 2.85941 3.88388 3.85602 3.82861 3.77509 3.72325 4.80773 4.76197 4.71710 4.62990 4.54595 3.67301 4.46511 5.71346 5.64583 5.57971 5.45182 5.32948 5.21236 6,60143 6.50813 6,41719 6.24214 6.07569 5.91732 6.58238 7.20979 7.80169 7.471997.349397 .23028 7.00205 6.78637 8.32548 8.17014 8.01969 7.73274 7.46321 9.16224 8.97087 8.78611 8.43533 8.10782 9.98259 9.75206 9.53020 9.11090 8.72173 10.78685 10.51421 10.25262 9.76048 9.30641 11.57534 11.25776 10.95400 10.38507 9.86325 12.34837 11.98319 11.63496 10.98565 10.39357 8.36009 8.88687 9.38384 14 9.85268 8% 9% 10% 11% 12% 15% (n) Periods 1.00000 1.00000 1.0000 1.00000 1.00000 1.0000 1.92593 1.91743 1.90909 1.90090 1.89286 1.86957 2 2.78326 2.75911 2.73554 2.71252 2.69005 2.62571 3.57710 3.53130 3.48685 3.44371 3.40183 3.28323 4.31213 4.23972 4.16986 4.10245 4.03735 3.85498 4.99271 4.88965 4.79079 4.69590 4.60478 4.35216 5.62288 5.48592 5.35526 5.23054 5.11141 4.78448 6.20637 6.03295 5.86842 5.71220 5.56376 5.16042 6.74664 6.53482 6.33493 6.14612 5.96764 | 5.48732 7.24689 6.99525 6.75902 6.53705 6.32825 5.77158 7.71008 7.41766 7.14457 6.88923 6.65022 6.01877 12 8.13896 7.80519 7.49506 7.20652 6.93770 6.23371 8.53608 8.16073 7.81369 7.49236 7.19437 6.42062 8.90378 8.48690 8.10336 7.74987 7.42355 6.58315 9.24424 8.78615 8.36669 7.98187 7.62817 6.72448 9.55948 9.06069 8.60608 8.19087 7.81086 6.84737 19.85137 9.31256 8.82371 8.37916 7.97399 6.95424 10.12164 9.54363 9.02155 8.54879 8.11963 7.04716 | 10.37189 9.75563 9.20141 8.70162 8.24967 7.12797 19