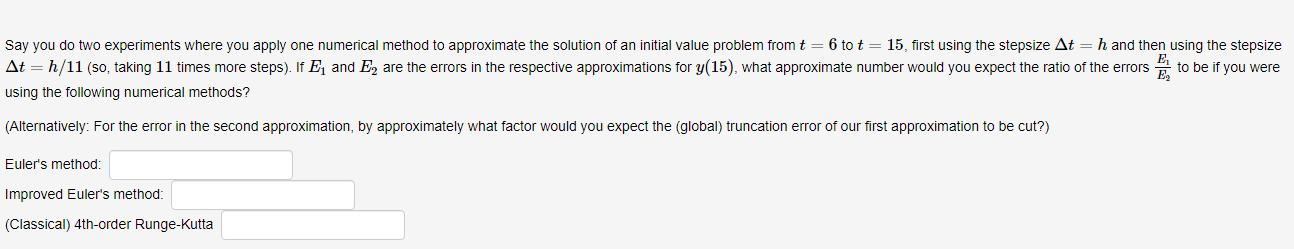
Say you do two experiments where you apply one numerical method to approximate the solution of an initial value problem fromt = 6 to t = 15, first using the stepsize At =h and then using the stepsize At = h/11 (so, taking 11 times more steps). If E, and E, are the errors in the respective approximations for y(15), what approximate number would you expect the ratio of the errors E, to be if you were using the following numerical methods? (Alternatively: For the error in the second approximation, by approximately what factor would you expect the (global) truncation error of our first approximation to be cut?) Euler's method: Improved Euler's method: (Classical) 4th-order Runge-Kutta