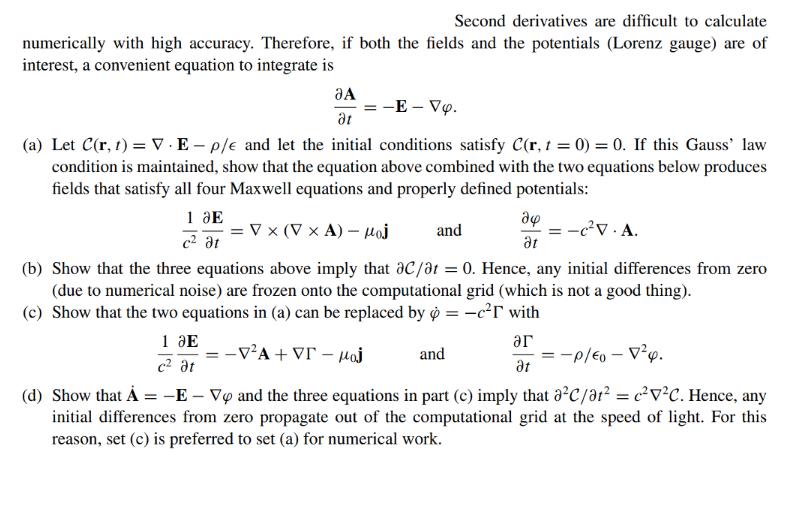
Second derivatives are difficult to calculate numerically with high accuracy. Therefore, if both the fields and the potentials (Lorenz gauge) are of interest, a convenient equation to integrate is -E V. r (a) Let C(r, t) = V.E - p/e and let the initial conditions satisfy C(r, 1 = 0) = 0. If this Gauss' law condition is maintained, show that the equation above combined with the two equations below produces fields that satisfy all four Maxwell equations and properly defined potentials: 1 JE = V x (V x A)- Hoj c2 at -c?v. A. and r (b) Show that the three equations above imply that aC/at = 0. Hence, any initial differences from zero (due to numerical noise) are frozen onto the computational grid (which is not a good thing). (c) Show that the two equations in (a) can be replaced by = -cr with 1 JE -vA + Vr Haj = -p/e0 V?o. and c2 at r (d) Show that = -E - Vo and the three equations in part (c) imply that a C/ar? = cvC. Hence, any initial differences from zero propagate out of the computational grid at the speed of light. For this reason, set (c) is preferred to set (a) for numerical work.