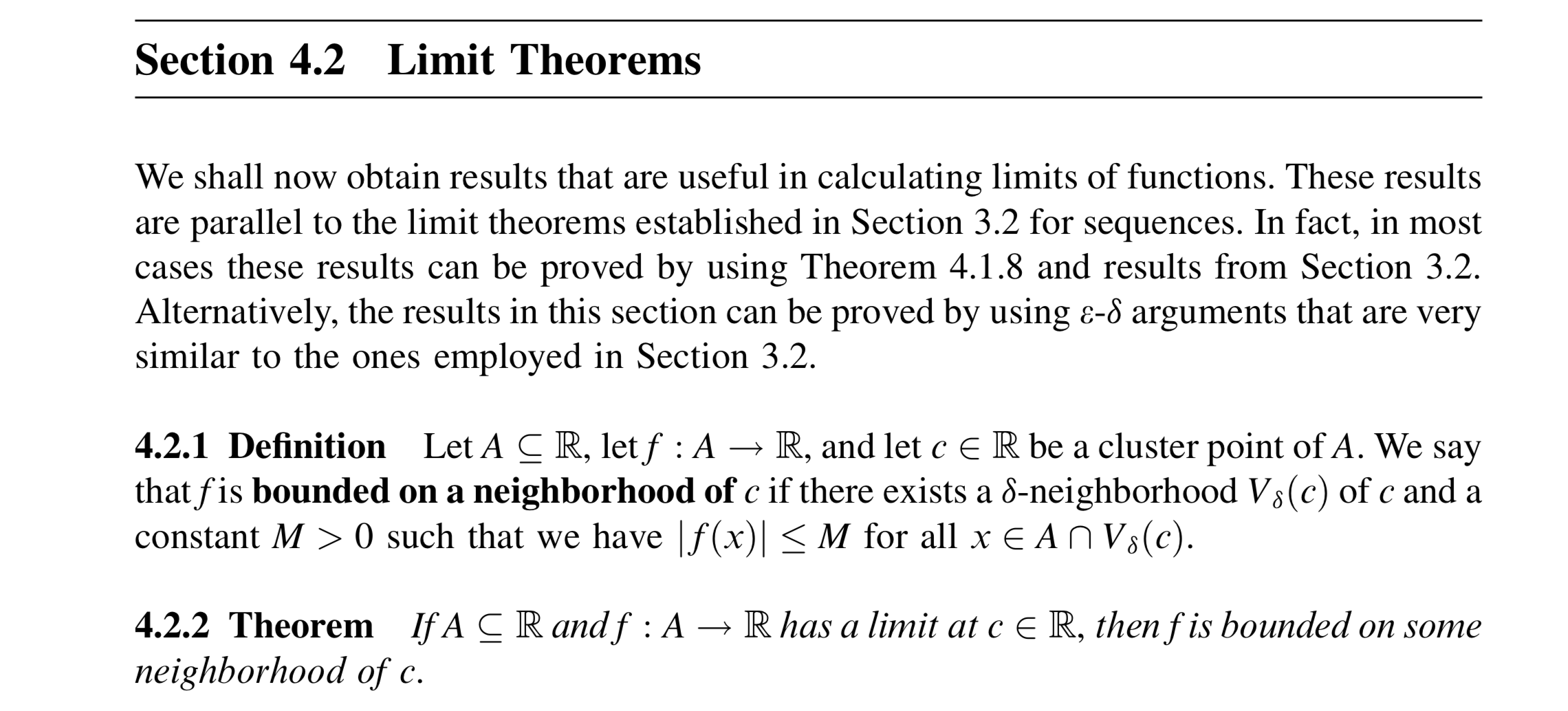
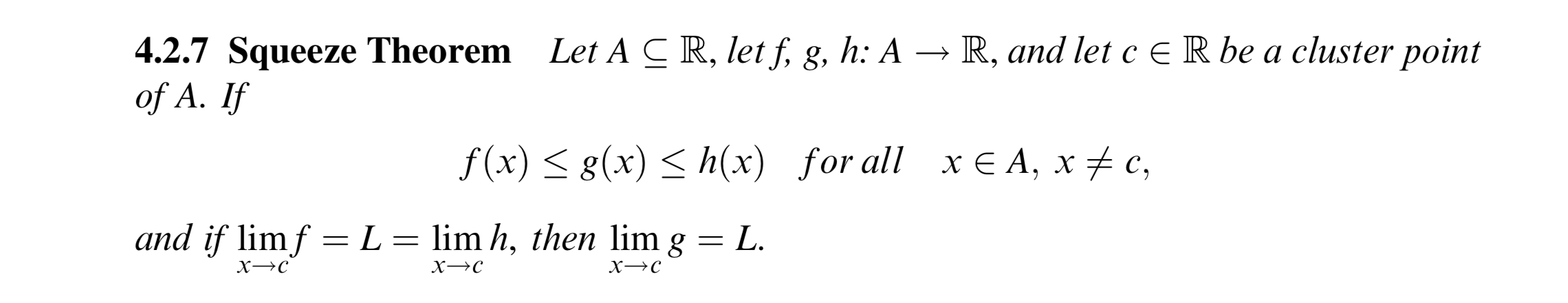
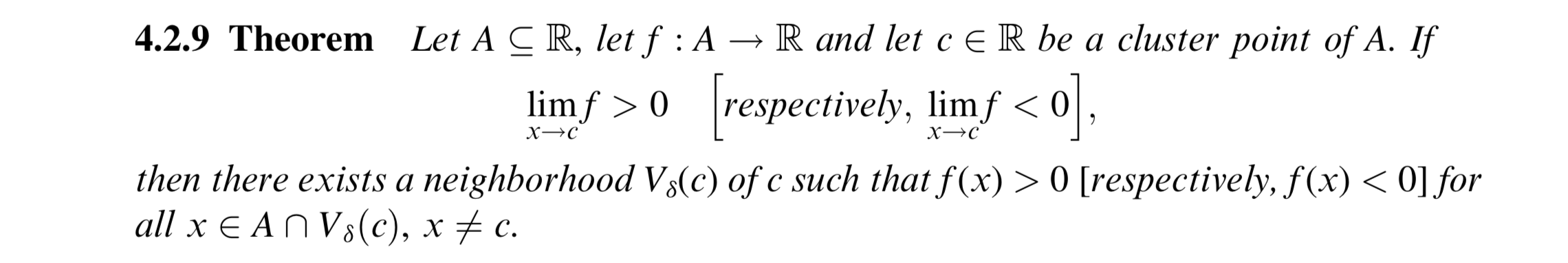
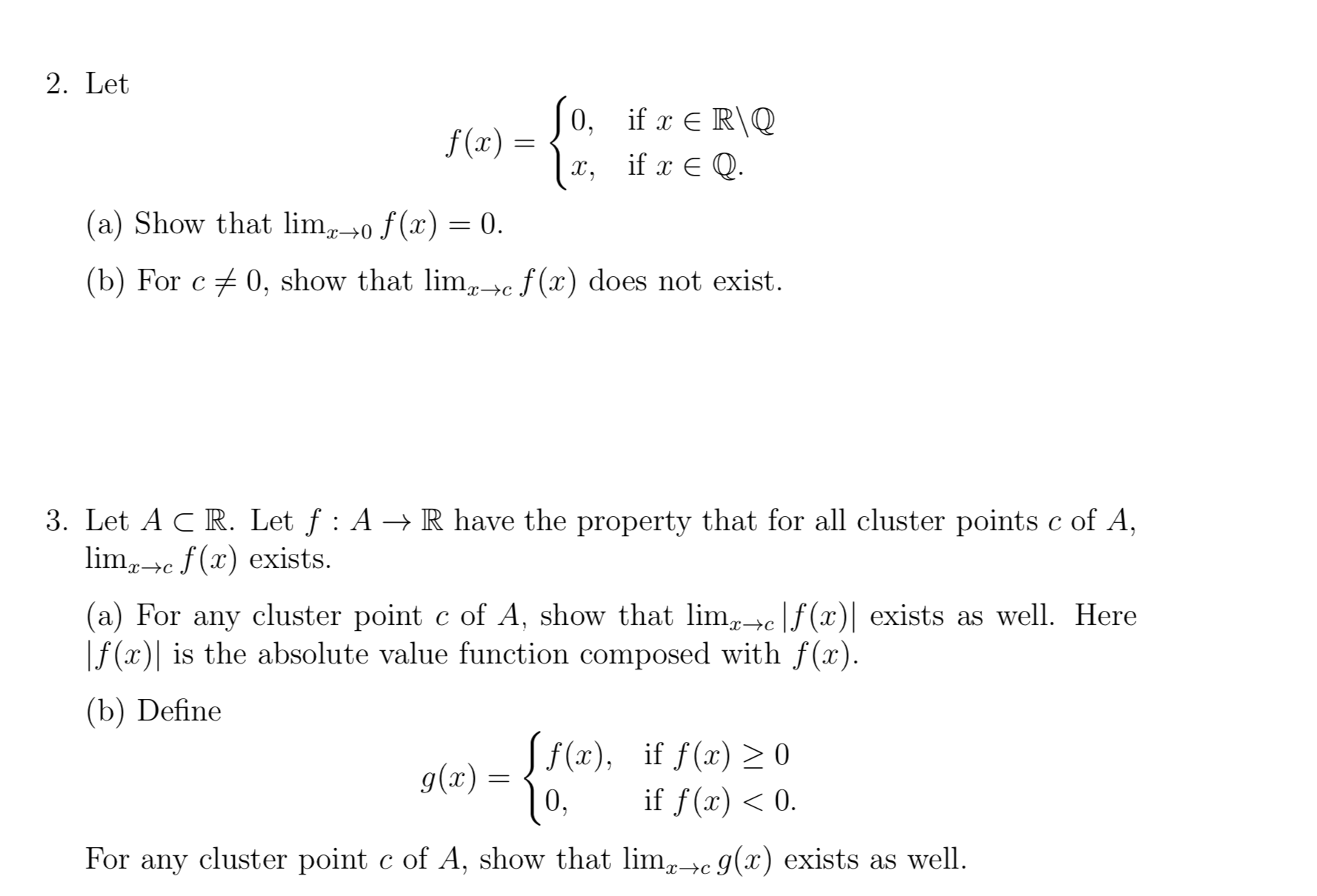
Section 4.2 Limit Theorems We shall now obtain results that are useful in calculating limits of functions. These results are parallel to the limit theorems established in Section 3.2 for sequences. In fact, in most cases these results can be proved by using Theorem 4.1.8 and results from Section 3.2. Alternatively, the results in this section can be proved by using 8-8 arguments that are very similar to the ones employed in Section 3.2. 4.2.1 Definition Let A CR, let f: A > R, and let c ER be a cluster point of A. We say that fis bounded on a neighborhood of c if there exists a 8-neighborhood Vs (c) of c and a constant M > 0 such that we have |f(x)| M for all x A V8(c). 4.2.2 Theorem If ACR and f : A neighborhood of c. R has a limit at c = R, then f is bounded on some 4.2.7 Squeeze Theorem Let A C R, let f, g, h: AR, and let c ER be a cluster point of A. If f(x) g(x) h(x) for all x A, x c, and if limf = L = lim h, then lim g = L. - X C X C 4.2.9 Theorem Let A CR, let f : A R and let c ER be a cluster point of A. If lim>0 [respectively, lim 0 [respectively, f(x) < 0] for all x = An Vs(c), xc. 2. Let f(x) = (a) Show that limo f(x) = 0. = = 0, x, if x R\Q X, if x = Q. (b) For c 0, show that lime f(x) does not exist. 3. Let A C R. Let : A R have the property that for all cluster points c of A, limxc f(x) exists. (a) For any cluster point c of A, show that lim c|f(x)| exists as well. Here |f(x) is the absolute value function composed with f(x). (b) Define f(x), if f(x) 0 g(x) = 10, if f(x) < 0. For any cluster point c of A, show that lim g(x) exists as well.