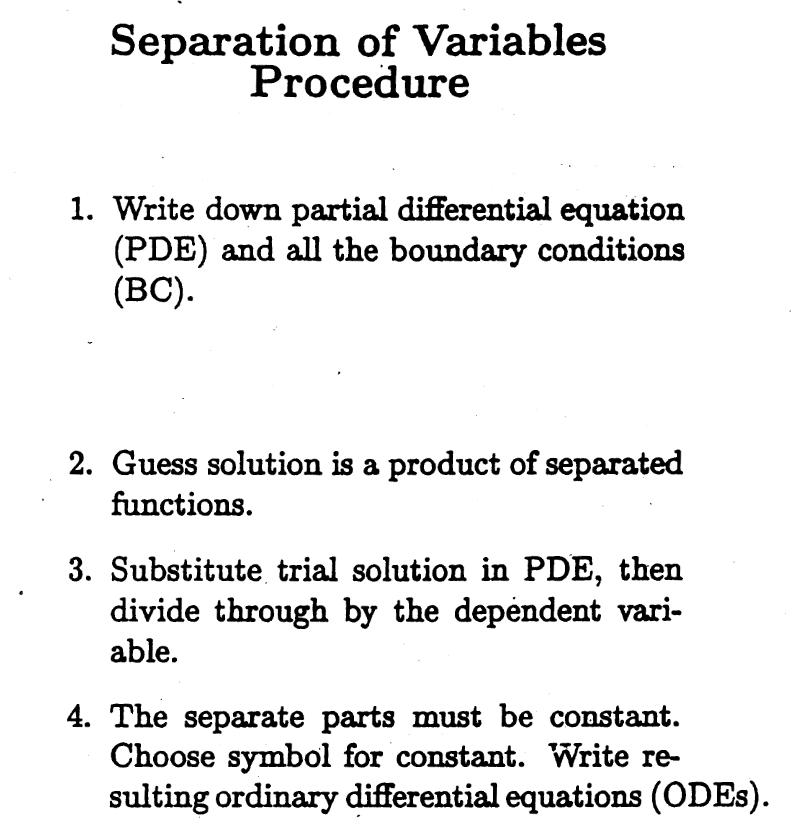
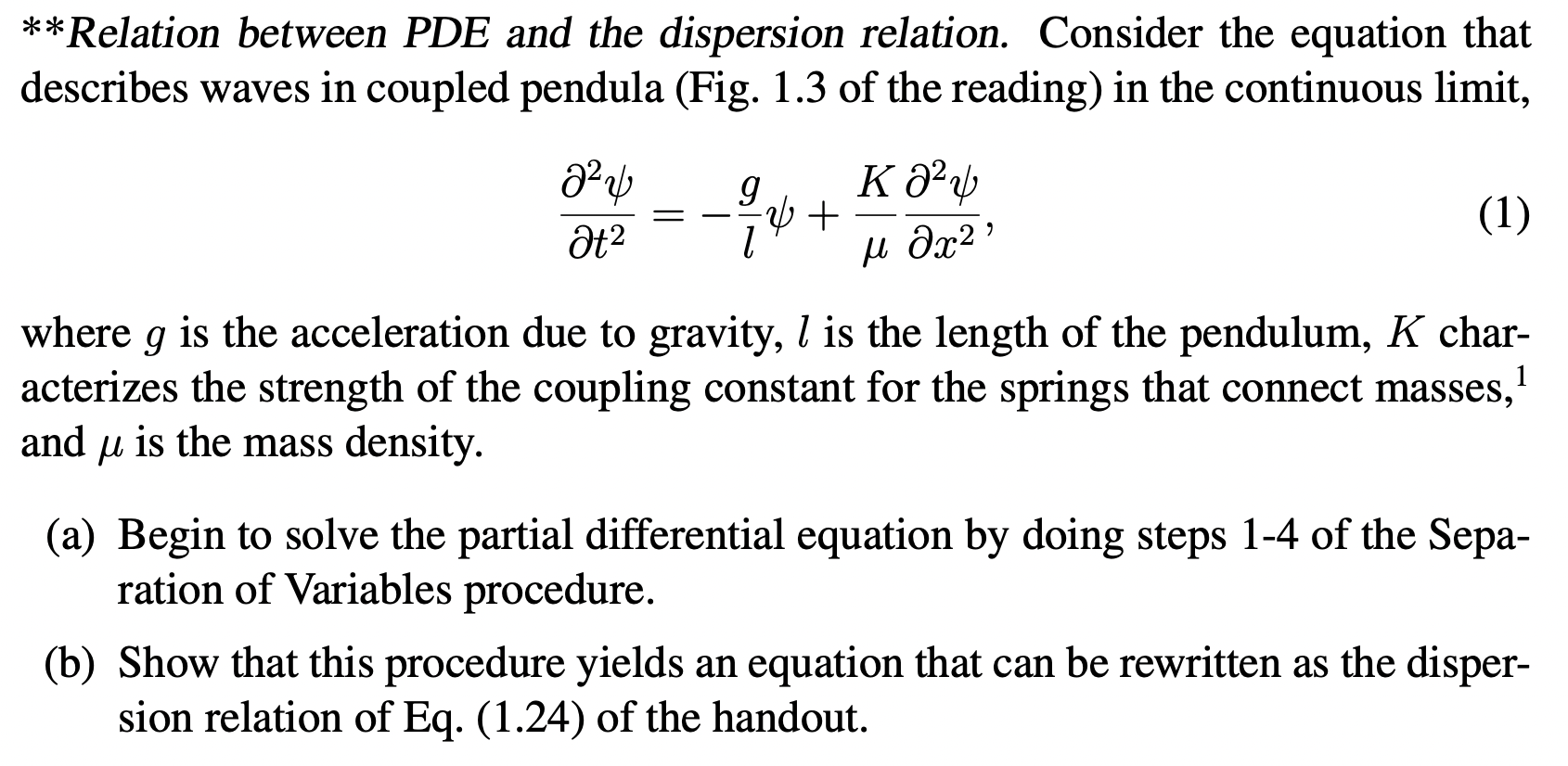
Separation of Variables Procedure 1. Write down partial differential equation (PDE) and all the boundary conditions (BC). 2. Guess solution is a product of separated functions. 3. Substitute trial solution in PDE, then divide through by the dependent vari- able. 4. The separate parts must be constant. Choose symbol for constant. Write re- sulting ordinary differential equations (ODEs). ** *Relation between PDE and the dispersion relation. Consider the equation that describes waves in coupled pendula (Fig. 1.3 of the reading) in the continuous limit, t K g 4 + 2 " (1) where g is the acceleration due to gravity, I is the length of the pendulum, K char- acterizes the strength of the coupling constant for the springs that connect masses, and u is the mass density. (a) Begin to solve the partial differential equation by doing steps 1-4 of the Sepa- ration of Variables procedure. (b) Show that this procedure yields an equation that can be rewritten as the disper- sion relation of Eq. (1.24) of the handout.