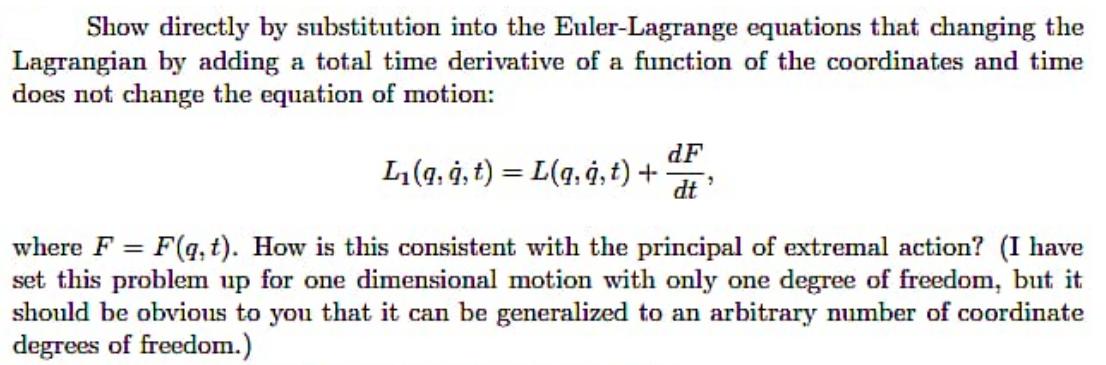
Show directly by substitution into the Euler-Lagrange equations that changing the Lagrangian by adding a total time derivative of a function of the coordinates and time does not change the equation of motion: L1(q, q,t) = L(q, q, t) + dF/dt where F = F(q, t). How is this consistent with the principal of extremal action? (I have set this problem up for one dimensional motion with only one degree of freedom, but it should be obvious to you that it can be generalized to an arbitrary number of coordinate degrees of freedom.)