show how the answer below looks in Matlab.

this is from chapter 3 of this book

answer from answer key please show what it looks like in matlab

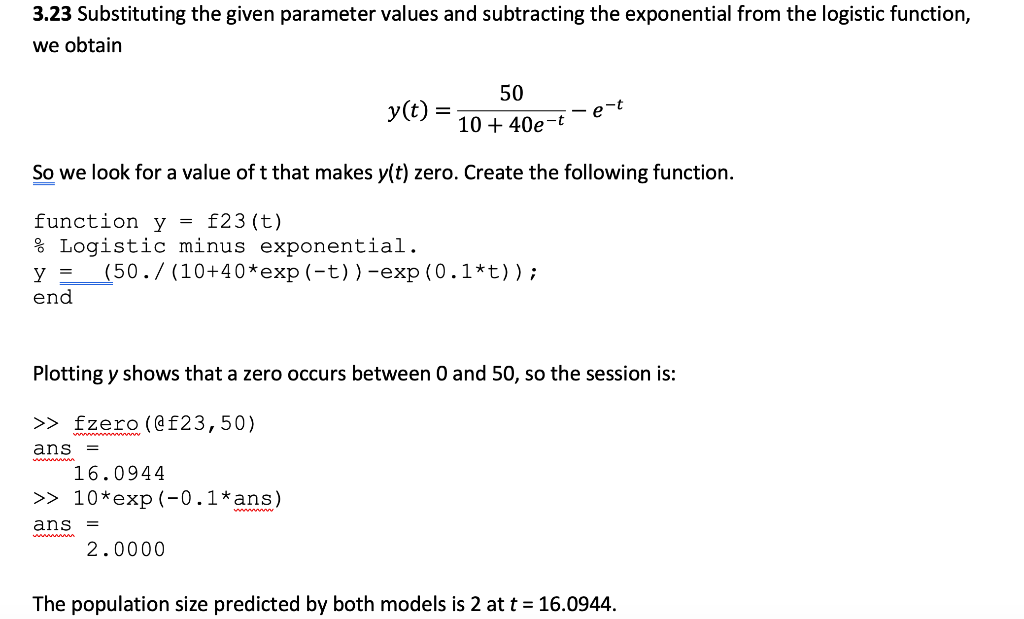
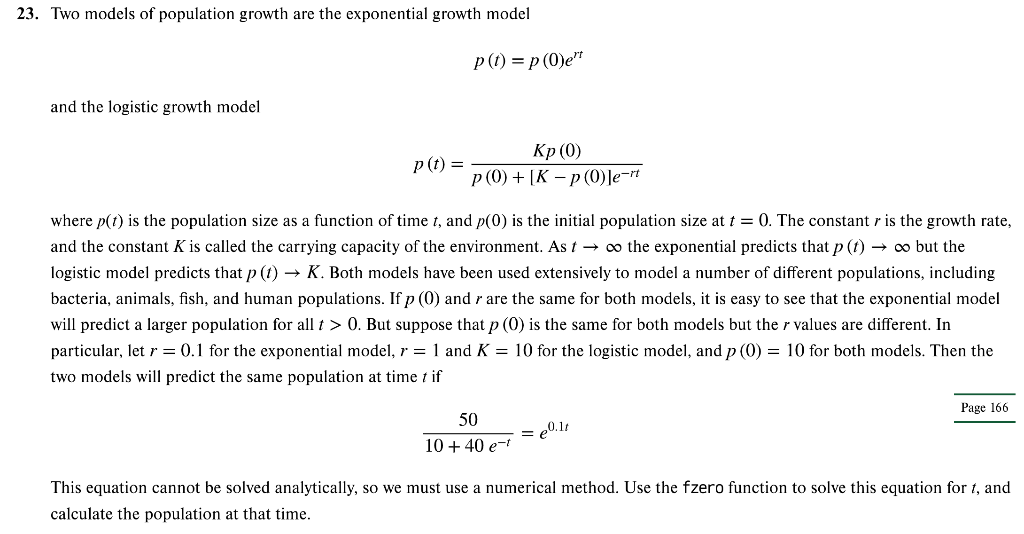
23. Two models of population growth are the exponential growth model p(t)=p(0)ert and the logistic growth model p(t)=p(0)+[Kp(0)]ertKp(0) where p(t) is the population size as a function of time t, and p(0) is the initial population size at t=0. The constant r is the growth rate, and the constant K is called the carrying capacity of the environment. As t the exponential predicts that p(t) but the logistic model predicts that p(t)K. Both models have been used extensively to model a number of different populations, including bacteria, animals, fish, and human populations. If p(0) and r are the same for both models, it is easy to see that the exponential model will predict a larger population for all t>0. But suppose that p(0) is the same for both models but the r values are different. In particular, let r=0.1 for the exponential model, r=1 and K=10 for the logistic model, and p(0)=10 for both models. Then the two models will predict the same population at time t if 10+40et50=e0.1t This equation cannot be solved analytically, so we must use a numerical method. Use the fzero function to solve this equation for t, and calculate the population at that time. Product: MATLAB for Engineering Applications Edition: 5th Author: William Palm ISBN10: 1264926804 3.23 Substituting the given parameter values and subtracting the exponential from the logistic function, we obtain y(t)=10+40et50et So we look for a value of t that makes y(t) zero. Create the following function. function y=f23(t) \% Logistic minus exponential. y=(50./(10+40exp(t))exp(0.1t)); end Plotting y shows that a zero occurs between 0 and 50 , so the session is: > fzero (@f23,50) ans = 16.0944 >10exp(0.1 ans ) ans = 2.0000 The population size predicted by both models is 2 at t=16.0944