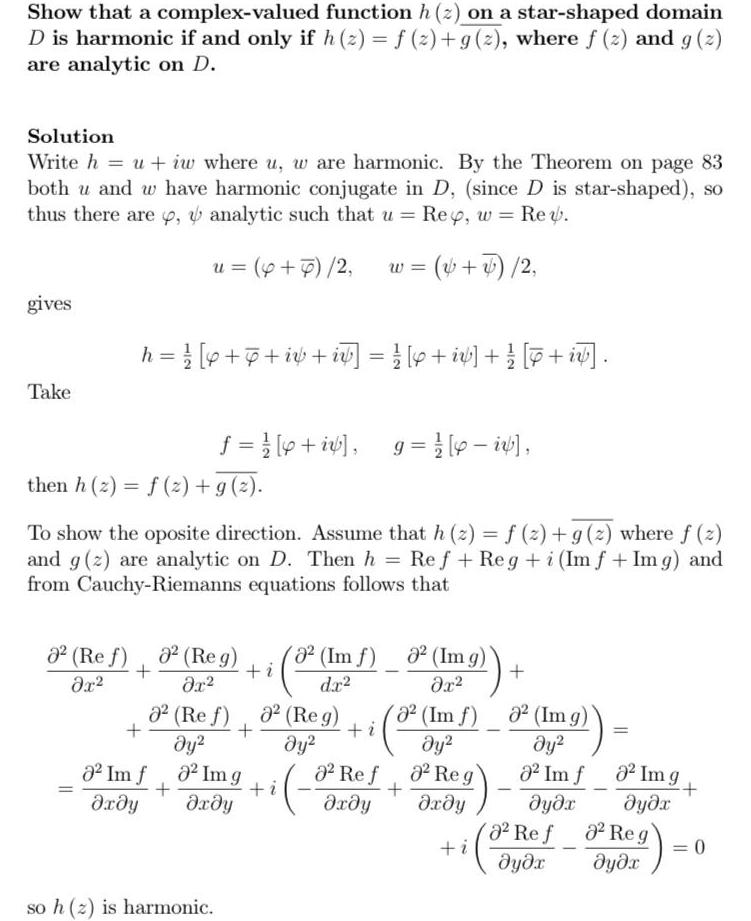
Show that a complex valued function h 2 on a star shaped domain D is harmonic if and only if h 2 f 2 g 2 where 2 and g 2 are analytic on D Solution Write h u iw where u w are harmonic By the Theorem on page 83 both u and w have harmonic conjugate in D since D is star shaped so thus there are analytic such that u Rey w Rev u y p 2 w v v 2 gives Take h p p iv iv 9 iv p ip then h 2 f z g z 8 Ref x To show the oposite direction Assume that h z f z g 2 where f 2 and g z are analytic on D Then h Ref Reg i Im f Im g and from Cauchy Riemanns equations follows that f 6 i 9 p iv 0 Im f x y Reg x 0 Ref dy so h 2 is harmonic Img x y i Im f Im g x dx 8 Reg dy i i 2 8 Im f dy 02 Reg x y Ref x y i 3 Im g dy 2 Im f y x 8 Ref y x Im g y x Reg y x 0